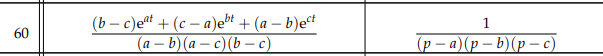How to calculate inverse Laplace of this response function?

Question

Sorry for my English in terms of control theory - up to now I used only German phrases in that field.

There is a controlled process of order 2 with

$$F_p(s)=\frac{1}{(1+s)^2} $$

which shall be controlled in "standard-form" by the controller of type I

$$F_c(s)=\frac{1}{sT} $$

The total transfer function from setpoint to output is $$F(s)=\frac{F_p F_c}{1+F_p F_c} = \frac{1}{sT(1+s)^2+1}$$

How do I get the time-function at the output, when a step-function is applied at the input?

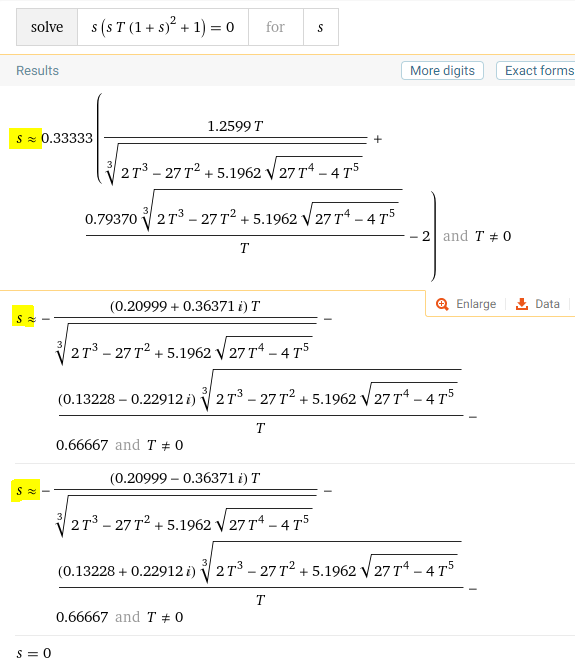
I cannot manage to get the complex roots of the denominator:

$$sT(1+s)^2+1 = K(s-s_1)(s-s_2)(s-s_3)$$

The original question was:

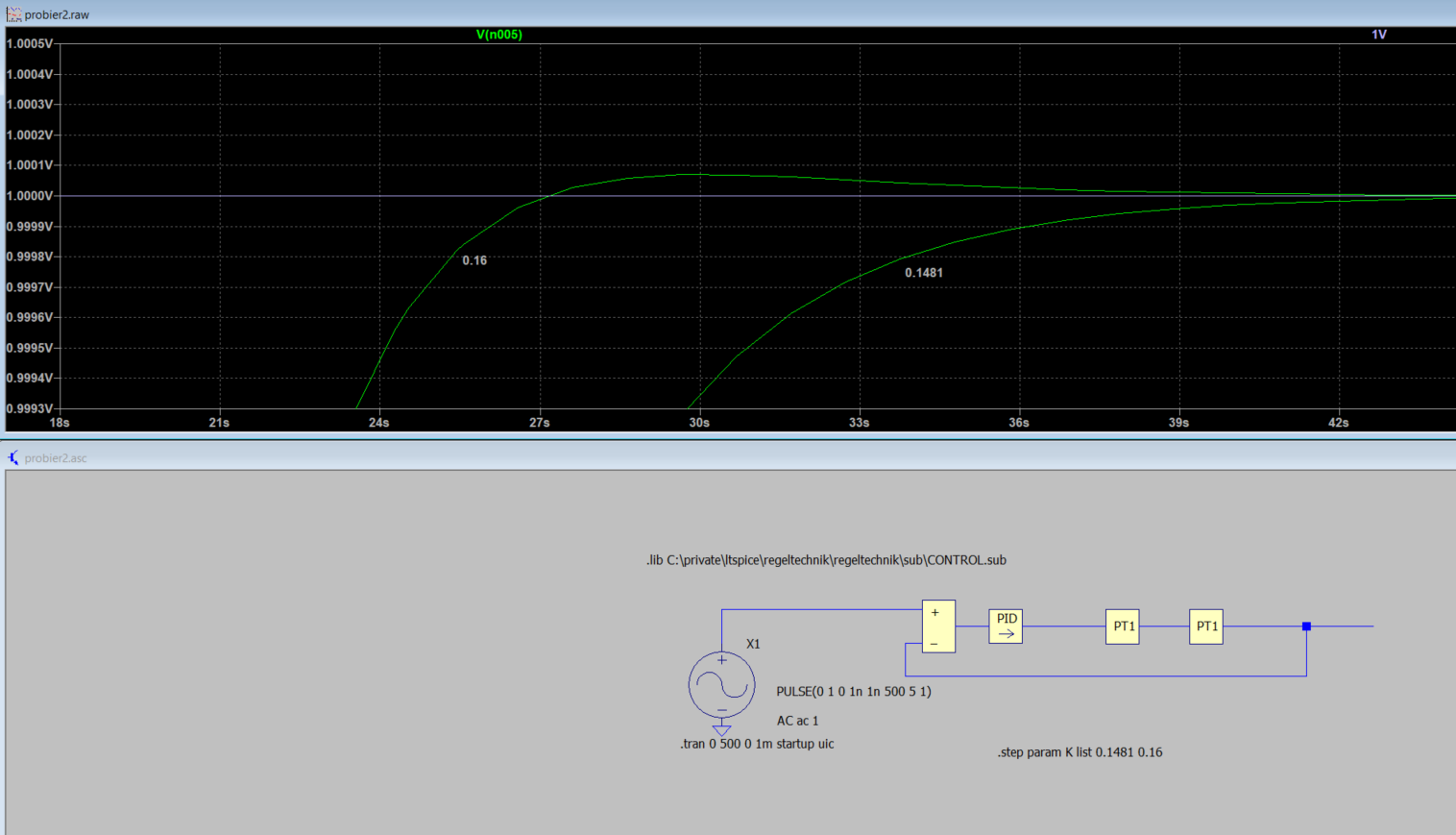
How to choose T, so that the system is right on the edge of a swing-over (aperiodic limiting case) behavior.

I have no Idea, how to solve this analytically.

By using Wolfram alpha

I found roots, but there are two are complex conjugated roots and one real one. The analytic representation is quite awful, but, anyway, there are complex solutions. Each complex solution gives rise to a damped oscillation, so how can it be, that T can be chosen at all to yield no overswing (see image of inverse Laplace)? BTW: By simulation of the closed loop I'm convinced, that there IS a limit for T to yield aperiodic behavior.

Answer 1

To answer my own question: I think the solution is as follows (it took me incredible long to find it out, although the problem seems quite simple):

Assuming to be at the edge of just having no complex solution, there must be one double root and one single root:

So the ansatz is:

$$Ts(1+s)^2+1 = T(1+s_1)^2(1+s_2)$$

This gives by coefficient comparison

$$s_1=1/3, s_2=4/3, T=27/4$$

So the transfer function for just having no overswing is

$$F(s) = \frac{1}{\frac{27}{4}s(1+s)^2+1}$$

and the I-control is

$$F_c(s) = \frac{4}{27}\cdot \frac{1}{s}$$

The simulation is excatly as expected:

The parameter is

$$K_I = 1/T$$

showing the behavior at the boundary. The limit for T is 27/4, a slightly smaller value gives rise to overswing.