I'm trying to follow along with this blog post reverse engineering the HC-SR04 ultrasonic distance sensor.
The signal from the receiving transducer is amplified, filtered, and then amplified again.
On the filter, there is a 1 nF cap C4 between the signal and inverting input which I believe is a DC block. There is a second 1 nF cap C3 between the signal and op amp output.
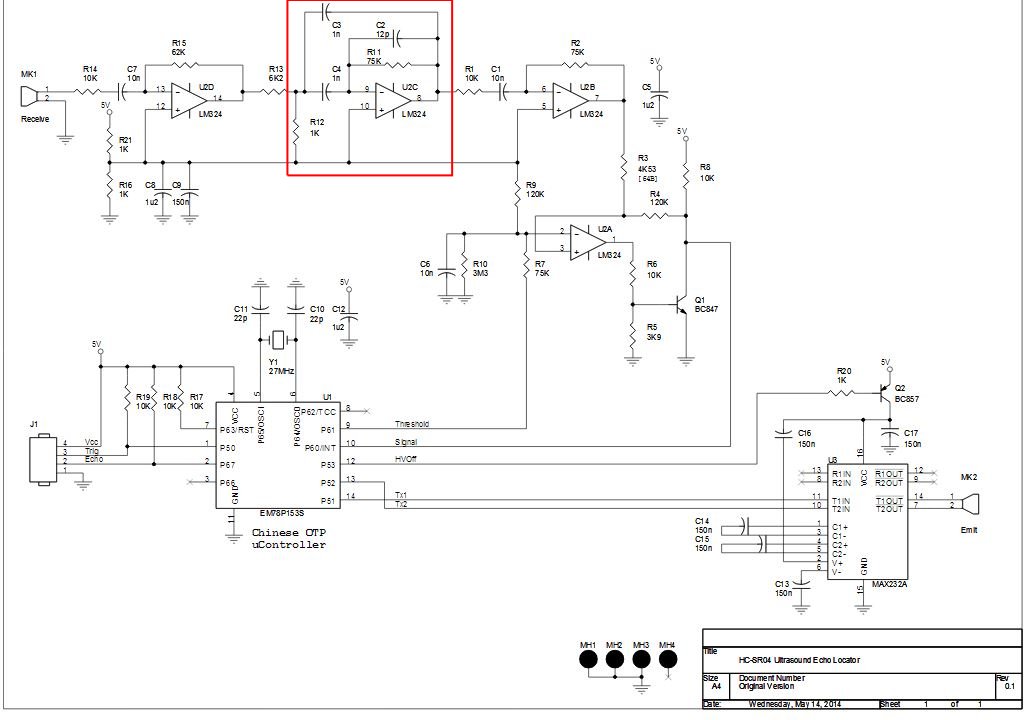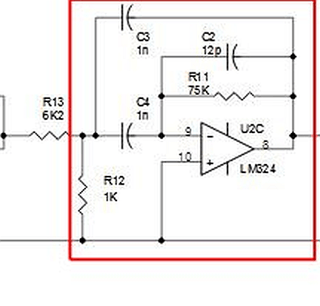
What is the purpose of C3?
The expected signal frequency is 40 kHz.
(corrected) Pardon my poor engineeringlish.
I meant Negative Inductance not -L. L is always a positive interger, with positive reactance, while C is a negative reactance, unless used in an impedance converter with feedback.
(so always think in terms of impedance)
C4 is an active inductor or negative capacitor as the negative impedance converter (NIC) while C3 is the active capacitor or negative inductor using negative feedback as an integrator by subtracting its response from the input 820 ohm source impedance. (1k // 6k2) While C2 has very little effect and C4 in series with C3 are equivalent to a passive L//C to virtual AC grounds. (Vin- and Vout) The NIC converts the series C3:C4 notch filter into a BPF using NIC negative feedback via Vin-. (I hope this is clear)
C2 is a weak integrator pole 2 orders of magnitude in f above the BPF, just before the possible GBW limits to normalize the filter variance with IC's having tolerances on a 5~10 MHz GBW or so as to reduce the peak gain variance yet drop it by a fixed amount 3dB. It could improve phase margin on IC's with << 60 deg. that is my hunch.
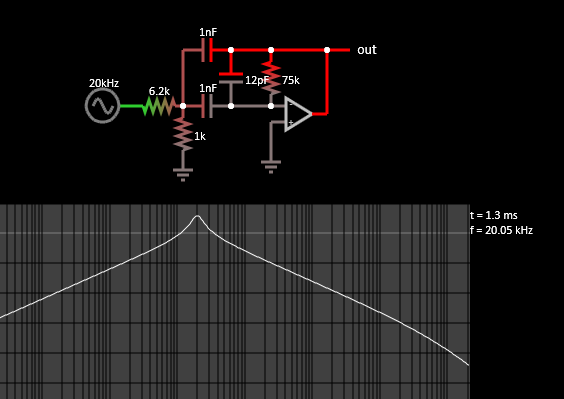
The actual frequency is about 20 kHz with a gain of 12 dB. It is mainly a 2nd order LC parallel resonant filter with some R ratio gain. The 12 pF reduces the Q peaking from 15 dB to 12 dB.
The GBW must be at least 4 MHz and preferably 10 MHz minimum from my RoT (Rule of Thumb).
GBW = Qmax^2 * Av * fo= 64 * 3.3 * 20 kHz
If we had to use an inductor, it would be quite large in physical size to achieve a low ESR <= 10 mohm and 50x times the cost of a cap, roughly.
ref This is a rough approximation of a similar LC filter.
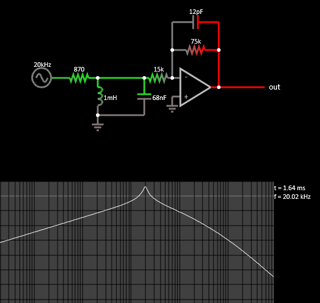
This does not mean you can pass 10 Amps thru the Inductance "C4". Something might explode. (LOL)
But the Op Amp draws current from supply to do the same as the inductor, except this is an inverting NIC. or INIC.
Welcome to EE.SE.
The block highlighted in red is an active bandpass filter, which C3 and C4 are part of. See https://www.electronics-tutorials.ws/filter/filter_7.html under Infinite Gain Multiple Feedback Active Filter. Presumably it is tuned to 40 kHz. I haven't checked but you are welcome to plug the component values into the formula given in the link.
C2 rolls off the gain at high frequency (>> 40 kHz) to improve the stability of the filter i.e. prevent it oscillating.
That filter topology is called a narrow-band multiple-feedback (MFB) band-pass filter.
C3 and C4 are both part of the band pass filter and define the pass band frequency range.
This is one of the known methods to improve the damping characteristics of a second-order bandpass.
With the exception of C2, all the other parts resemble the well-known second-order bandpass in multi-feedback-topology.
The additional capacitor C2 adds an additional lowpass function to the circuit with a pole at app. fp=180 kHz [wp=1/T=1/(12p*75k)]. This effect can be seen in the BODE diagram: The rising slope is +20dB/dec whereas the falling slope of the magnitude approaches -40dB/dec.
