Does a voltage drop exist in a current-carrying loop of wire?

Question

Suppose current is induced in a loop of wire by a changing magnetic flux, would there be a voltage drop along the wire?

If a voltage drop does exist then somewhere along the length of the wire there should be a jump in voltage otherwise it would violate Kirchoff's voltage law, but considering the symmetry I do not think there is a point where voltage jumps.

In the other case, if there is no voltage drop, wouldn't that violate Ohm's law because current times resistance gives you some voltage drop not equal to zero?

What is the way out of this problem?

Thank you.

Answer 1

There is both a voltage drop along the loop, due to the current flow times resistance per length, and a voltage rise along the loop, due to the changing magnetic induction. They will be equal and opposite, and add to zero, resulting in the loop being equipotential all the way round, for a uniform loop.

If the loop is non-uniform, for instance heavy wire 95% of the way round, and a thin resistive wire or even a discrete resistor for the other 5%, then you'd see a significant voltage drop across the higher resistance section.

As a practical issue, you would find it difficult to make a convincing measurement of the voltage drop from point to point of the loop, as you would need to connect your DVM to two points on the loop by test leads that make their own loop. How you run this loop with respect to the area of changing magnetic field will influence the voltage generated in the meter-lead loop, and so added to the voltage actually present at the probe points.

Answer 2

Lets say you have a coil of wire that is 1000 ft long @ 30 AWG thickness, but no isolation, just carefully wrapped around a insulating core without touching. Now in order to have current flow, you need to complete the circuit, so add a resistor to the end wires. Now induce a current with the magnetic flux. Certainly you can imaging having lots of wire resistance and voltage drop at both ends with this experiment. Now move the voltage probe from the end and touch each loop, and you will see reduced voltage.

Now change the coil to one single loop, but still 1000 ft long @ 30 AWG. You will observe the same inform voltage drop along the wire.

Now change the loop to 1 ft and 12 AWG. It works the same, just smaller voltage and more difficult to detect it.

Answer 3

Suppose current is induced in a loop of wire by a changing magnetic flux, would there be the voltage drop along the wire ?

The answer may depend upon what you mean by "voltage drop along a path".

Consider two points, A and B, and a curve that goes from A to B. One definition you may use for "the voltage drop from A to B along path C" is the work done by an electric field while moving a unit charge from A to B along C.

In mathematical notation,

$$V_{C:A\rightarrow B} = \int_{C:A\rightarrow B}\vec{E} \cdot d\vec{\ell}$$

where \$\vec{E}\$ is the (total) electric field vector.

Using this as the definition of "voltage drop" there IS a voltage drop along the wire. (Its value happens to equal the current through the loop times the resistance of the section of the wire under consideration.)

Note, however, that in this case, because there is voltage induced by a changing magnetic field, the voltage drop along the wire will be path dependent. Since we are assuming a closed loop of wire, there are two paths between the starting point A, and the end point B. That is, if we cut the wire at points A and B, we would have two pieces of wire, each corresponding to a different path from A to B.

Now, when there are no time-varying magnetic fields involved, then the voltage drop from A to B will be path-independent. In that case, we say that there is a potential difference between A and B. However, that is not the case here, so, for the moment, we will say no more about potential differences.

Kirchhoff's Voltage Law

If voltage drop does exist then somewhere along the length of the wire there should be a jump in voltage otherwise it would violate Kirchoff's voltage law,

No. Kirchhoff's Voltage Law states that in any loop composed of paths 1, 2, 3,...n, the sum of the emf's (electro-motive forces) encountered in 1, 2, 3,...n, is equal to the sum of the products of the currents in 1, 2, 3,...,n times the resistances of the paths 1, 2, 3,...,n. That is

$$\mathscr{E}_1+\mathscr{E}_2+\mathscr{E}_3 + ... \mathscr{E}_n=I_1R_1 + I_2R_2 + I_3R_3 + ... I_nR_n$$

This relationship holds, however you choose to divide up the wire into segments. But there is no voltage drop "jump".

Pseudo-KVL

It is worth discussing why you might have thought differently.

Remember that I said that when no time-varying magnetic field is involved, the voltage drop between two points becomes path independent, and we have a potential difference?

Well, if KVL holds generally, then it also holds when no time-varying magnetic fields are involved. That means that the total voltage drop around any loop (when there are no magnetic fields involved) is zero!

Through a protracted game of telephone, Kirchhoff's voltage law, as Kirchhoff expressed it, became recast in the form

"The total voltage drop (as defined above) around any closed loop is 0"

or in mathematical symbols

$$\oint_C\vec{E}\cdot d\vec{\ell} = 0$$

I call this recasting of Kirchhoff's voltage law "pseudo-KVL".

Pseudo-KVL is a special case of KVL that holds whenever no time-varying magnetic fields are involved. However, it does not hold, when time-varying magnetic fields affect a circuit.

You were probably taught pseudo-KVL, and that is why you suspected that there would need to be a "voltage jump" if KVL held.

---

For those who are interested, the original German version of Kirchhoff's paper "Ueber den Durchgang eines electrischen stromes etc.", in which his two circuital laws are found, can be read for free at zenodo.org/record/2422851 (see page 513)

Answer 4

Due to other ongoing discussions I was having in other provinces of the metaverse, I originally interpreted your question in a very different setting, where the loop of wire is basically a single-turn transformer connected to a resistive load (potentially degenerating into an open circuit). I have now modified my answer to also consider what happens both in a closed uniformly resistive ring (which seems to be your question) and in the similar but strange case of a perfectly conductive ring, but I will retain and expand the rest to make this a more general answer. Links and figures (and possibly formatting and corrections) will be added as soon as I figure out how to make the GUI buttons visible (if ever).

Let me start anyway from the case of a loop of good conducting wire connected to a resistive load. The reason is that this is a more general situation where both components of the electric field (the induced field Eind and the coulombian field Ecoul) are nonzero, and this makes it easier to understand what is going on before considering the rather particular cases of uniform nonzero and zero resistivity. In the following, 'good conducting' means with an overall resistance that is negligible compared to that of the load.

A coil with a resistive load

To understand what is really going on inside the loop, it is better to look at the fields. It is also easier if we consider an (independently generated) variable magnetic field confined at the center of the coil (the simplifying hypothesis of an infinitely long solenoid generating the field) and a single turn coil that goes around it at a distance. At first I will consider a good conducting single turn coil closed on a resistive load (with the limit case of an open circuit). When the load is finite, a further simplification hypothesis (that can be removed, see note 4) is that the current flowing in the coil will not produce any appreciable flux; that is, we only consider the effect of mutual inductance and not self-inductance.

fig single turn coil with resisitive load - mutual inductance only
(to be added when I figure out how to make the GUI buttons visible)

In this settings, there is no significant voltage along the good conducting wire of a coil in quasistatic conditions. All voltage will appear where the load, or the gap, is located.

Looking at the fields
Let's look at the fields. The changing magnetic field located in a region inside the coil's is associated with a rotational induced electric field Eind around it. The coil, along with its load or even a gap, will be subject to this induced Eind field. This field will very quickly redistribute the free charge on the surface (and let's say in the interior) of the conductor in such a way that it will create a charge displacement with accumulation at the open terminals or at the interfaces with the resistor (some charge will in general be necessary on the lateral surfaces as well).

This is what emerges by solving Maxwell's equations along with the continuity equation and the constituent relation in the material (Ohm's law in its local form). Under rather general conditions, when a current is flowing in the material, we get that charge density is given by:

https://i.postimg.cc/CMNLych2/image-3.png

That is: for a given current density, charge density changes according to gradients in conductivity and permeability. A sketch of the derivation of this expression can be found towards the end of my answer here. but I botched a sign in the last two equations, I will correct that later. What is important to notice is that in obtaining the above result, the electric field used is the total electric field E = Etot, and that Ohm's law is obeyed in all parts of the ring (wires and resistors).

Now, this is the key to understand why the configuration of the resultant electric field changes from the externally imposed (magnetically induced, in our case) electric field: this surface and interface charge generates an electric field **Ecoul that is superposed to the induced electric field Eind.

In a loop with a resistive load and a perfect conductor as coil,

• the coulombian electric field inside the good conductor opposes the induced electric field in such a way that the resultant net electric field Etot = Eind + Ecoul is zero. This implies that there is neither a voltage build up (nor a voltage drop representing a loss) along the filament of the coil. [see footnote 2 for the case of finite resistivity, spoiler alert: Ohm's law will be obeyed and the voltage drop in a good conductor will still be negligible - in the case of a coil it's the ohmic drop in a piece of good conducting copper]
• Conversely, inside the resistor or in the space between the open terminals, sandwiched between opposing accumulations of charge, the coulombian electric field Ecoul compounds with the Eind field to build a strong resultant nonzero Etot. What you get is the full voltage that is basically equal to the path integral of Eind along the full extension of the coil.

The result is that you have zero voltage ALONG the coil and nonzero voltage (for example 5V) ACROSS the coil [see footnote 3 on how to correctly measure voltage]. And yes, the implication of this is that Kirchhoff Voltage Law will no longer apply because, in the presence of variable magnetic field, voltage becomes path dependent. [See footnote 5 for references, or my answers here, here, and here but keep in mind that I have been using different notations from the one used in this answer]

I happen to have ready the pictures with the decomposition of the electric field in the solenoidal and conservative components Eind and Ecoul in and outside a single turn coil with two load resistors on the opposite sides of the magnetic field region (what we can call the Zahn - Romer - Lewin ring [see footnote 5 for references])

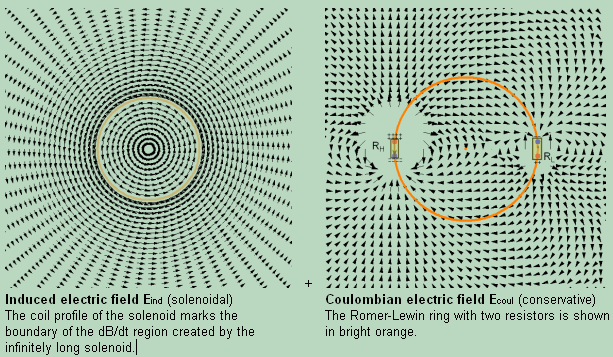
fig the Eind and Ecoul fields around the ring

and for the total and component fields Etot, Eind and Ecoul, inside the ring.

The dots inside the first ring represent the negligible (ideally zero) total electric field Etot in the conductor arcs.

The Helmoltz decomosition
This decomposition of the electric field, the Helmoltz decomposition, is mirrored by a decomposition of the actual voltage (computed as work per unit charge) into two partial components. Instead of just passively split the path integral for Etot**.dl** into the two components E+coul*.dl** and Eind*+.dl**,

voltage = induced voltage + scalar potential difference

we can gain some insight by starting from Faraday's law expressed in integral form (remember that Etot is the one and only electric field present in a given point at a given instant of time)

and considering that the surface integral that expresses the time derivative of the flux can be turned, by using B = curl A and applying Stoke's theorem, into the path integral of the magnetic vector potential A.

The right hand side represents the contribution of the induced field Eind. Now, the interesting consequence of this manipulation is that (assuming everything is mechanically stationary so that the contour of integration does not depend on t) if we rearrange the terms in this way

we now see that the circulation of Etot - (-dA/dt) is zero. This is Ecoul, the component of the actual field that is irrotational and admits a potential function (the electric scalar potential phi). Ecoul can be recovered from the actual electric field Etot by subtracting the term -dA/dt (corresponding to the induced electric field Eind). That double minus sign that becomes a plus sign can lead to the illusion that Ecoul, when written as Ecoul = E + dA/dt is some sort of "resultant" field, but it is not. It is A PART of the one and only total electric field, the electric field any charge experience at time t in the point (x,y,t), i.e. Etot.

We have therefore determined that

Ecoul = - grad phi ---->(partial component)
Eind = - dA/dt ---->(partial component)
Etot = - grad phi - dA/dt ---->(the real deal)

and therefore the actual path-dependent voltage in the complete physical system can be split in a component of path-independent voltage due to the distribution of charges (the scalar potential difference) and a second component of voltage due to the effect of (nearby) changing magnetic fields.

Using VBA_gamma for the actual voltage that depends on the path gamma, phi for the electric scalar potential, and rearranging the expression a bit, we arrive at the following decomposition of voltage (the work done per unit charge that considers the complete physical system and obeys Ohm's law):

voltage = scalar potential difference + path integral (dA/dt).dl

A... potential source of confusion
Unfortunately, some textbook authors (most of them, probably) like to call the scalar potential difference with the generic term 'voltage' because in electrostatics, where the concept of voltage is first introduced to students, the definition of voltage as work per unit charge is identical to the difference in potential. In electrostatics, the electric field E is conservative and admits a potential function that said authors denote with the symbol V, the same symbol use to denote the work done per unit charge. I believe this choice can only cause confusion and that it would be better to separate the concepts of voltage and scalar potential difference right from the start. The standard developing body IEC (International Electrotechnical Commission) has chosen this approach: (links to be added etc. etc):

IEC definition of voltage
IEC definition of (scalar) potential difference
IEC definition of induced voltage

(if your positive voltage 'arrows' go from low to high, you might want to add a minus sign to the above definitions). Definitions and conventions are almost always just a matter of tastes. But what is not a matter of tastes is the fact that, even if you choose to call the scalar potential phi with the letter V, this potential alone is not sufficient to fully describe the configuration of the total electric field in the presence of nearby variable magnetic fields. It will only describe the conservative component Ecoul, basically giving you an incomplete description of what happens in parts of your circuit that are just near a variable magnetic flux region, even without being inside the region itself. In fact, the authors that choose to identify 'voltage' with what the IEC calls the (scalar) potential always clarify that you need both the (electric scalar) potential "V" and the magnetic vector potential A to fully describe your system even if all you are interested in is the electric field, since in their notation:

Etot = - grad "V" - dA/dt (this notation can lead to confusion)

Note 1: A drawback of using the scalar potential

The Helmoltz decomposition is not a big deal in electrodynamics, where one usually gets to compute the fields everywhere (sometimes analytically, more often numerically) but can be cumbersome to use with practical circuits, unless there is some special and almost unique geometry that simplifies the computation of the path integral of Eind along the coil.
Consider for instance the - quite common - case of a system where the induced electric field Eind has some asymmetrical configuration. For example, the induced electric field associated with a toroidal transfomer is not going into symmetrical centered circumferences around a rectangular section toroid)

fig using the scalar potential difference with a big and small load resistor around toroidal core
big resistor in two positions
small resistor in two positions

You will find for the scalar potential difference values across the load resistor will depend on the size of the resistor and on its position relative to the core. And of course you cannot use Ohm's law with the scalar potential difference alone. In short, in the presence of variable magnetic fields, the scalar potential does not behave as one would expect the electrostatic potential difference to behave.

Note 2: Accounting for the finite resistivity of copper

If you want to consider the role of the finite resistance of a copper coil when it is closed on a resistive load, you will observe just a small ohmic voltage drop of a handful of millivolts per meter (probably less, dependending on the section of the wire and the current carried) but nothing even comparable to the voltage across a non-shorted coil. This means the superposition of the induced electric field Eind and the coulombian electric field Ecoul in the conductor of your loop will not be zero but will instead be the value Etot = j / sigma, where sigma is the extremely high conductivity of copper. This is a small value that is normally considered negligible when compared to the voltage you can measure between the terminals of your loop, across the load resistor.

fig single loop with resistor case perfect conducting wires - case finite conductivity wires

Even when the coil has finite resistivity, there is no voltage build up in the conductor of the coil. Yes, if the resistivity is high you will see an appreciable voltage drop in the coil, but this is just 'a loss' and not the voltage build up that justifies the voltage you see at the load's terminals.

Note 2 1/2: A uniform closed loop of resistive wire

If the loop is completely made of uniform resistive material there cannot be any gradient in conductivity or permeability along the whole closed loop (inside the loop there are no longer interfaces between conducting parts and resistive parts), so you won't be able to see any accumulation of charge inside the ring that will justify jumps in voltage (or even in scalar potential difference).

In the case of a circular ring of uniform resistive material perfectly centered around a circular region of spatially uniform changing magnetic flux, the Eind field inside the material will be directed along the circumference and the Ecoul field will be zero. The resultant net electric field Etot = Eind will comply with the local form of Ohm's law for the material - that is the current density in the loop will adapt to the value j = Eind / sigma and will follow the direction of the loop. It is this field that gives rise to the voltage along any arc of the ring [see note 3 on how to measure it], while the scalar potential difference will be zero for any two points on the ring.

fig total field inside and outside a uniform resistive ring with perfect symmetry Etot = Eind At least in 2D, Ecoul is not needed to comply with Ohm's law inside the material (it probably will not be needed for a ring with square cross section that will follow the cylidrical symmetry of the induced Eind field, but expect surface charge to show up in the case of a circular, or worse, irregularly shaped cross section of the wire.)

The voltage - associated with the nonconservative field Etot = Eind - will be path dependent and it will not obey KVL. Case in... point, pick two points A and B diametrically opposed on the ring and the voltage between A and B along one branch of the loop will be the negative of the voltage between A and B along the other branch. The sum of the voltages along the closed loop will be the difference of these values and will add up to the EMF linked by the loop.

Note that there still are discontinuities between the material and the space around it and so there still is the possibility for charge to appear on the surface of the lossy conductor that makes up the loop, especially if there is no special symmetry between the shape of the loop and the configuration of the induced electric field it is bathed in.This charge acts as sources and sinks for the conservative electric field Ecoul, which will be present in the space around the ring of material, disturbing the exciting Eind field, much like in the case of electrostatic induction.

fig the total field inside and outside the uniform resistive ring with broken symmetry

If the resistive ring is closed so that a current can flow, in this asymmetric situation surface charge will distribute along the ring in order to produce a coulombian field inside the ring as well, in such a way that it will superpose to the asymmetrically distributed induced electric field to ensure that Ohm's law is obeyed by the resultant Etot = j / sigma inside the ring (where j is directed along the conductor and, for small enough frequencies so that we can neglect the skin effect, has magnitude given by I / area of the cross section of the conductor, something we can compute as Etot = (linked EMF/total resistance of the ring) / area of the cross section.)

If you create a gap in a uniformly resistive ring, you break the symmetry in a very abrupt way. Instant of time after instant of time, surface charge will in large part accumulate at the terminals, producing an Ecoul field that will exactly compensate the Eind field inside the ring and will disturb the induced field on the outside. Now Etot = 0 inside the material, a value compatible with the absence of current flowing, while on the outside it will be not zero. In particular, the resultant field Etot = Eind + Ecoul will be responsible for the voltage you can read between the terminals (for example along the path A-->B in the following figure).

https://i.postimg.cc/g00pypFq/screenshot-2.png
fig magnetoquasi static induction, ring broken Etot is zero inside but nonzero outside (in particular, Etot will be strong between 'terminals', near the small gap)

If you measure the voltage along the coil [again, see note 3], you will read exactly zero volts, a value that obeys Ohm's law for the case of zero current flowing. The scalar potential difference between two points on the coil, on the other hand, will be nonzero even if no current is flowing in the finite resistivity material. Proving once more that using the scalar potential difference alone breaks Ohm's law.

As a side note: you can compare this behavior with what happens in the case of electrostatic induction. There, a conservative exciting electric field Eext makes charges on the surface of the conductor react in order to make the total electric field inside the material zero. If you compute the total voltage between any two points on the surface of the conductor, you always will get zero (since, being the total field conservative, voltage is path independent and equal to the difference in electrostatic potential - which is the same on the equipotential surface of the conductor). But if you consider the voltage associated with the component of the total field that is generated by the charges on the conductor alone, you would get a voltage difference between points on the surface of the conductor. (See my answer here for a picture of the two phenomena side by side. Yes again the links will appear when or if I can get this darn interface to work - good job with the GUI, fellas - I am sure it streamlined your work a lot - too bad you will lose contributors on this)

figure showing electrostatic and magnetoquasistatic with externally impressed field, field generated by surface and interface charges and total electric field

Note 2 5/8: superconducting loop

A uniformly perfectly conducting loop poses some extra problems because inside the perfectly conducting material there cannot be any resultant electric field Etot. This means that the surface charge will redistribute in such a way as to produce a coulombian field Ecoul that completely obliterates Eind everywhere inside the ring. The conclusion is that you cannot have a current flowing inside the ring.

fig superconducting ring and zero field inside, therefore zero current

I believe that the Eind field is also never 'seen' inside the ring, in the sense that free charge inside does not even need to react to it: all the charge movement happens on the surface before any Eind has any chance to be present inside the material. If you slowly bring the ring towards a region of space where there is an inducing Eind field, the surface will react to it as soon as it is exposed to it, preventing any electric field from getting inside; while if you 'turn on' the changing magnetic field where the ring sits still, the ring will have to wait for Eind to propagate there from the source, hitting its external surface first. Either way the surface reacts first by quickly rearranging surface charge in a way that will prevent a net electric field to ever be inside the perfectly conducting material.

A problem of this kind was proposed by Walter Lewin here, and the solution is given here and here. (links will appear when I figure out how to make the gui buttons appear)

Note 3: Measuring the voltage along the loop

Whatever the resistivity of the coil material, you can easily measure the voltage drop along it (and for many paths across it) by placing your voltmeter and probes in such a manner that the 'measurement loop' formed by voltmeter, probes and arc of loop you want to measure the voltage of, does not cut any variable flux. If you can visualize your system in two dimensions, this is the same as saying that the measurement loop must not go around the dB/dt region. Two examples are given in the following figures: (again, to be added when I figure out how to make the GUI buttons visible)

fig measurement loop for arc of coil, load, jump at single open coil

fig uniform resistive coil from the left, from the right

If your voltmeter has an internal resistance high enough not to disturb the original flow of current, it does not matter that you can form other loops with the attached remaining circuit that will include the variable flux region. What matters is that your measurement loop does not cut any changing flux. If that is the case, KVL works just fine in your measurement loop and the voltage ALONG the arc of coil that is part of it is also the voltage read by your voltmeter.

If you cannot devise such a lucky flux-free measurement loop, do not despair: you can still apply Faraday's law and detract (with due sign) the linked EMF from what you read on your voltmeter. For example, in the case of a multitap transformer, you can consider your voltmeter and probes as part of two distinct measurement loops: one completed by the jump in the space between tap points, and the other completed by the coil filament around the core.

fig multitap transformer: two measurement loops, same result

What you read on the voltmeter is the voltage in the space across the tap points (flux-free measurement loop); if you want to know the voltage along the filament between tap points you need to account with due sign for the linked EMF. You will end up with the value corresponding to Rcoil * I where Rcoil is the resistance of the portion of coil between tap points, and I is the current flowing in it. If the voltmeter is ideal and is the only thing attached to the coil, then I = 0, so the voltage across that part of the coil will be zero. In general, when a significant nonzero current is flowing (because the coil is attached to a load) you will measure a very very small voltage drop, comparable of what you will measure in a straight piece of copper wire of the same length when the the same current flows through it.

Note 4: self-inductance

If the current in the loop is sufficiently rapidly varying, the effects of the mutual induction from the primary source of flux will be counteracted by those associated the self-inductance of the ring. The externally created changing flux will create a (changing) current in the loop that will be responsible for the generation of a (changing) flux that opposes the original change. I am here assuming the loop does not contain, nor is part of a circuit that contains, generators of any kind.

Note 5: References

Some additional reading

J Roche (paper)
Explaining electromagnetic induction: a critical re-examination. The clinical value of history in physics.
Physics Education, Volume 22, Number 2 - IOP Publishing Ltd
considers all components of voltage: from mutual or self induction, from resistive losses and, obviously, from the coulombian field of the charges (what he calls the 'masking electrostatic potential difference')

Purcell & Morin
Electricity and Magnetism
Berkeley Physics volume II, 3rd edition
2013, Cambridge University Press
For certifying the death of KVL and the nonuniqueness of voltage (p.359) ; it also features the ring with two resistors as a solved exercise (p. 710).

Zoya Popovic, Branko D. Popovic
Introductory Electromagnetics
1999, Prentice Hall
a gentle introduction to undergrad EM; for the decomposition of voltage, see sec. 14.4 "Potential difference and voltage in a time-varying electric and magnetic field" (yes, he uses the symbol V for the scalar potential, but he makes the decomposition of the actual voltage explicit - see my already mentioned answer here.)

J. A. Brandão Faria
Electromagnetic Foundations of Electrical Engineering
2008, Wiley
lots of interesting examples, but mentioned here because it's another textbook showing the decomposition of voltage and the nonuniqueness of voltage. It uses the 'German' notation for the arrows that represent voltage across a component - some might prefer it.

Markus Zahn
Electromagnetic Field Theory: A Problem Solving Approach
1979 Wiley - 2003 Krieger Publishing Company
a good intro textbook with many examples, including the ring with two resistors - predates Romer
The full textbook is freely downloadable from the MIT OCW website

Robert H. Romer (paper)
What do voltmeters measure? Faraday's law in multiply connected regions
American Journal of Physics vol 50, no 12, December 1982
for the ring with two resistors and what a voltmeter actually measures, a very often cited paper

Herman A. Haus, James R. Melcher
Electromagnetic Fields and Energy
1989, Prentice Hall
for nonuniqueness of voltage and the role of surface charge in the multitap coils
This text, too, is freely available on the MIT OCW website

Ramo, Whinnery, VanDuzer
Fields and Waves in Communication Electronics 3rd ed.
1994, Wiley
An engineer's point of view on the multivaluedness of voltage and when it is still possible to pretend it's singlevalued, like a potential difference.

Answer 5

The problem with your question is that you state a false premise, i.e. that the loop follows KVL.

KVL and KCL are only valid for lumped circuits, i.e. for circuits made of components (whose dimensions are irrelevant) connected with ideal wires. Step out of these premises and you will start experiencing "weird" contradictions in your reasoning.

The fact is that KVL derives from a more general law, which is part of Maxwell's equations, i.e. Faraday's law (see this).

What Faraday's law states is that a current is induced in a closed loop if the linked magnetic flux changes with time. If the loop is made of non-superconducting wire, it will have some resistance and the integral of the E field along that loop will be non-zero. This means the E field will perform some work to make that current do its merry-go-round.

Since Ohm's law is still valid (microscopically), that work (per unit time) will be equal to the R^2*I power dissipated in the loop. However you can't get a "voltage across two points" that has the same meaning of "voltage across a resistor", since the resistance is not lumped in a single element, but it's distributed along the loop.

Bottom line: there is no terminals across which you could find the induced voltage, as you would expect with KVL. You only have the circulation of E field along the loop.

Answer 6

Faraday's law of induction is about voltage being induced (not current). Current may flow but will only flow as a result of the loop being closed either with a short or a load AND the induced voltage being present.

Either way, there is still an induced voltage.