Coaxial Cable with signal applied to shield and center conductor grounded

Question

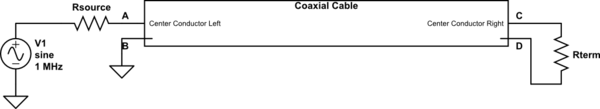
The standard way to drive a signal through a coaxial cable is to have the shield grounded, and a single-ended signal applied to the center conductor.

(Throughout, I will assume that \$R_{term}\$ and \$R_{source}\$ match the characteristic impedance of the coax, so that there are no reflections.)

Referring to coaxial cable, the article "Magnetic Coupling In Transmission Lines and Transformers" states that

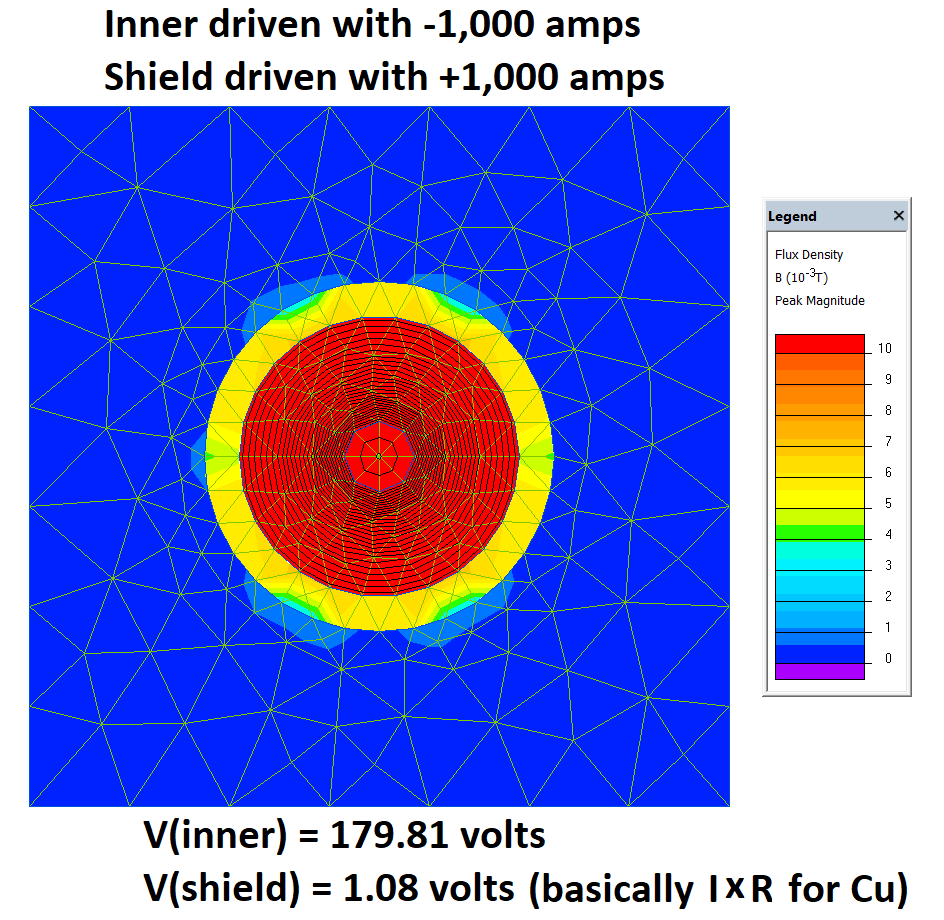
Because of the absence of flux loops from the outer conductor in its interior, the self-inductance of the outer conductor exactly equals the mutual inductance between the two conductors.

This seems incontestable.

The paper goes on to explain

What this means is that no longitudinal voltage will exist on the outer conductor due to inductance, if the inner and outer conductor currents are equal and opposite!

Which also seems incontestable. If the currents in the inner and outer conductors are equal and opposite, and the self-inductance of the outer conductor or shield is equal to the mutual inductance between the inner and outer conductors, then the total voltage magnetically induced by the inner and outer conductor currents on the outer conductor will be zero.

Consequently,

there will be no voltage difference between the source and load ends of the outer conductor due to inductance.

[However, there could be a voltage difference between the source and load ends of the outer conductor due to resistive voltage drop.]

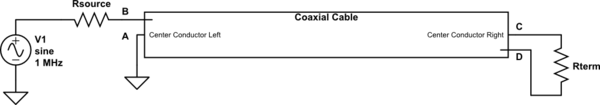
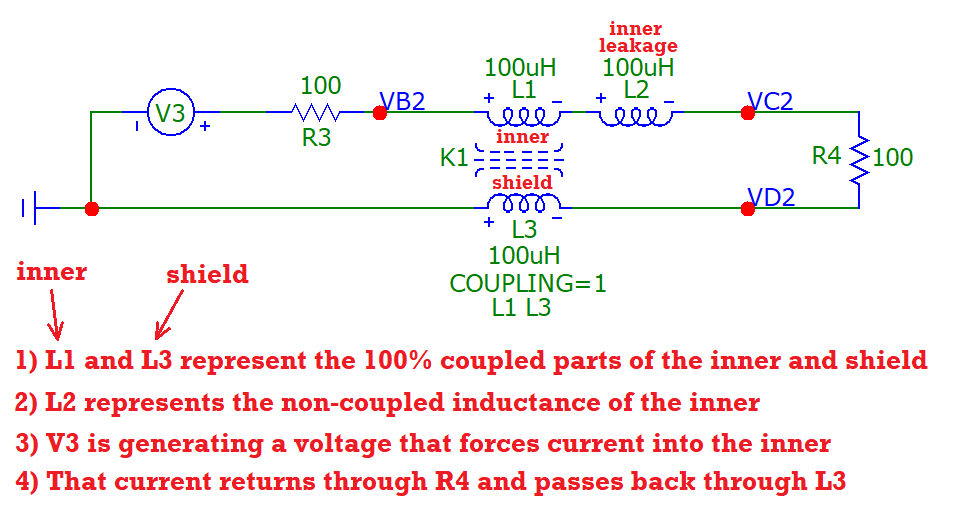
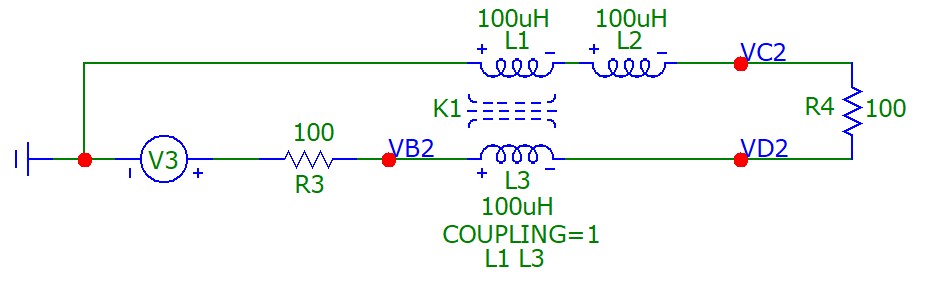
Now let's consider the case where the coaxial cable is wired up "incorrectly", that is, with the center conductor grounded, and with an AC signal applied to the outer conductor.

When a coaxial cable has particular lengths related to the frequency of a signal, the voltages at either end may be in phase due to some multiple of 2\$pi\$ phase shift occurring along the length of the cable. Let us assume that is not the case.

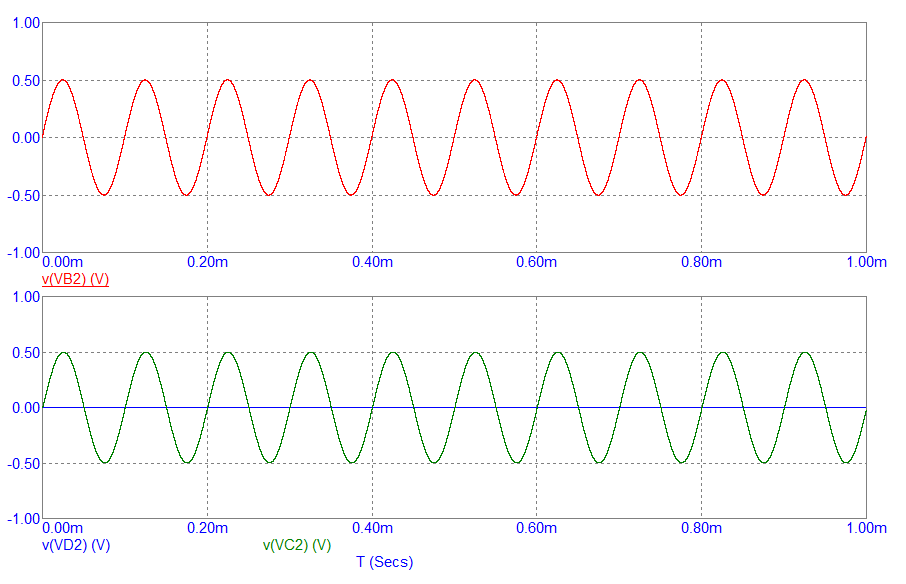
If we discount the possibility that the voltage at B is in phase with the voltage at D, and we discount the possibility that the voltages at B and D are constant, we seem to be left with the choice that the voltages of B and D are out of phase.

According to the analysis above, if the currents in the inner and outer conductors are equal and opposite, then there will be only a negligible resistive voltage drop across the outer conductor. However, since the voltages at B and D are out of phase, there must be a non-negligible voltage difference between them.

However, if there is a non-negligible voltage difference between B and D, this apparently leaves us with the conclusion that the currents in the inner and outer conductors cannot be equal opposite.

My questions

1. Is this reasoning correct?
2. If this reasoning is correct, how can one explain the different currents in the two conductors?
3. If this reasoning is incorrect, where does it go wrong?

Odds and Ends

This question arose for me as I am working to understand this EESE answer.

---

Edit:

At this point, I believe that the reasoning is in error, and the source of the error lies in discounting the possibility that the voltage at B is in phase with the voltage at D. Another possibility, and the one that I believe happens in reality, is that the phase velocity of the voltage along the shield is infinite. At first my thoughts revolted against such an idea. How can fluctuations in the voltage at B instantly propagate to D?

However, upon reflection, I think this initial rejection of the idea that there is infinite phase velocity for the voltage between B and D is in error. My error lay in conflating the phase velocity of the voltage on the outer conductor relative to ground, with the phase velocity of the voltage on the outer conductor relative to the inner conductor. They are not the same. The phase velocity of the voltage relative to ground can be infinite because what we choose to call "ground" is arbitrary. The phase difference between an arbitrary point and ground does not need to "propagate". So whether or not the voltage on the outer conductor relative to ground is "fluctuating" is relative to our choice of what to call "ground". Once I accept that it is OK for the phase velocity of the voltage on the outer conductor (relative to ground) can be infinite, then I can accept that the voltage drop between the ends of the outer conductor is negligible. Once I accept that, I am no longer logically obliged to reject the fact that the currents in the inner and outer conductors are equal and opposite. All becomes right in the world.

Answer 1

As we need to consider voltages from end to end of the line, we need a reference from one end to the other. Let's avoid the case of the outer being considered the 'inner' of another line with respect to ground, and instead consider the simpler case with the coax looped back so the input and the output are effectively co-sited.

Several things are still true in comparison to the driven inner case.

• The currents in the inner and outer are equal and opposite
• They couple no flux to the outside world
• The differential signal voltage at AB becomes the differential voltage at CD some time later.
• The only difference is that there is a strong capacitive coupling from the signal line to the outside world.

It's this feature that means we (almost) invariably designate the outer shield as the ground conductor when using unbalanced co-ax. There is as much symmetry for currents in unbalanced coax as there is for a balanced line like twisted pair. The asymmetry only manifests itself for capacitive coupling.

However, there is an inductance for the loop of wire AD. It's a loop, and no magic is happening. There's also an inductance for the loop BC. It's that when we drive AB differentially, we don't couple into the loop AD or BC, because of the equal and opposite currents thang. We describe the inductance from end to end of the coax as a common mode inductance.

Having a finite inductance AD means we can support a voltage AD. The easiest way to understand 'driven outer' coaxial cable arrangements, and they are used in RF components as 'baluns' specifically to use this AD voltage, is to regard the transmission line as a transformer. Here I've drawn what is often known as a 'transmission line' transformer. The wikipedia entry doesn't do it justice, I'm sure an app note from a mixer or balun manufacturer could be found which did a better job.

^{simulate this circuit – Schematic created using CircuitLab}

By looking at the transformer, we can see that the AD inductance can be equated with the transformer's primary inductance, and BC the secondary. As such, there is no low impedance control of voltage at the far end of the line, for both the inner and the outer!

Note I've not applied a ground symbol at the receiving end. If I ground the lower end of the load, I get a non-inverting transmission line transformer. If I ground the upper end, I get an inverting one, which 'works' for all frequencies where the common mode inductance is significant.

The same goes for the source ground, by reciprocity. I can replace those ground symbols by a direct connection, and choose to ground either side of the transmission line input, or leave both sides high impedance.

So why do we ground the far end of a conventionally driven coax? Usually to get the signal (inner) voltage to be referenced to the ground at the end of the cable, where we want to use it. Without that ground connection, it would be a very high impedance signal, referenced to the sending end, able to pick up any kind of magnetic coupling interference between sender and receiver.

Another way to arrive at the 'all (most of) the return current flows along the coax outer' result for conventional cable use is to notice that this common mode inductance exists in the way of unbalanced currents. As the frequency increases, the effect of this inductance increases. At DC of course, the inductance isn't 'working' and we get the resistive result.

Although this common mode inductance is always present and will always have some effect, it often isn't enough, or isn't predictable enough, to make a usable transformer component. In such cases, it's boosted by either winding the coax several times through a ferrite toroidal core (wideband), or by choosing the length of the coax to be λ/4 long (narrowband).

Answer 2

---

$$\color{red}{\text{Let me preface this answer with a little additional information.}}$$

Directly below are quotations from the original question that I had to debunk in order to place my answer on the correct foundations. Those are now changed in the latest version of the question so, anyone reading this should not be fixated by what might appear to be misquotes from me.

$$\color{red}{\text{End of preface}}$$

---

It seems necessary to pick through your words and make corrections...

Assuming the correctness of the notion that if the current in both conductors is equal then there will be negligible voltage drop across the outer conductor, we seem obliged to conclude that

The current in the two conductors are not equal; they are equal in magnitude but not equal in polarity; one travels left to right and the other travels right to left.

Apparent result: The current in the two conductors cannot be equal / in phase, because there is a voltage difference between the two ends of the outer conductor.

It is correct that they cannot be in-phase; they are equal in magnitude but exactly opposite in their phase relationship; one travels left to right and the other travels right to left.

It is incorrect to conclude that there is a voltage difference across the outer conductor (shield).

As I showed in this answer, if the currents are the same magnitude but of opposing phase, the magnetic flux density outside the shield is zero. And, we know that from the magnetic theory of current tubes (the shield is a tube): -

The above picture shows the inner (of a rather clumsily drawn 2D model of a coax) driven with -1,000 amps at 100 kHz. The outer (shield) is driven with +1,000 amps and 100 kHz.

The 2D field solver (QuickField) can also be used to measure the volt drops per metre in the simulation. So, across 1 metre of shield there is a volt drop of 1.08 volts RMS and, this is purely due to \$I\cdot R\$; I modelled the conductors using copper and it has a finite conductivity of 59600000 siemens per metre. You can do the math if you want but, there will be a small lossy volt-drop.

But, look at the volt-drop across the inner; it's about 180 volts RMS and, this is solely due to the magnetic field it produces inside the shield. You can see the flux density is "in the red".

And, of course, outside the shield there is no field. This proves that the shield inductance is virtually zero when two opposing currents of the same magnitude are used. But maybe a simulation will help using inductors: -

So now, the analysis: -

• VB2 (the driven inner to the left) is 1 volt p-p
• VC2 (the output of the inner to the right) is 1 volt p-p
• VD2 (the shield voltage at the right) is 0 volts

And, of course, the left end of the shield is also at 0 volts.

Given what I've said in the answer I linked, and the clear absence of external flux surrounding the shield AND the simulation, the only sensible conclusion is that, when there are equal and opposite currents flowing in shield and inner, the net inductance of the shield must be zero.

And here's what happens when I drive the shield instead of the inner: -

• On the left end of the shield is VB2 (1 volt p-p)
• On the right end of the shield is VD2 (1 volt p-p)
• They are in phase hence, the net voltage across the shield ends is zero

Answer 3

When a coaxial cable has particular lengths related to the frequency of a signal, the voltages at either end may be in phase due to some multiple of 2\$\pi\$ phase shift occurring along the length of the cable. Let us assume that is not the case.

If we discount the possibility that the voltage at B is in phase with the voltage at D, and we discount the possibility that the voltages at B and D are constant, the only alternative that seems to be left is that the voltages of B and D (with respect to ground) are out of phase.

According to the analysis above, if the currents in the inner and outer conductors are equal and opposite, then there will be only a negligible resistive voltage drop across the outer conductor. However, since the voltages at B and D (with respect to ground) are out of phase, there must be a non-negligible voltage difference between them.

However, if there is a non-negligible voltage difference between B and D, this apparently leaves us with the conclusion that the currents in the inner and outer conductors cannot be equal opposite.

1. Is this reasoning correct?

No, there is a mistaken assumption.

The voltages at each end of the shield are equal (exactly if we consider an idealized coaxial cable with inductance and capacitance but no resistance, and approximately if we consider a real coaxial cable).

Since the voltage of the shield at one end relative to ground is varying sinusoidally, and the voltage at the other end is equal or nearly equal on the other end, the phase of the voltage of the shield at the far end relative to ground will be equal or nearly equal to the phase of the voltage on the shield at the near end, relative to ground.

It seems that this implies that the sinusoidal signal must propagate from one end of the shield to the other. And if the voltages relative to ground are the same or nearly the same at each end, then the phase velocity of voltage along the shield relative to ground must be infinite, (or at least approach inifinity as the cable length approaches infinity).

However, we know that the phase velocity of the voltage difference between the two conductors cannot be infinite. It can exceed the speed of light, but it cannot be infinite. It seems reasonable at first to assume that if the phase velocity of the voltage difference between conductors cannot be infinite (nor even approach infinity) that the same must also apply to the voltage difference between a point on the shield and ground. But it is a mistake to assume this.

Let's look again at the two circuits, the one where the inner conductor is driven and the outer shield is grounded, and the other where the outer shield is driven and the inner conductor is grounded. The essential difference between them lies only in the placement of the ground symbol. However, the placement of the ground symbol has no physical significance at all, (unless the ground symbol is meant to represent a physical connection (say to a chassis, a "ground" wire, a grounding rod in the earth or something similar). That is, what we choose to call ground, or zero volts, is arbitrary.

Now, consider the following circuit.

^{simulate this circuit – Schematic created using CircuitLab}

There is a difference in voltage between \$V_{far}\$ and \$V_{near}\$, but I don't think we would say that there is a physical propagation of the sinusoidal voltage.

Now let us move the ground symbol (but make not physical changes to the circuit).

^{simulate this circuit}

\$V_{far}\$ now has a sinusoidal voltage with respect to ground. But this is not really the result of a sinusoidal signal propagation between the resistor and \$V_{far}\$. Furthermore, the phase at each point of the wire between the resistor and \$V_{far}\$ is the same, and so the phase velocity is infinite.

Returning to Coax

What is described above is exactly what is happening when the shield of a coaxial cable is "driven". It was a mistake to:

discount the possibility that the voltage at B [relative to ground] is in phase with the voltage at D [relative to ground].

They are in phase, despite the apparent infinite phase velocity (relative to ground). And therefore we can avoid the unhappy conclusion that the currents in the inner conductor and outer shield are not equal in magnitude and opposite in phase.

I think it is easy to get confused by the apparent infinite phase velocity, and it is worthwhile to ponder that an infinite phase velocity, where one voltage is referenced to another voltage which is physically distant, doesn't imply physical propagation of signal, nor does it violate any rules about propagation speeds in a transmission line.