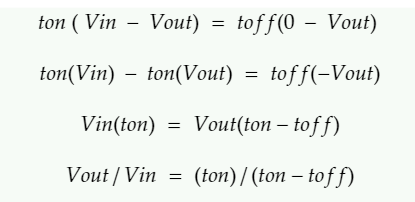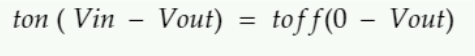Buck Converter Theory Confusion

Question

For a buck converter, I'm having a hard time conceptually understanding what is happening.

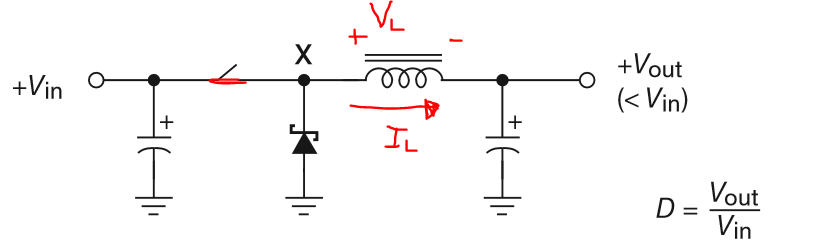
Here's my thought process: When the switch is closed, their is a positive voltage across the inductor equal to Vin - Vout. At this point there is a positive rate of change of current through the inductor.

This is the part I can't reconcile:

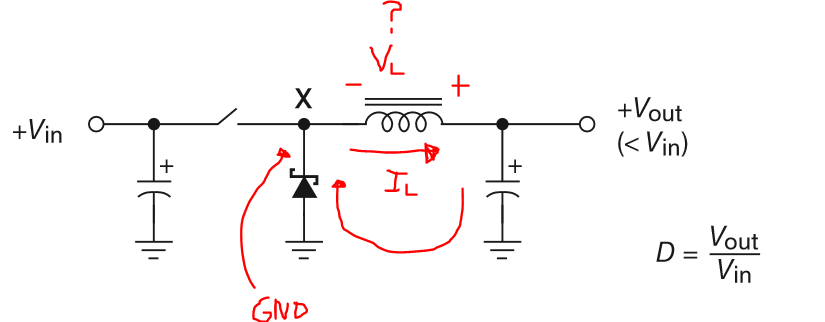
When the switch is closed, the rate of change of the current through the inductor is negative. We know from the state equation of an inductor (V = L di/dt) that if we have a negative rate of change for the current, than the voltage across the inductor must be negative as well. This is also corroborated by the fact that the average voltage across the inductor must be 0. So if the voltage across the inductor is positive when the switch is closed, it must be negative when the switch is open.

Keeping our same convention as before, this would imply that, at this point, there is a higher voltage at Vout than there is at Vin (making the voltage drop across the inductor negative).

I know this isn't right because if it were, our buck circuit would act more like a boost circuit - creating larger voltages at the output than input. Further, I know that, for a buck, Vout/Vin = Duty Cycle = ton/ton+toff, but my math below, given the assumptions discussed above, don't yield this result.

My math clearly has a backwards sign, which suggests that the polarity across the inductor is positive when the switch is open. I'm having a hard time intuitively reconciling these things.

Can someone help me understand what I'm missing conceptually?

Answer 1

That schematic you have there is a bit ambiguous: it uses an on/off switch for the series element, but a diode for the parallel one. If the diode would have been drawn as another switch, then things would have been clearer (I hope):

^{simulate this circuit – Schematic created using CircuitLab}

When SW2 is closed, the inductor is connected to ground with its negative end, thus the voltage at the output is solely due to the inductor.

Answer 2

The way you have defined your current \$V_L = -L \frac{dI_L}{dt}\$

for the conventional \$V = L \frac{dI}{dt}\$ the current I flows INTO the positive terminal of the inductor.

Answer 3

The average inductor voltage should be zero which means that V-sec of the inductor voltage should be balanced. As per your your derivation it is not true.. Volt-sec in Ton must be equal to Toff.. Therefore, it should be => ton(Vin-Vout)+toff(-Vout)=0 => Vin(ton)= Vout(T) => Vin/Vout = ton/T