If there is a power supply charging a capacitor (e.g. 4 μF) through a resistor (3.2 MΩ), the time constant can be calculated with the capacitance and resistance.
If I, however, measured the voltage across the capacitor, then that voltmeter in parallel adds a loading effect. How significant is this to the time constant assuming the internal resistance has the same order of magnitude as the resistor?
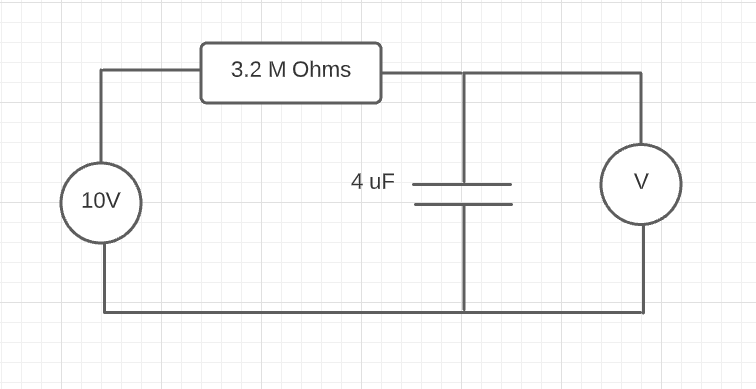
If the voltmeter's internal resistance is comparable to the 3.2 MOhm, then the loading effect is important and worth considering. We can form a Thévenin equivalent for the circuit attached to the capacitor (i.e. the voltage source, its series impedance, and the loading impedance of the voltmeter):
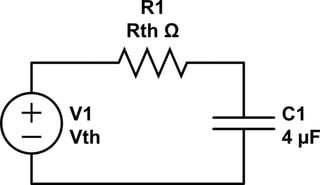
simulate this circuit – Schematic created using CircuitLab
where Vth and Rth are a consequence of both resistances. We can show that:
$$R_{th} = \left(\frac{1}{3.2\,[\text{M}\Omega]} + \frac{1}{R_{\text{voltmeter}}}\right)^{-1} $$
and
$$V_{th} = \frac{R_{\text{voltmeter}}}{3.2\,[\text{M}\Omega] + R_{\text{voltmeter}}}$$
You might recognize the second equation as being a simple voltage divider. However, it's worth noting that the capacitor charges to a lower voltage, but its time constant is shorter since it sees a smaller effective impedance across its terminals.
If you assume that the multimeter is a cheap entry level model, and it has same 3.2 Mohm load impedance as the source impedance, then the multimeter will eventually discharge the capacitor to equilibrium and it will read exactly 5V which half of the source voltage.
If the capacitor is assumed to be charged to 10V before connecting the multimeter, then the RC time constant of the new circuit is 1.6 Mohm * 4 uF is 6.4 seconds. It takes approximately 5 time constants, or 32 seconds, to discharge the capacitor to within 1% of the final result, and of course longer to discharge it to within 0.1% of the final result.
