BJT operation in saturation

Question

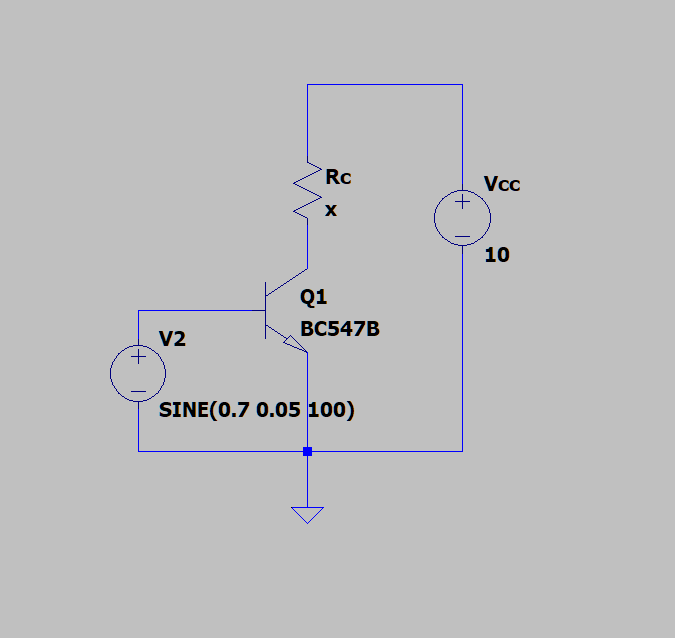
I am currently learning the fundamentals of BJTs from "Microelectronic cicuits" by Sedra and Smith. More specifically I am reading about how BJTs can be used as voltage amplifiers. From my understanding of the content, the process of amplification can be summarized by the following equations:-

$$ \begin{aligned} V_{CC} &= I_{C}R_{C} + V_{CE} \\ \rightarrow V_{CC} &= I_{C}R_{C} + V_{CB} + V_{BE} \\ &(\because V_{CE} = V_{CB} + V_{BE}) \\ \rightarrow V_{CC} &= I_{C}R_{C} + V_{CB} + 0.7 \\ &= const\ {\rm (from\ KVL)}\hspace{1cm}(1) \\ \end{aligned} $$

$$ {\rm and} $$

$$ I_{c} = I_{s} \cdot e^{V_{BE}/V_{T}}\hspace{1cm} (2) $$

Let us consider an example. Let the input signal be an AC voltage riding on a DC biasing voltage across the Base-Emitter junction. During a positive cycle of the AC, Vbe increases and this results in a corresponding increase in Ic (by Equation-2). This also leads to a greater potential drop across the Rc resistor as more current flows through it now. In order to compensate for the increase in Vbe and IcRc, Vcb decreases to maintain a constant voltage drop (Equation-1). Now if Vcb goes low enough, the transistor enters saturation. This results in a decrease in Ic (like a negative feedback loop). My question is: If the above is correct then how does a transistor actually go into saturation without the above stated feedback loop pushing it out of the loop?

Answer 1

This is from onsemi's BC547 datasheet:

As shown in figure 3, the more you go into saturation, the harder it becomes to pass the same collector current (i.e., the more base current you need). But that just means that I_C increases slower; as long as the source can supply enough base current, a higer voltage will always increase I_C.

Answer 2

You mentioned "Now if Vcb goes low enough, the transistor enters saturation. This results in a decrease in Ic " . This assumption is not true

When the transistor goes into saturation it attain the maximum Collector current possible in a given circuit (static DC situation. When coils and capacitors are involved its another story) .

If you keep pushing some current into the base by raising Vb you will inevitably keep staying in saturation status but absolute Vb and absolute Ve will increase if you had an Emitter resistor. Your circuit shows none.

Therefore, if you keep raising Vb you will quickly attain Maximum Ib and will eventually destroy your transistor. Until then, Vc remain at its lowest possible. Ic will not decrease until the transistor is destroyed. Keep in mind that Vb cannot raise more than 0.7 volt or so. At this point most devices are saturated.

Your formula may be correct but only when the Transistor in not in saturation. When in saturation the transistor is no more an amplifier but almost a simple On/Off switch in a circuit.