A battery with zero internal resistance connected to identical resistors in parallel

Question

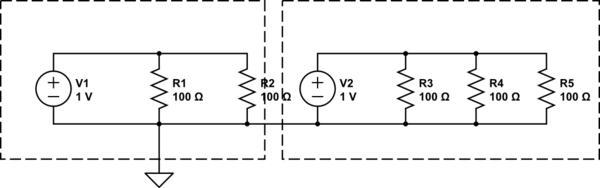
How is it possible that the current in each branch of a set of identical resistors in parallel would change when adding one more parallel resistor?

I know that since the battery doesn't have internal resistance (by assumption), its voltage would equal the voltage drop across the parallel combination, that is V_bat = V_ab = V_R1 = V_R2 = … = V_Rn.

V_bat is constant, therefore V_Rn is constant, and since V_Rn = I_Rn·Rn, then I_Rn should be constant.

So, if we say we have a parallel combination of n identical resistors, then I_Rn = I/n = constant.

However, adding resistor n+1 makes the new I_Rn = I/(n+1), but this would alter V_Rn since we know that Rn is constant, so that would then contradict the fact that V_Rn should equal the constant V_bat!

How this is possible?

Answer 1

The problem is that you assumed that \$I\$ stays the same. If you add another parallel resistor, then \$I\$ goes up.

Answer 2

You assume the total resistance of the circuit remains the same but this is not the case.Counter example:

^{simulate this circuit – Schematic created using CircuitLab}

the total resistance seen from the voltage source of the first circuit (from left to right) is 50Ω while the resistance seen from the voltage source of the second circuit (from left to right) IS 33.3Ω

Answer 3

Voltage is constant, the supply is ideal and wiring too. Therefore, each individual resistor has the same constant voltage over it, so the other resistors don't affect the voltage over each resistor and current through each resistor.

But the ideal wiring and ideal battery needs to output and deliver the total current to all the resistors. The more resistors you add, the more current flows in the wiring.

Answer 4

If the voltage source and connections are ideal, adding resistors in parallel will only affect the added resistor. Reality, of course, is different.

Similarly, adding resistors in series with an ideal current source would not affect the current, no matter how many you add.