How to calculate the natural frequency of a series LC circuit?

Question

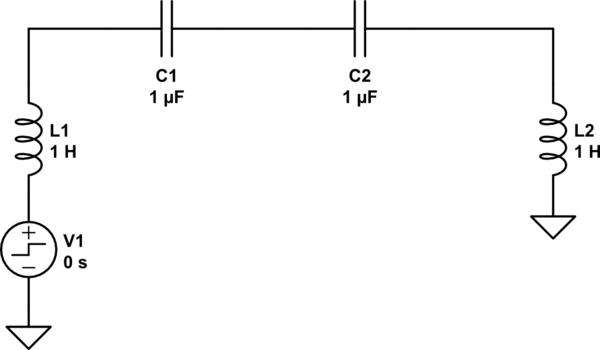
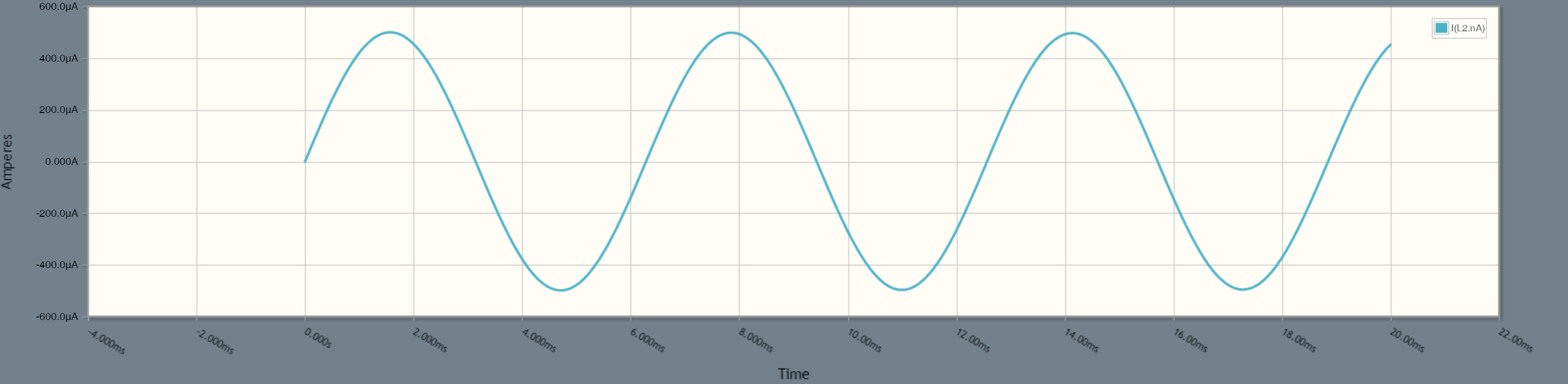
The below question is just to exemplify what I mean, I don't need help with this question, but rather the concepts behind it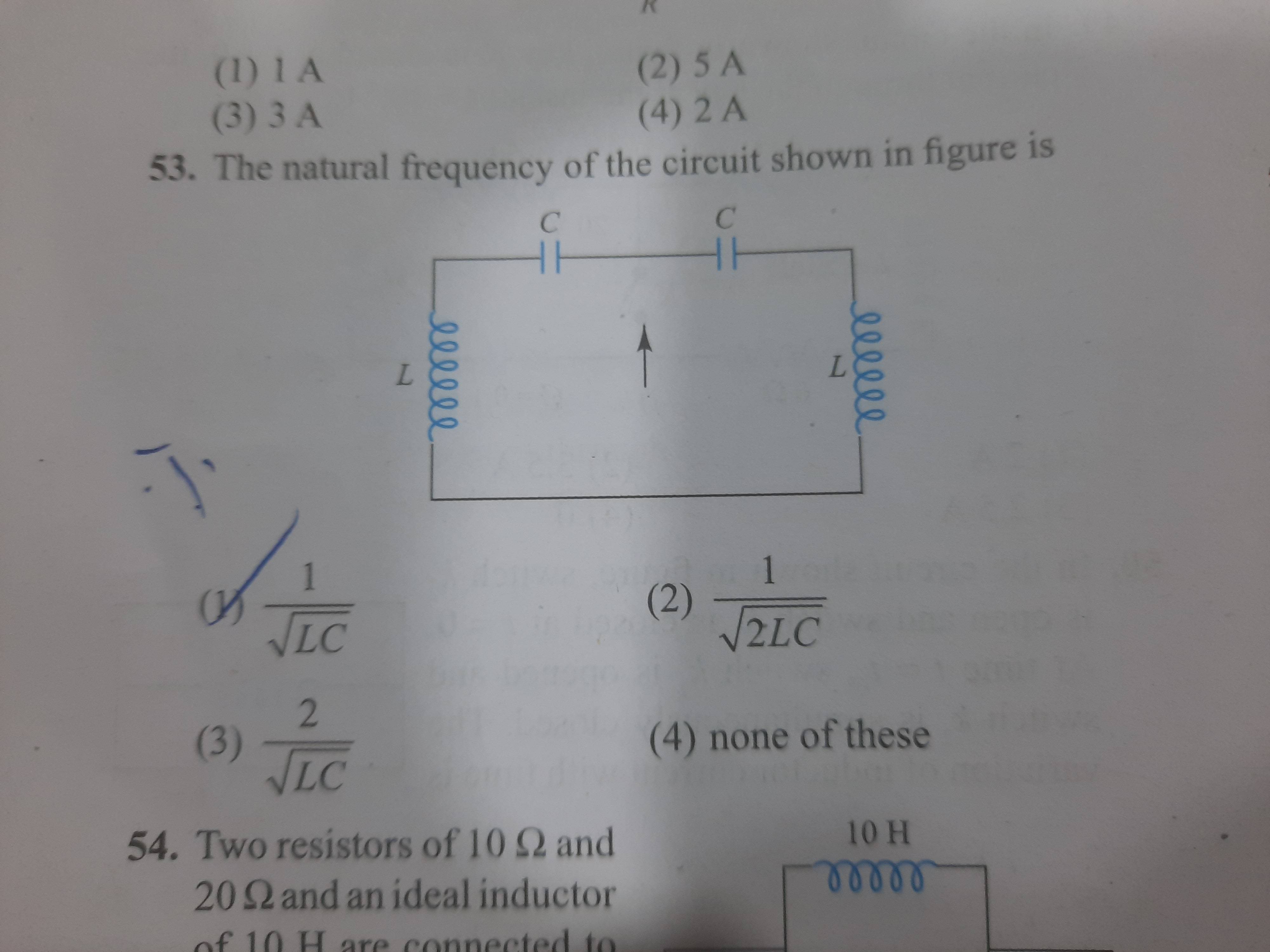

From the wwikipedia article on natural frequency

I concluded that for an LC circuit, the natural state would be that in which the current faces negligible resistance. Which would mean \$X_C=X_L\$

The capacitors are in series as are the inductors, from which, using the resonance condition for series LCR circuit, I got \$X_C=X_l\$

which gives \$ \omega = \frac{1}{ \sqrt{LC}}\$

however, I have a few doubts here

a) is my understanding of natural frequency correct? I have looked at a few answers on this site, but they usually end up talking about poles and other things that are beyond me at the moment. of which I have no idea.

b) If the capacitors were charged, would this be the frequency at which the charges would oscillate between the capacitor and the inductor(say the equivalent capacitor and inductor for simplicity)? If so , why?

Answer 1

\$X_L = \omega L\$

\$X_C = \frac{1}{\omega C}\$

Inductors in series add like resistors.

Capacitors in series are like resistors is parallel.

\$C = \frac{1}{\frac{1}{C_1} + \frac{1}{C_2}}\$

a) Yes, natural frequency is when \$X_L = X_C\$

Then \$\omega = \frac{1}{\sqrt{LC}}\$

b) Yes, assuming that a non-zero condition exists at the beginning, the energy will oscillate back and forth between the C and L. I suggest that you play with a simulator to see it in action.

Answer 2

The magnitudes of the reactances are the same, but of course the impedances are purely imaginary and the signs are opposite so they cancel out.

^{simulate this circuit – Schematic created using CircuitLab}

Here's an impulse input to the circuit in question. As you can see, \$\omega\$ = 1000, as expected.

"Why" is answered mathematically by the solution to the differential equation. Intuitively, maybe you can think of analogies with a pendulum or swing and potential vs. kinetic energy, because the energy really does slosh back and forth between the inductance and capacitance. At zero voltage across the capacitance all the energy is in the inductance, and at zero current all the energy is in the capacitance.