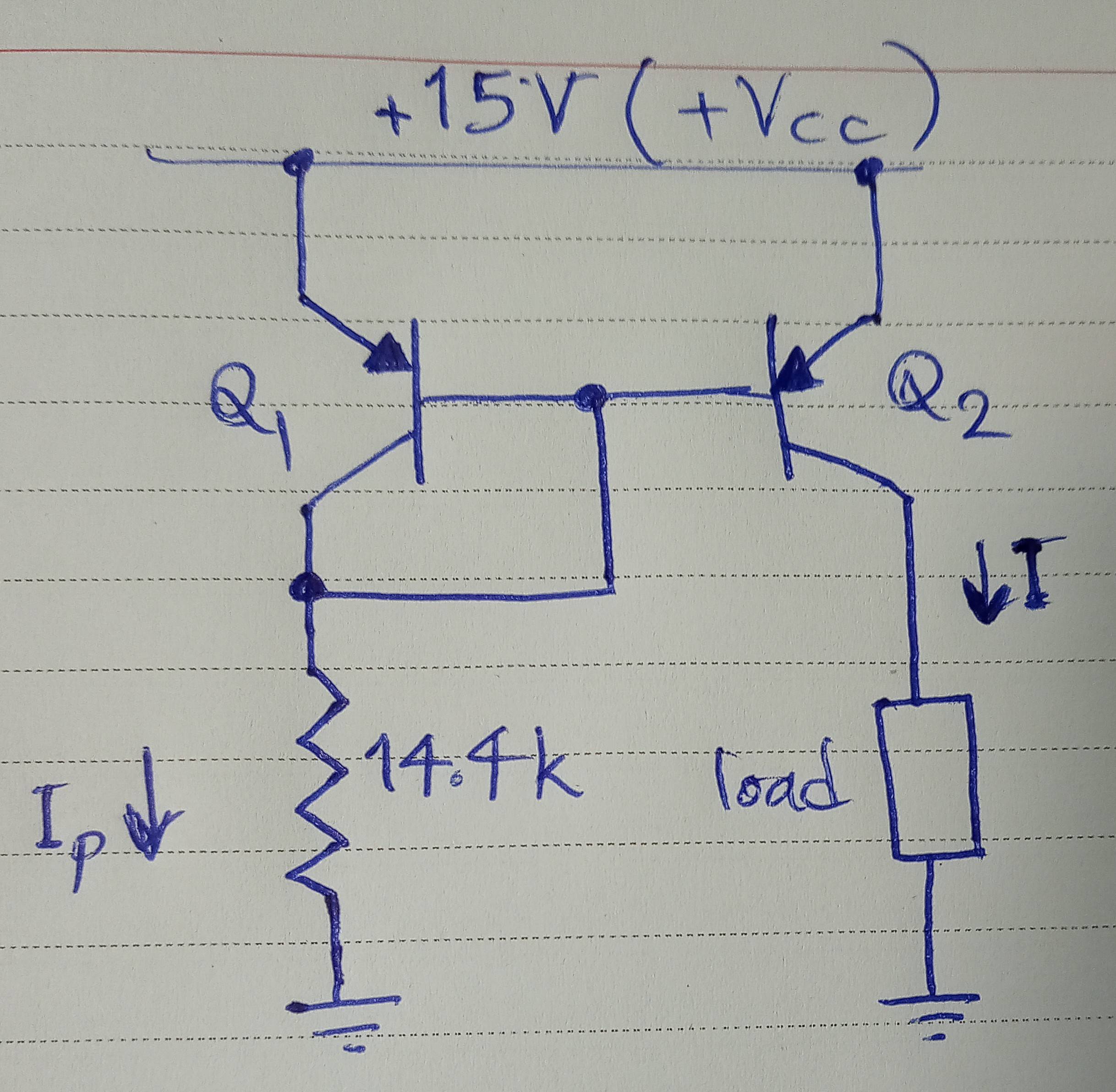
By "simple current mirror" I mean the following circuit.
Due to the Early effect the output current changes to some degree with output voltage. According to The Art of Electronics (3rd edition),
this is because of the slight variation of \$V_\text{BE}\$ with collector voltage at a given current in \$Q_2\$.
The way I understand the sentence above: Change in \$V_\text{CE}\$ at constant \$I_\text{C}\$ leads to change in \$V_\text{BE}\$, which, considering the Ebers-Moll equation, causes a change in \$I_\text{C}\$! By the way, the Ebers-Moll equation, according to the book, is \$I_\text{C}=I_\text{S}[\exp(V_\text{BE}/V_\text{T})-1]\$.
In the book, the Early effect is mathematically formulated for two cases: 1. Changes of \$V_\text{BE}\$ with \$V_\text{CE}\$ at constant \$I_\text{C}\$ 2. Changes of \$I_\text{C}\$ with \$V_\text{CE}\$ at constant \$V_\text{BE}\$. But in this circuit neither \$V_\text{BE}\$ nor \$I_\text{C}\$ is constant.
Another point: The two transistors have the same \$V_\text{BE}\$. So, applying Ebers-Moll equation, they must have the same \$I_\text{C}\$. The book says that output current can change as much as 25% due to change in output voltage. On the other hand, the current in the 14.4k resistor is almost constant (\$(15-0.6)/14.4\$). So about 25% of \$I_\text{C}\$ in Q1 must go to the bases via the wire connecting collector of Q1 to bases, which sounds strange.