Origin of the signal in an oscillator composed of a Schmitt trigger and an integrator

Question

Following instructions, we built this oscillator as part of our electronics course:

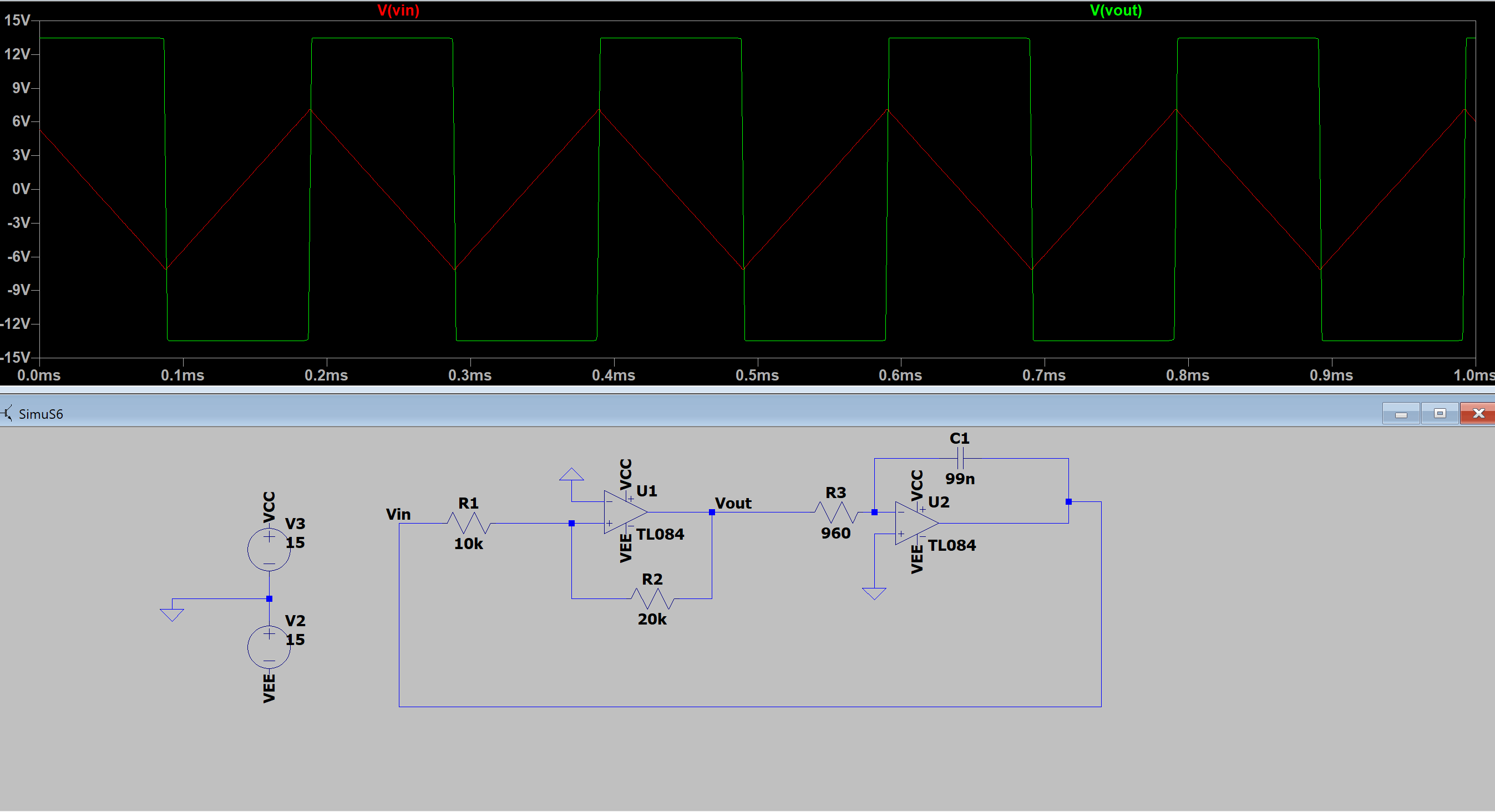
I was surprised at first that we could get those signals without any initial signal connected to the op-amps input. Is it correct that the op-amp (via the positive feedback on the Schmitt trigger) is simply amplifying random noise (always present in the circuit, for various reasons such as ambient magnetic radiation or temperature gradient which results in movement of 'electrons) until it first flips the switch?

EDIT: I'm only talking about the very first moment the signal is created and the very first flip of the switch. I agree that the regular operation of the Schmitt trigger and the integrator take over without any link with this phenomenon afterward.

Answer 1

Is it correct that the op-amp (via the positive feedback on the Schmitt trigger) is simply amplifying random noise (always present in the circuit, for various reasons such as ambient magnetic radiation or temperature gradient which results in movement of 'electrons) until it first flips the switch?

No, that is not entirely correct.

Once the circuit has overcome the (theoretical only) initial start-up problem of an op-amp/comparator with positive feedback, the circuit is implicitly unstable without any of the influences you mention It's a type of relaxation oscillator and this link explains about the theoretical unstable equilibrium problem: -

The system is in unstable equilibrium if both the inputs and outputs of the comparator are at zero volts. The moment any sort of noise, be it thermal or electromagnetic noise brings the output of the comparator above zero (the case of the comparator output going below zero is also possible, and a similar argument to what follows applies), the positive feedback in the comparator results in the output of the comparator saturating at the positive rail.

Answer 2

The random noise together with the unavoidable asymmetries of the internal circuitry of any real opamp play a role only during power-up.

After the power-up transient has ended, the output of the Schmitt trigger will end up (randomly) in either of two states, defined by the two saturation level of its output (Vee or Vcc). From that moment on, the integrator will simply integrate that initial Vout level, producing either a negative or a positive ramp, which is then fed-back to the Schmitt trigger, whose function is simply that of inverting the input of the integrator periodically, so that to produce a periodic signal (triangle wave).

Of course this in turn also produces a square wave as the trigger output (Vout).

So, in the end, random noise has no crucial role in this circuit besides during the startup transient. It's not even essential for that. Even if noise were absent (impossible in real circuit) it would be enough that the asymmetries of the opamp internal circuitry kept the output in saturation during power-up, i.e during that brief period of time when the supplies go from 0 to their nominal level.

But this is only of theoretical relevance, since the two factors (noise + asymmetries) are always present together, so it is not possible in general to ascribe the startup process to either one alone.

Answer 3

Is it correct that the op-amp (via the positive feedback on the Schmitt trigger) is simply amplifying random noise ...

It is not amplifying "random noise", but really "integrating" (through C1) a signal (which is a two-level voltage) at input Vout ...