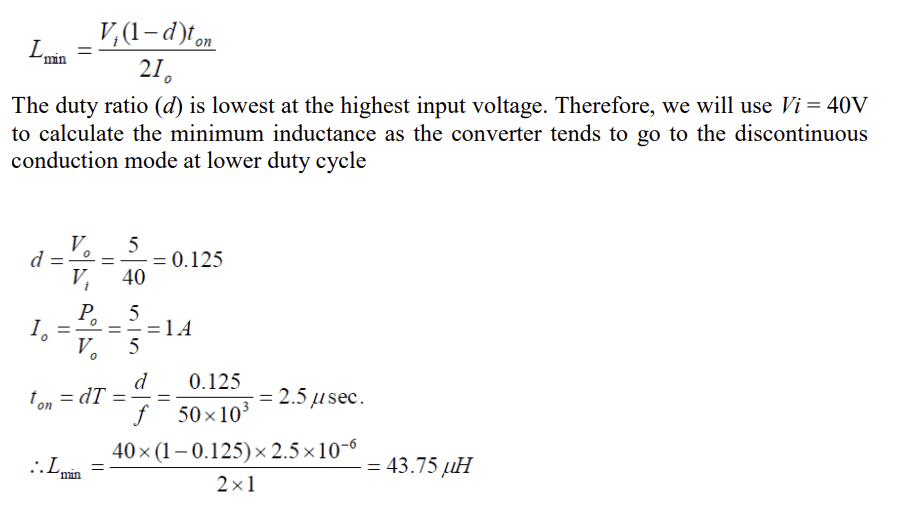In a buck converter operated in continuous conduction mode or CCM, the inductor current ramps up to the peak value \$I_p\$ at the end of the on-time and goes down to the valley current \$I_v\$ when the switching cycle is complete. The difference between the peak and the valley is called the inductor ripple current and noted \$\Delta I_L\$. The inductor average current is the output current \$I_o\$ of the buck converter and, in CCM, it equals the sum of peak and valley currents divided by 2.
When the output current decreases, the inductor peak and valley currents are shifting down and, if the load gets lighter, the valley current reaches 0 A. At this exact point, the converter is at the border between continuous and discontinuous conduction mode (DCM). This operating point is called borderline or boundary conduction mode (BCM) and often represents a wanted operating mode such as in boost power factor correction stages (PFC) for instance. But here, we want to determine the value of the inductance which guarantees CCM for the given operating point. In other words, we must determine the inductance value which brings the valley current to 0 A. By keeping our inductance above the minimum we compute, CCM is always ensured down to the specified output current.
I have gathered the derivation below and it is not very complicated: draw the inductor current and identify the peak and valley currents:
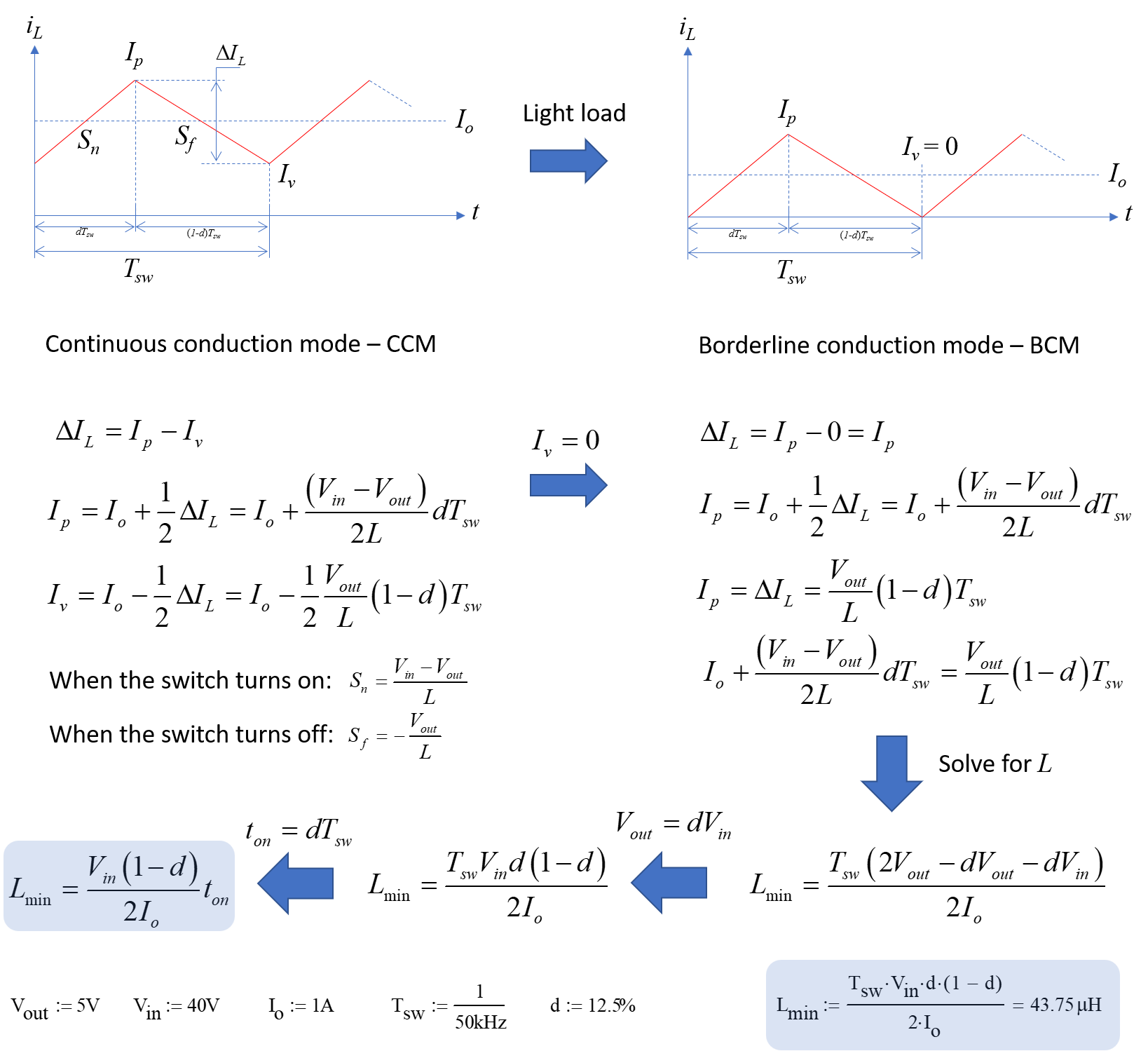
When the load current decreases, you can see in the right side of the picture how the whole inductor current is shifted down until the valley current cancels. Write the corresponding equations and solve for \$L\$ which ensures this result. Rearrange the expressions linking \$V_{out}\$ and \$V_{in}\$ and you are done.