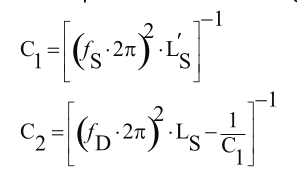How can I solve these two equations to determine resonant capacitors for a wireless charging coil?

Question

My questions:

• What are S, D, and the apostrophe above Inductance?
• Is the equation looking for the inductance of the coil or the circuit?
• Once I know what S and D stand for how can I determine their frequencies?

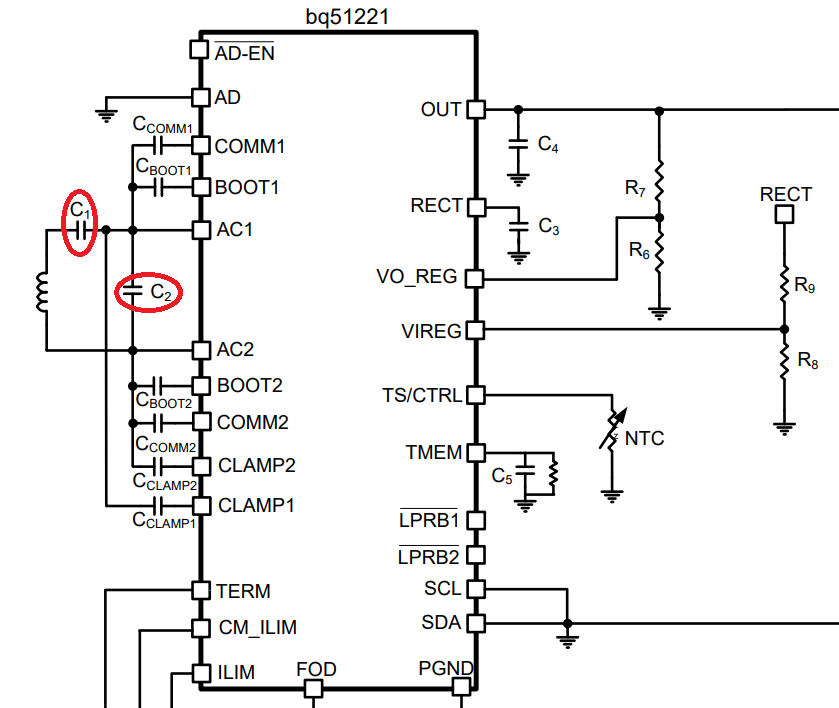
The following is an excerpt from the datasheet for Texas Instruments bq51221 Single Chip Wireless Power Receiver.

10.2.1.2.9 Series and Parallel Resonant Capacitors

Resonant capacitors C₁ and C₂ are set according to WPC specification. Although this is a dual mode solution, the PMA does not specify an exact resonance frequency for the resonant capacitors and in fact does not specify that resonant capacitors are indeed needed.

The equations for calculating the values of the resonant capacitors are shown:

Answer 1

If you look at the main circuit on page 1 of the data sheet they show C1 and C2 in series: -

However, they forgot to indicate what \$L_S\$ is so, it's somewhat of a guess on my part. Firstly however, we have to think of pins AC1 and AC2 as current sources in opposition i.e. one is the inversion of the other.

This then permits us to be able to say that C1 and C2 are truly in series and, excitation from the AC pins to C2 is in the form of a current and, that current doesn't screw around with the impedances and formulas.

The resonant frequency of a parallel inductor and capacitor is this: -

$$F_R = \dfrac{1}{2\pi\sqrt{LC}}$$

And, it appears that C1 is the dominant reactance i.e. the highest reactance compared to C2 and, I suspect that this means you can rearrange the above formula to find C1: -

$$C_1 = \left(\dfrac{1}{2\pi F_R\sqrt{L}}\right)^2$$

Hopefully you can see that it is equivalent to your first formula (apart from the inductor being called \$L'_S\$). I think they are calling it \$L'_S\$ to fudge the math to make it work. So, I would call \$L'_S\$ the equivalent inductance that makes the circuit resonate at the correct frequency if we ignored C2.

Again, it seems an incompetent way of doing it but, TI no doubt have their reasons.

Then, for the 2nd formula TI are doing the right thing and solving my first formula where C is replaced with: -

$$C = \dfrac{1}{\frac{1}{C_1}+\frac{1}{C_2}}$$