Transformer saturation value in volt-seconds

Question

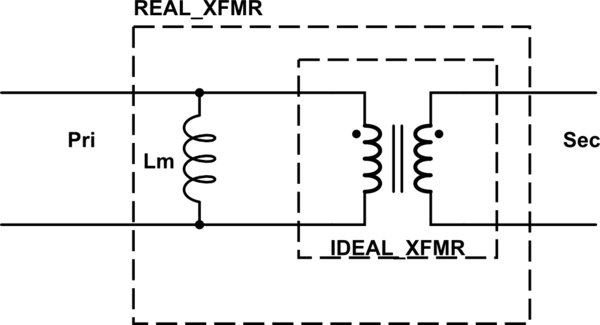
I have a slight confusion regarding the saturation rating of transformers. I often find this value stated in Volt-Seconds, which is a unit for the saturation flux, i.e. the maximum flux that the core can hold. After all, the saturation flux density \$B_\text{sat}\$ and the cross section of the core \$A\$ are both independent of its windings. So their product \$\Phi_\text{sat}=B_\text{sat}\cdot A\$, which happens to have units of Volt-Seconds, has to be independent of the windings, too.

Therefore, I regarded the saturation Volt-Second rating to be a property of the transformer core.

But then I thought about what happens in a 2:1 transformer when I apply the same Vs to one or the other winding (with no load on the second winding):

• The inductance of the shorter winding is 1/4th of the inductance of the longer winding
• So the current through the shorter winding will rise 4x as fast
• So for the same Vs applied to each winding, the shorter winding will have developed twice as many Ampere-Turns
• So the shorter winding would have generated twice the flux for the same Vs (and would thus saturate at a lower Volt-Second rating)

Now I was confused, because the latter consideration would indicate that the Volt-Second saturation does in fact depend on the winding properties and is not a property of the core.

Where did I go wrong?

Answer 1

The transformer/inductor core saturation parameter is not a Volt.Second product, though it is frequently pronounced like that, perhaps to save syllables, assuming a single turn. It is a Volts_Per_Turn.Second product.

If you have a winding with twice as many turns, you can apply twice as many volts.

Consider an inductor wound with two separate identical windings. The core flux does not care whether you connect these windings in parallel, to get V at twice the I, or in series, to get twice the V at only one I.

If you have some windings on a core, you can easily scale the core Vs/turn property by the actual number of turns on a particular winding, to give you a Vs product specific to that winding.

Answer 2

Now I was confused, because the latter consideration would indicate that the Volt-Second saturation does in fact depend on the winding properties and is not a property of the core.

Core permeability, current and number of turns dictate the saturation point. For convenience that can be equated to volt.seconds but, it will be a different value for a different number of turns.

We probably all know this: -

$$V = N\dfrac{d\Phi}{dt}$$

So, if we rearrange we get this: -

$$V\cdot dt = N\cdot d\Phi$$

Or, volt.seconds yields flux multiplied by the number of turns.

Answer 3

Correct, you need to know the winding reference. Usually it's labeled "primary", but datasheets are often vague about this, and one may wish to ask the manufacturer.

There's also the matter of whether it's peak, peak-to-peak, sine wave, RMS, or what flux. Peak should be the most common, but you never know unless specified.

Regarding the confusion between cores and windings, consider that there are two fluxes in play here: the free field flux \$B A_e\$, and the electrical or in-circuit flux \$B A_e N\$. When I present calculations on these, I include a fake unit of "turns" (t) (usually arising from \$\mu_0\$ or \$A_e\$), to make it explicit that the field flux is per-turn, while the electrical flux is at given turns. (Inserted on the proper constant, we automatically get \$A_L\$ in units of H/t², for example. Oh hm, it's both then isn't it? :) )

Alternately, one might carefully label the variables, like using capital \$\Phi\$ for field flux, and lowercase \$\phi = \Phi N\$ for circuit flux.

Answer 4

I often find this value stated in Volt-Seconds

Volt-seconds i.e. flux, along with core properties including saturation flux density, becomes a transformer parameter. Volt-seconds also equals to Ampere-Henries.

$$ V_L \ \Delta t = L \ \Delta i $$

So, for an inductor (can also be a transformer's one of the windings) with an inductance of L with a number of turns of N, by applying either of the following, you shouldn't exceed the Volt-seconds product which corresponds to, as you said, \$\Phi_{sat} = B_{sat} \ A_e\$:

• a voltage of V across the terminals, for a time duration of Δt, or
• a current change of Δi

But then I thought about what happens in a 2:1 transformer when I apply the same Vs to one or the other winding

We can model a transformer like this:

^{simulate this circuit – Schematic created using CircuitLab}

^{I'll not explain what is what, as you already know.}

The above model shows that only the primary winding has a "magnetising" inductance which is a result of an assumption like "This winding will magnetise the core" (The rest is an ideal transformer which has infinite magnetising inductance and therefore isn't mentioned). We often don't think about magnetisation using any other windings. As a result, when we design a transformer, after determining the turns ratio, we calculate the number of turns of the "primary" or "magnetising winding" using Faraday's equation:

$$ V_t = N \ A_e \ \frac{dB}{dt} $$

So we define the Volt-seconds for primary (or whichever winding is assumed to be magnetising the core). So if you apply the same Volt-seconds to another winding, specifically to one having a lower number of turns, the flux swing will be higher and maybe closer to (or even beyond) the saturation level.

As a result, if we define Volt-seconds for a transformer, we should also define its "primary winding". Or in other words, Volt-seconds per turn. We don't often use the latter because we know that one specific winding will be used as a primary i.e. the winding that magnetises the core.

Also, as I mentioned above as Ampere-Henries, if you apply the same Volt-seconds to a lower inductance (which is a result of a lower number of turns) you'll cause a larger current change which in turn will cause a higher H-field change. Because of the relation between B and H, the B swing will be higher and closer to the saturation point.