RLC circuit with a step DC source connected to it

Question

Consider an RLC circuit. I have found much questions related to RLC's asked before, but I think I need to educate myself a bit more. I have the general idea about critical damping, over-damping and under-damping. However, the issue is, why doesn't my output in an oscilloscope match with the expected one from the equation for the case of underdamping.

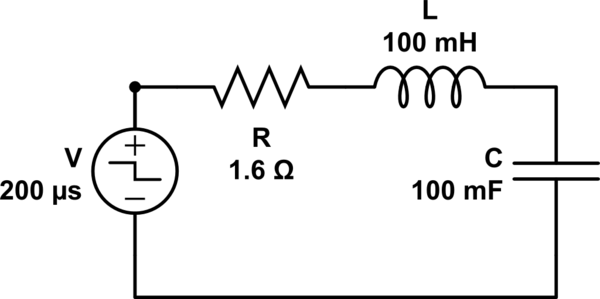
^{simulate this circuit – Schematic created using CircuitLab}

Here, a step voltage \$V_s=0.5V\$ has been applied in the circuit. Here, we have: $$\alpha=\frac{R}{2L}=8$$ $$\omega_0=\frac{1}{\sqrt{CL}}=10$$ excluding the units. Therefore, the damping frequency is:

$$\omega_d^2=\sqrt{\alpha^2-\omega_0^2}=-36$$ The series circuit about has \$\alpha<\omega_0\$ which means it is underdamped. For such a system the voltage across the capacitor is governed by the equation: $$v_c(t)=V_s+(A_1\cos{\omega_d t}+A_2\sin{\omega_d t})e^{-\alpha t}$$ To solve the equation, I have considered two initial conditions. The first is that, capacitor voltage must be 0 at \$t=0^+\$ since it cannot abruptly change its voltage. Second, the rate of change of voltage just at that time is \$0\$, since inductor does not allow change of current just at \$t=0^+\$. Therefore, I have in my hand: $$v_t(0^+)=0$$ and $$\frac{di(0^+)}{dt}=C \frac{dv_c(0^+)}{dt}=0$$ Plugging this two conditions into the equation, I found out \$A_1=-0.5\$ and \$A_2=-\frac{2}{3}\$. The equation, so becomes: $$v_c=\frac{1}{2}+\left(-\frac{1}{2}\cos(6t)-\frac{2}{3}\sin(6t)\right)e^{-8t}$$ Everything goes well up to this. As I plug this equation in desmos, what I get is something like this:

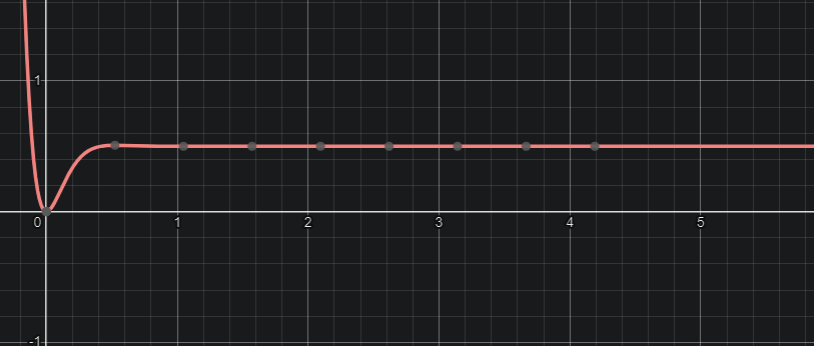
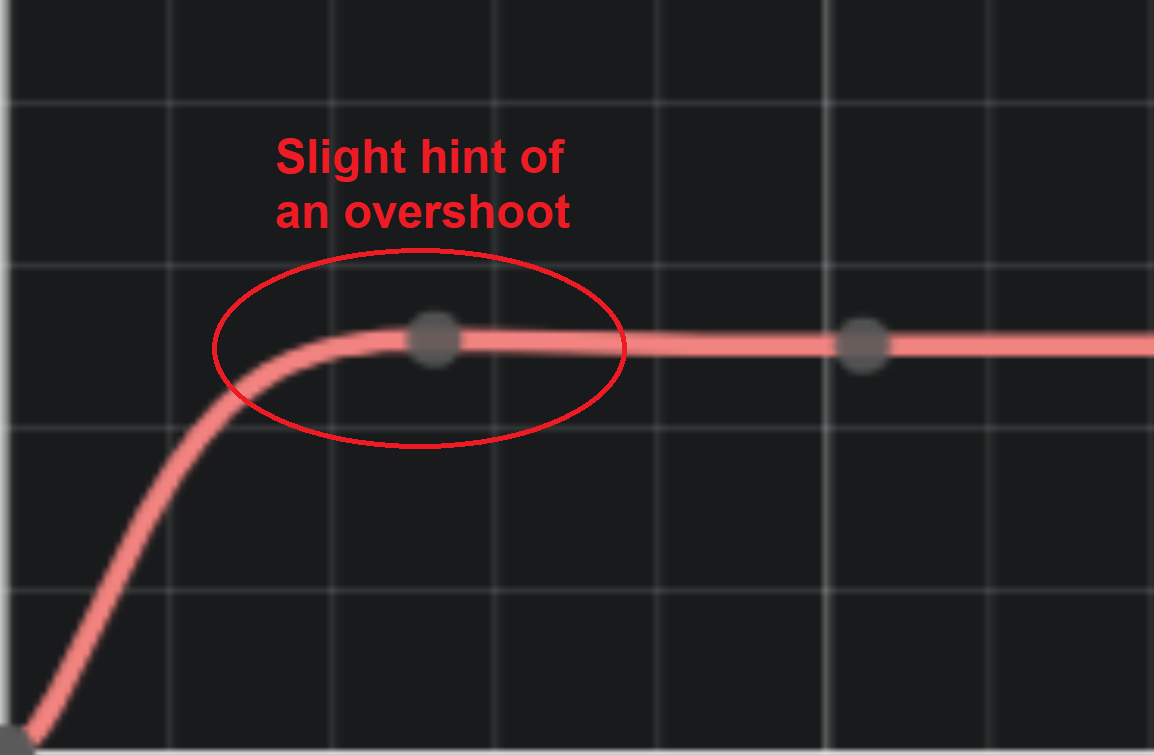
However, this shall not be the case. An underdamped circuit usually have the response:

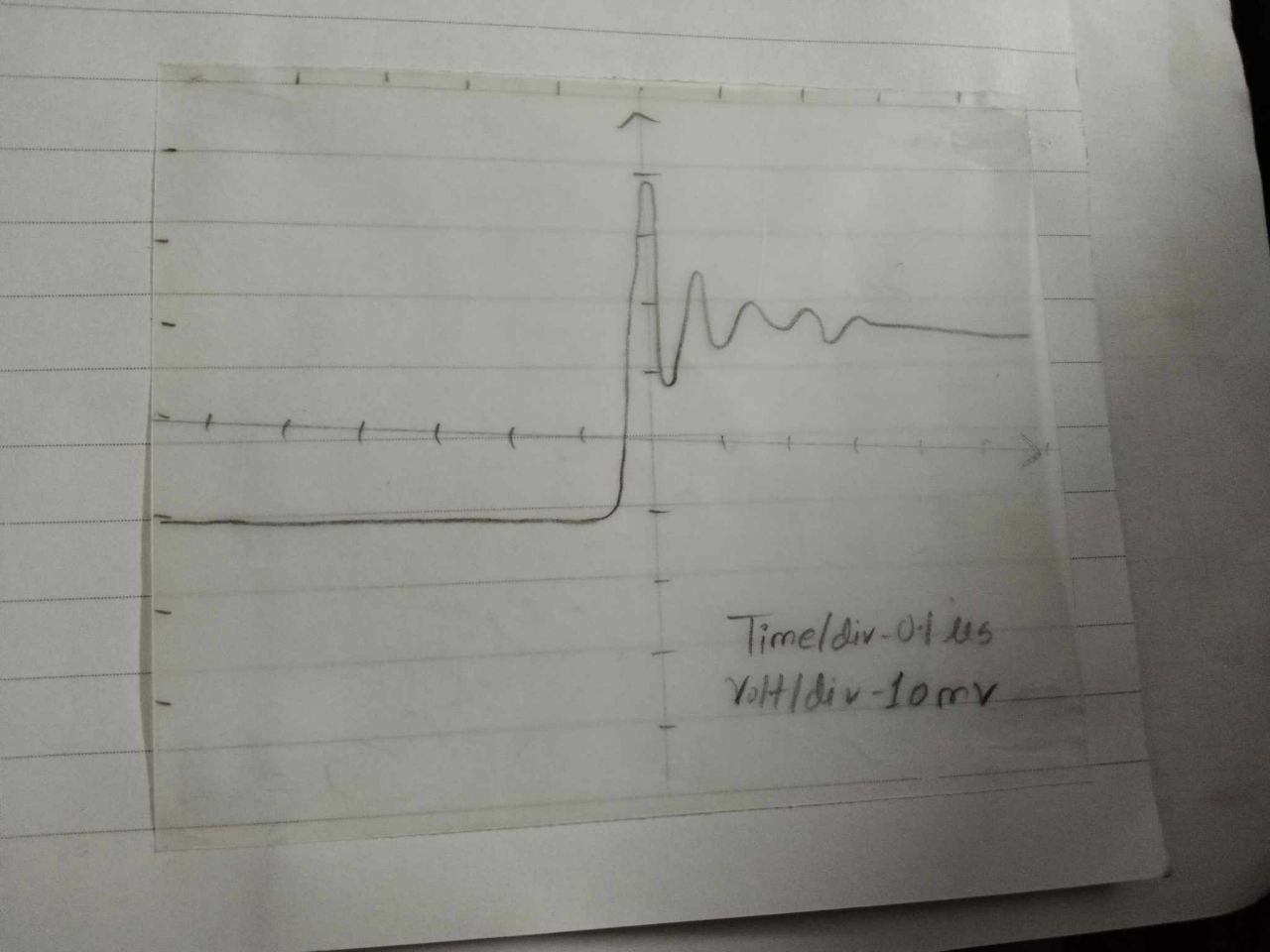
Now, there are few issues that need to be solved. First, what's incorrect in my equation. I presume that some of my initial conditions have gone wrong. Second, if the oscilloscope is correct [and I surely know it is], then what line am I suppose to consider as \$t=0\$ line? I need to properly place the graph in the tracing paper, so that my data, extracted from here, is accurate.

Answer 1

The series circuit about has α <\$ω_0\$ which means it is underdamped.

It is underdamped (\$\zeta = 0.8\$) but significantly, you thought that its response will be far more underdamped than what it is. Here is a view of my RLC low-pass calculator with your values plugged-in: -

There are small signs of overshoot (and ringing) but the major overshoot peak is only 1.516 % higher than the input step amplitude. I expect that if you analysed your scope shot more carefully, you will see the same: -

You also made this rookie error: -

Therefore, the damping frequency is: \$\omega_d^2=\sqrt{\alpha^2-\omega_0^2}=-36\$

The error you made was to believe that a negative sign inside a square root can be transferred to the outside of the square root as a negative sign. The square toot of -1 is actually \$j\$ hence, your damped resonant frequency is \$j6\$.