Calculating inductance from energy

Question

^{simulate this circuit – Schematic created using CircuitLab}

I have voltage source and an inductor with internal resistance in a circuit and I have measured the voltage and current across the inductor when switched on. Now I want to calculate the inductance or at very least a time constant.

I have managed to come to this equation but was not able to solve for the time constant. $$\small E=\frac{1}{2}Li^2=\frac{1}{2}LI^2(1-e^{-t/\tau})^2=\frac{1}{2}LI^2(1-2e^{-t/\tau}+e^{-2t/\tau})=\frac{1}{2}\tau RI^2(1-2e^{-t/\tau}+e^{-2t/\tau})$$

I am a bit out of practice with these equations so any help is appreciated.

Answer 1

To find the value of the inductance in this first-order circuit you will need to look at the transient waveforms, not just the steady-state response to a DC voltage. To find the value of the inductance the analysis is extremely simple in sinusoidal steady-state (frequency domain), but since it seems that you are attempting a time-domain solution I will present that here. I will assume that the voltage source is turned on at time t=0. Writing a KVL results in $$iR+L\frac{di}{dt} - V_s=0, ~~~~~~i(0)=0$$ The solution to this differential equation for the current is $$ i(t) = \frac{1}{R}(1-e^{-\frac{R}{L}t})u(t) $$ You can monitor this current waveform (by monitoring the voltage across the resistor) as you switch the voltage on, measure the time constant, and infer the inductance, assuming you know the resistance.

Answer 2

I ran your equation through an old Maple solver and got this for the time constant T. There are two solutions.

It would be best to post the schematic of your circuit.

Answer 3

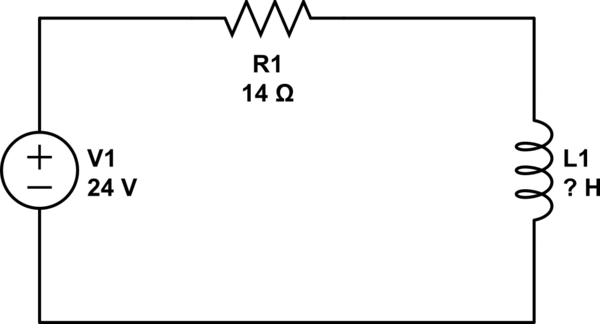
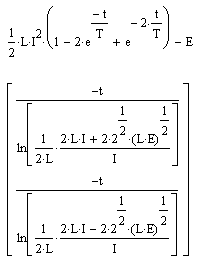
Notice, notice that the energy is given by:

$$\text{E}\left(t\right)=\int\text{P}\left(t\right)\space\text{d}t=\int\text{V}\left(t\right)\cdot\text{I}\left(t\right)\space\text{d}t\tag1$$

The voltage-current relation in an inductor is given by:

$$\text{V}_\text{L}\left(t\right)=\text{I}_\text{L}'\left(t\right)\cdot\text{L}\tag2$$

So, we get:

$$\text{E}_\text{L}\left(t\right)=\int\text{I}_\text{L}'\left(t\right)\cdot\text{L}\cdot\text{I}_\text{L}\left(t\right)\space\text{d}t=\text{L}\int\text{I}_\text{L}'\left(t\right)\cdot\text{I}_\text{L}\left(t\right)\space\text{d}t=\text{L}\cdot\frac{\text{I}_\text{L}^2\left(t\right)}{2}+\text{k}\tag3$$

So, assuming that \$\displaystyle\text{E}_\text{L}\left(0\right)=\text{I}_\text{L}\left(0\right)=0\$, we get \$\displaystyle\text{k}=0\$.

So, back to your circuit. We can see that:

$$\text{I}_\text{L}\left(t\right)=\mathscr{L}_\text{s}^{-1}\left[\frac{\displaystyle\frac{\displaystyle\hat{\text{u}}_\text{i}}{\displaystyle\text{s}}}{\text{R}+\text{sL}}\right]_{\left(t\right)}=\frac{\displaystyle\hat{\text{u}}_\text{i}}{\displaystyle\text{R}}\cdot\left(1-\exp\left(-\frac{\displaystyle\text{R}t}{\displaystyle\text{L}}\right)\right)\tag4$$

So, we get:

$$ \begin{alignat*}{1} \text{E}_\text{L}\left(t\right)&=\text{L}\cdot\frac{1}{2}\cdot\left(\frac{\displaystyle\hat{\text{u}}_\text{i}}{\displaystyle\text{R}}\cdot\left(1-\exp\left(-\frac{\displaystyle\text{R}t}{\displaystyle\text{L}}\right)\right)\right)^2\\ \\ &=\frac{\displaystyle\text{L}\hat{\text{u}}_\text{i}^2}{\displaystyle2\text{R}^2}\cdot\left(1-\exp\left(-\frac{\displaystyle\text{R}t}{\displaystyle\text{L}}\right)\right)^2\\ \\ &=\frac{\displaystyle\text{L}\hat{\text{u}}_\text{i}^2}{\displaystyle2\text{R}^2}\cdot\left(1+\exp\left(-\frac{\displaystyle2\text{R}t}{\displaystyle\text{L}}\right)-2\exp\left(-\frac{\displaystyle\text{R}t}{\displaystyle\text{L}}\right)\right) \end{alignat*} \tag5 $$

Now, we can notice that when \$\displaystyle t\to\infty\$ we get:

$$\lim_{t\space\to\space\infty}\text{E}_\text{L}\left(t\right)=\frac{\displaystyle\text{L}\hat{\text{u}}_\text{i}^2}{\displaystyle2\text{R}^2}\cdot\left(1+\underbrace{\exp\left(-\frac{\displaystyle2\text{R}t}{\displaystyle\text{L}}\right)}_{\to\space0}-2\underbrace{\exp\left(-\frac{\displaystyle\text{R}t}{\displaystyle\text{L}}\right)}_{\to\space0}\right)=\frac{\displaystyle\text{L}\hat{\text{u}}_\text{i}^2}{\displaystyle2\text{R}^2}\tag6$$