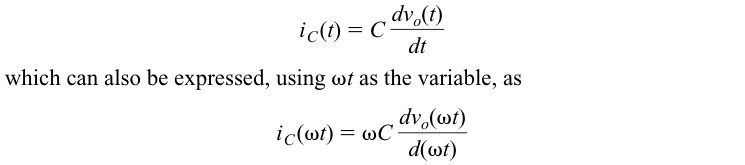Is \$i(t)=i(\omega t)\$ always valid in power electronics?

Question

This might be an easy question but it really confused me when reading Hart's Power Electronics, especially while dealing with differentials. For example, the following image is from the book's page 90:

If I am not mistaken the last equation in the above image is valid only if we define $$i_C(\omega t)=C\cdot\frac{dv_o(\omega t)}{dt}$$ This means that we substitute \$\omega t\$ only at \$v_o(t)\$ and not at \$dt\$. This is my first point.

Second, if \$v_o(t)\$ is something like \$\sin(\omega t)\$, then, mathematically, \$i_C(\omega t)\$ will have a frequency equal to the square of the frequency of \$v_o\$ since \$v_o'(t)=\omega\cdot \cos(\omega t)\$ and \$v_o'(\omega t)=\omega\cdot\cos(\omega^2 t)\$. Here, I think that substitution is not a change of variable in a sense that changes the function like compressing it but just a change of perspective. I might not be able to explain myself here so I have a second image from the book (page 69) that clarifies what I mean.

Regarding my first point, why do we only substitute \$dv_o\$? Is it because \$v_o(\omega t) = v_o(t)\$?

Is \$i_C(\omega t)=i_C(t)\$ and is this always the case?

Sorry if the question is a bit vague but it is also such for me. Thank you in advance.

Answer 1

I believe your question has nothing to do with power electronics but only to do with how those terms are expressed.

In your first image, the capacitor current \${i_C}\$ is simply represented as a function of \$t\$ in the upper equation, and as a function of \$\omega t\$ in the lower equation. A function \$f(\omega t)\$ is a function of \$\omega t\$, but it is also a function of \$t\$, \$f(t)\$ (if \$\omega\$ is constant w.r.t. \$t\$).

For a function \$f(\omega t)\$, using the chain rule

\$\frac{d}{dt}f(\omega t) = \omega\frac{d}{d(\omega t)}f(\omega t)\$

Consider your example where \$v_0(t) = sin(\omega t)\$. It can also be written as \$v_0(\omega t) = sin(\omega t)\$, and

\$\frac{d}{dt}v_o(\omega t) = \omega cos(\omega t)\$

but

\$\frac{d}{d(\omega t)}v_0(\omega t) = cos(\omega t)\$

---

In the second image, in eq. (3-12), \$i(\omega t)\$ is a function of \$\omega t\$ but it is also a function of \$t\$, just like eq. (3-11). They are only calling the term \$\omega t\$ as a different variable (say \$\theta\$, representing angle), and creating that equation. So, eq. (3-12) can be written as \$i(\theta)\$, with both the \$\omega t\$ terms replaced with \$\theta\$.