The relation between light electric field and light power (optical modulation)

Question

I have been keeping thinking this question for one week. Hope could get some help from you.

• Light 1:

  Electric field = \$\text{e}^{j\omega t}\$;

  Power = \$\frac{1}{2}A^2\$

• light 2: \$ \alpha = \omega_h \cdot t\$

  Electric field = \$A \text{e}^{j\omega t}\cos(\alpha)\$;

  power = \$\frac{1}{2}A^2 \cdot \dfrac{1+\cos(2\alpha)}{2}\$

• light 3:

  \$ \alpha = \omega_h \cdot t\$

  power= \$\frac{1}{2}A^2 \cdot \dfrac{1+b\cos(2\alpha)}{2}\$ , 0 < b < 1.

  electric field??

• why do I want to do this?

  Electric field = \$ \frac{1}{2}A^2\text{e}^{j\omega_c t}(\frac{1}{2}(\text{e}^{j\omega_h t} +\text{e}^{-j\omega_h t} )) = \frac{1}{4}A^2(\text{e}^{j\omega_c t + j\omega_h t} + \text{e}^{j\omega_c t -j\omega_h t})\$

  The the frequency components of the light can be observed from \$ \omega_c - \omega_h\$ and \$ \omega_c + \omega_h\$

My question is, what is the electric field of light 3? Is it possible to write down a mathematical formula for this? If not, how to simulate it in Matlab?

Answer 1

So, in general case, the electric field should be kind of: $$E_3=A\sqrt{\frac{1+b\cos(2\omega_h t)}{2}}\text{e}^{j\omega_c t}$$ You may worry about the sign '\$\pm\$'before the square root. That would be a very good consideration and we need to extend our solution into a generic expression. We may notice the amplitude factor could be extended as: $$\pm\sqrt{\frac{1+b\cos(2\omega_h t)}{2}}\\ =\pm\sqrt{\frac{1+b[2\cos^2(\omega_h t)-1]}{2}}\\ =\cos(\omega_h t)\cdot\sqrt{b+\frac{1-b}{2\cos^2(\omega_h t)}} $$ It is easy to verify that for \$b=1\$ the above expression is just \$\cos(\omega_ht)\$ as we desired. So we can rewrite \$E_3\$ as: $$ E_3=\frac{A\cdot\beta(t)}{2}\cdot\bigg[\text{e}^{j(\omega_c-\omega_h)t}+\text{e}^{j(\omega_c+\omega_h)t}\bigg]\;, $$ where \$\beta(t)=\sqrt{b+\frac{1-b}{2\cos^2(\omega_h t)}}\$. Maybe...Maybe... You also worry about the singularities of \$\beta(t)\$ $$\cos(\omega_ht)\rightarrow0\quad\Rightarrow\quad\beta(t)\rightarrow\infty\;.$$ To cure this problem, I introduce a prescriptive parameter \$\epsilon\$, it could be an arbitrary small positive number (like 0.01,0.001) in the denominator for avoiding the divergence. $$ \beta(t)\rightarrow\beta'(t)=\sqrt{b+\frac{1-b}{2\cos^2(\omega_h t)+\epsilon}} $$ My next step is to find the Fourier spectrum of '\$\cos(\omega_h t)\sqrt{b+\frac{1-b}{2\cos^2(\omega_h t)}}\$' between \$ -\omega_h\$ to \$\omega_h\$--because we already have the phase '\$\text{e}^{j\omega_c t}\$'in our expression. This will be useful for getting monochrome modes.

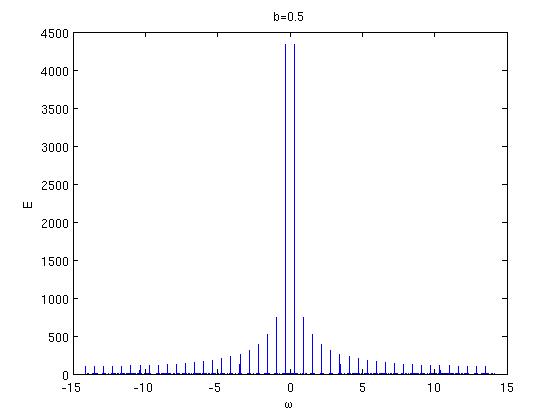
Here are the pictures I made

for b=1

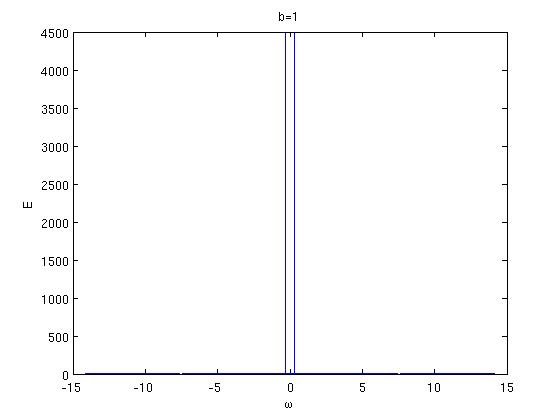
for b=0.5

$$-------------------------------------$$ Here is the code for Matlab

Th=20; %% period of omega_h
omegah=2*pi/Th;%%% we define \omegah via defining Th
N=90; %% divide the time interval (one period) into N equal parts.
L=100; %% Length of time interval interms of numbers of periods.
tt=Th/N*[1:L*N]; %% we generate a time vector including 3 periods.
b=0.5;
amph=cos(omegah*tt).*sqrt(b+(1-b)*power((2*cos(omegah*tt).*cos(omegah*tt)),-1)); %% this is our factor in the amplitude.
plot(tt,amph) %%% the picture of the amplitude including 'L' periods.
ttrial=Th/N*[1:3*N];
amphtrial=cos(omegah*ttrial).*sqrt(b+(1-b)*power((2*cos(omegah*ttrial).*cos(omegah*ttrial)),-1));
plot(ttrial,amphtrial) %%% the picture of the amplitude including '3' periods, this picture just helps you tell the pattern of the mode easily.
%%
FThh=fft(amph);
Homg=fftshift(FThh); %%% if you were not familiar of usage of commands 'fft' and 'fftshift' check them in the matlab help, they are extremely useful!!!
angleomg=-pi*N/Th:2*pi/(L*Th):pi*N/Th-2*pi/(L*Th);
plot(angleomg,abs(Homg))
title('b=0.5')
xlabel('\omega')
ylabel('E')