Initial Conditions and current for an RLC circuit

Question

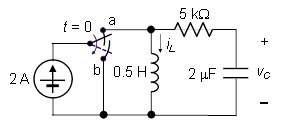
It is required to find \$i_L\$ and \$v_c\$. It is quite easy to find \$v_c\$ from \$i_L\$ using simple integration. So, for now finding \$i_L\$ will be the concern of the question. Prior to closing the switch or at \$0^{-}\$, the current in \$i_L\$ is 2A because it is a short circuit and the capacitor is a open circuit assuming that its been in this state for a while. My concern is at \$0^{+}\$ what is the voltage across the inductor-\$\frac{di(0^{+})}{dt}=\frac{v_L}{L}\$. So, once the switch is closed the short circuit and the current source can be ignored. What is the voltage across the inductor? Its one of two: either \$0 V \ \text{or} \ 10 V\$

I am finding the voltage across the inductor in order to find the initial conditions later on. There is another way to find the other initial condition using the voltage across the capacitor at \$t=0^{+}\$ but I only use that to evaluate my other method. Following the capicitor method, the voltage 0V across the inductor provides the same answer. This leads me to believe that the voltage across the inductor is in fact zero.

Can someone please explain to me what the voltage across the inductor is at \$t=0^{+}\$? Why it is or isn't zero?

Answer 1

The current through an inductor is continuous so

$$i_L(0+) = 2A$$

The voltage across a capacitor is continuous so

$$v_C(0+) = 0V$$

Now, the inductor and the resistor are in series so they have identical currents. So, armed with just KVL and Ohm's Law,

$$v_L(0+)=?$$

Answer 2

To actually answer the question: It's neither 0 nor 10 V.

As Alfred hinted, it's the sum of the voltage over the capacitor (which is zero) and over the resistor.

Now, as the inductor's current \$i_L(0-) = I_L(0+) = 2 A\$, the voltage over the resistor, and therefore over the inductor \$ u_L(0+) = 5 k\Omega * 2 A = 10 kV\$.