0
\$\begingroup\$

I am reading "Electronic Devices and Circuit Theory" by Louis Nashelsky, Robert L. Boylestad.

I am not able to grasp article 3.5: Transistor Amplifying Action. I've mention some particular points on which I am having questions. In general I want to understand this article. I want to understand what the authors are saying here.

At the last of the article the authors say that "because the source current is transferred from a low- to a high-resistance circuit, that's why we call this device as transfer+resister \$\rightarrow\$transister." But I don't understand the way they reached this statement. I've bought this book and I don't want to waste my money; I do not want to put this book aside and buy some other book. Please help me understand it.

The circuit schematic is as shown:

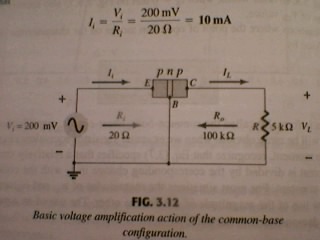
image

  • Input resistance \$R_i= 20Ω\$,
  • Output resistance \$R_o = 100KΩ\$.
  • Input voltage \$V_i = 200mV\$
  • The emitter current \$I_i= V_i/R_i = 10mA\$

Since \$α_{ac}≈1\$, so \$I_c≈I_e\$

Output voltage \$V_L=I_LR=(10mA)(5KΩ)=50V\$.

Voltage amplification is \$A_v=V_L/V_i=50V/200mV=250\$.

What I don't understand is:

  1. What is \$200mV\$, is it the instantaneous voltage, rms voltage or peak voltage? What are \$I_L ,\ I_i,\ R_o\$ and \$R_i\$ etc's?

  2. Why the input resistance doesn't get affected by the load resistance. In fact, what determines the input resistance? Are the \$n\$ region and load resistance acting as a set of two parallel resisters for \$V_{in}\$?

  3. Since there is no DC biasing i.e \$Vcc\$ is \$0\$, how is this high output voltage supplied?

  4. How can we apply KVL to the loop BCB? The power dissipated across \$R\$ is \$I_LV_L=0.5W\$. What is the source of this high power, is it the minute reverse bias collector junction (which is quite impossible because a P-N junction cannot provide energy)?

  5. This circuit acts like a current source. \$I_L\$ is completely independent of the load resistance. How can we use this (common base) circuit as an amplifier for low resistance load? Or suppose we want to apply the \$V_L\$ to some resistor of order 10 ohms, the output will reduce to \$10 \Omega \times 10mA = 100mV \$ so the output voltage is reduced than the input voltage(200mV).

    If the biasings are suppressed please explain this circuit. That is with proper biasing at the same parameters and how much \$V_{EE}\$ and \$V_{CC}\$ should have been applied? what are the actual input and output waveforms?

\$\endgroup\$
2
  • 1
    \$\begingroup\$ Please tell me the reason for the downvote so i could improve my post. \$\endgroup\$
    – user31782
    Commented Mar 1, 2014 at 10:50
  • \$\begingroup\$ I'm not sure which edition of the book is used above (there are 11 editions of this textbook, for real!), but in the 7th edition that Google Books lets me peek in, they cleary say that DC circuit/bias is omitted for that analysis. \$\endgroup\$
    – got trolled too much this week
    Commented Jan 18, 2015 at 5:34

2 Answers 2

Reset to default
3
\$\begingroup\$

This is an AC analysis of signals and all the clutter of DC components is removed - input and output capacitors are replaced with short circuits and power supplies are also replaced with short circuits. It's not a DC analysis or any other analysis - it's an analysis of signal amplification and AC impedances with a minimalist approach diagramatically. This method is meant to help beginners but I've never thought it was helpful - show me the full circuit any day and I'll figure it out from that has always been my approach!

And for your point 3 it is assumed that base current is zero in a lot of AC analyses.

Point 4 - this circuit can be used with the collector effectively seeing a load resistance of under 100 ohms. Try researching an emitter fed cascode amplifier - two transistors are used in common base and the collector of the 1st transistor feeds the emitter of the 2nd transistor. Most cascode amplifier circuits feed the base of the first transistor from the external input but it can work with both transistors in common-base configuration.

The input resistance is determined by the forward conduction dynamic resistance of the emitter-base junction - it's forward biased and has a dynamic (AC) impedance of 20 ohms in your question - this is presumed to be the slope of the emitter-base diode. Load resistance will not affect this.

\$\endgroup\$
6
  • \$\begingroup\$ If the input resistance is dynamic then how this book wrote:\$I_i= V_i/R_i = 10mA\$ dynamic resistance is the derivative so we should have \$R_i=\dfrac{dV_i}{dI_i}\$. Moreover \$\alpha_{ac}\$ is taken as it would be \$\alpha_{dc}\$. It is also not mentioned what \$I_i\$ and \$I_L\$ represents are they instantaneous current or rms? Also i didnt got explainaition of 3rd point. \$\endgroup\$
    – user31782
    Commented Feb 22, 2014 at 13:27
  • \$\begingroup\$ @Anupam In AC analyses it is assumed that the input AC voltage is small and sits at a particular point on the VI curve of the BE diode - I take the 20 ohms to mean that that resistance can be assumed constant for small signals. For a different biasing arrangement it could be 30 ohms or 40 ohms or less but I can't tell from the info you have given. \$\endgroup\$
    – Andy aka
    Commented Feb 22, 2014 at 13:44
  • \$\begingroup\$ The book doesn't mention whether the input resistance \$R_i\$ is static or dynamic. It appears from the context that it is static because they've written \$V_i=I_iR_i\$. \$\endgroup\$
    – user31782
    Commented Feb 22, 2014 at 13:59
  • 1
    \$\begingroup\$ @Anupam, I recommend that you look for a page in the book that lists the notation convention for circuit variables. The case of the variable and subscript are important. The fact that the subscript is lower case, leads me to suspect that these quantities are small-signal or AC quantities. For example, see: www-inst.eecs.berkeley.edu/~ee40/fa03/lecture/lecture24.pdf \$\endgroup\$
    – Alfred Centauri
    Commented Feb 22, 2014 at 14:20
  • \$\begingroup\$ @AlfredCentauri There is no page which lists the notation convention for circuit variables. In the link you given it says "upper-case letter with upper-case subscript" is DC. Does It mean in the context of my book \$I_L\$ refers to DC output current? And what about \$V_{i}\$ and \$I_{i}\$ there are uppercase letter with lower case subscript? \$\endgroup\$
    – user31782
    Commented Feb 22, 2014 at 14:47
0
\$\begingroup\$

Seeing this diagram out of context, it's hard to say, but frequently in AC circuit analysis, the DC sources are omitted from diagrams in order to make the analysis simpler (superposition principle).

You just have to take it for granted that there's a DC source in series with the AC source shown on the emitter side so that the actual instantaneous current is always positive, and that there is another source in series with the load so that the instantaneous collector current is also always positive.

It is the second source that provides the actual power dissipated in the load, not to mention the power dissipated in the transistor itself.

\$\endgroup\$
1
  • \$\begingroup\$ If there is a DC source in series with \$V_{in}\$ then what is \$I_i\$? How they write \$I_i= V_i/R_i = 10mA\$ what is \$R_i\$ is this the static resistance or the dynamic? Would you explain anything? At least tell me some resource where i could know in detail about this article( given in the book that i mentioned in my question). \$\endgroup\$
    – user31782
    Commented Feb 22, 2014 at 14:11

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.