Graphical Intuition for Band Pass/Stop Active Filter Responses to Square Wave Input?

Question

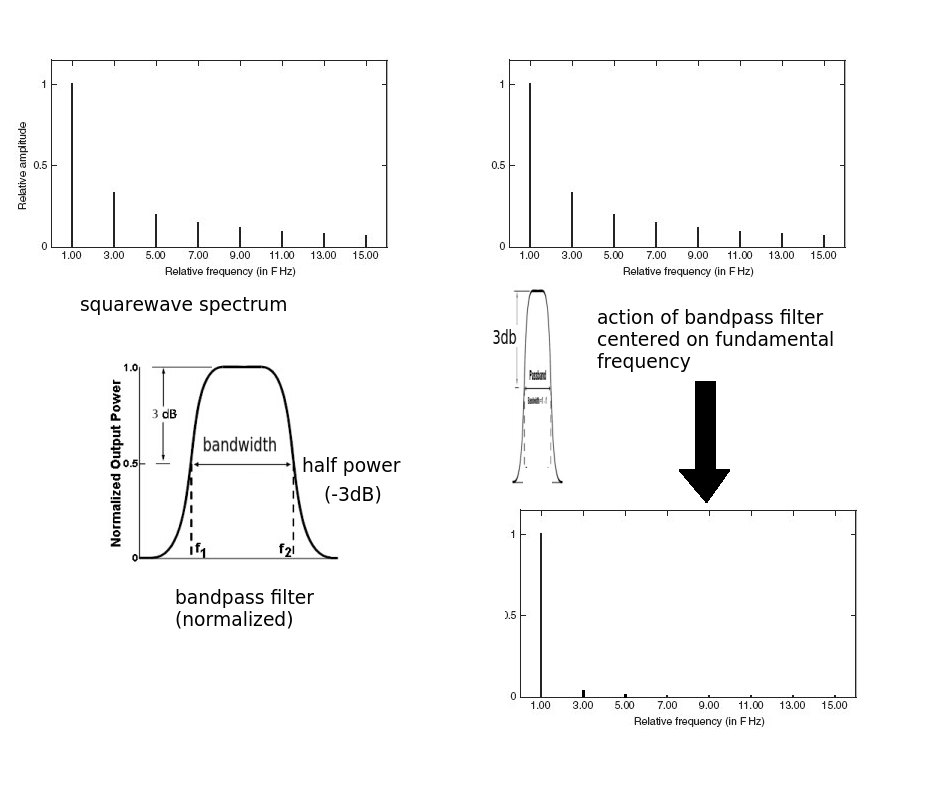
In reading my text, it notes that, when a square wave of a certain frequency is applied to band pass/ band stop active op-amp filters:

• Band pass filters will output the fundamental frequency of the square wave multiplied by the gain at the center frequency.

• Band stop filters will carry all the harmonics of the square wave other than the fundamental.

I have some idea of what this means, but I would really like to see a graphical representation to confirm. I've searched my book, but it doesn't have any accompanying graphs. Not had any luck on the Internet either, though it may be a case of me searching for the wrong thing.

Can anyone help me out? It would be greatly appreciated!

Answer 1

The two statements need a small but important caveat.

Statement 1: Band pass filters will output the fundamental frequency of the square wave multiplied by the gain at the center frequency - provided the center frequency of the filter is the same as the fundamental and the bandwidth of the filter is narrow enough to filter out the 3rd harmonic (3 x fundamental).

If the filter has a gain or loss then this is simply applied to the amplitude of the fundamental frequency, just in the same way you would treat the signal passing through any amplifier or attenuator. Note that the harmonics don't actually disappear - they are simply reduced in amplitude.

Statement 2: Band stop filters will carry all the harmonics of the square wave other than the fundamental - provided it is a high pass filter and the cut off frequency is higher than the fundamental.

Note that a low pass filter may act like a bandpass filter if the cutoff frequency is greater than the fundamental.

Both statements also assume perfect filters with very steep cut off slopes and flat tops.