BJT Class A Amplifier - Help calculating resistor values \$R_C\$ and \$R_E\$

Question

I'm having difficulty working out required values of resistors \$R_C\$ and \$R_E\$ in the circuit below. Can't seem to find any formulas on the web.

I know how to find \$R_1\$ and \$R_2\$:

$$R_1 = (\frac{R_2}{V_B} \times V_{CC})-R_2$$

$$R_2 = \frac{β_{DC} \times R_E}{10}$$

I'd appreciate any help you can give with this as I can't move forward with other calculations until I find values for \$R_C\$ and \$R_E\$.

Technical specs of circuit:

• BJT BC108
• Voltage divider bias used
• \$I_{C(sat)} = 20mA\$
• \$V_{CC} = 10V\$
• 10μF coupling capacitors for the input and output connections
• 100μF capacitor for the bypass capacitor
• \$β_{DC} ≈ 200\$
• Voltage gain without bypass capacitor = 1.5

I plotted a DC Load Line and the Q-point is at \$I_C = 10mA\$ and \$V_{CE} = 5V\$.

Answer 1

You have 4 resistors and thus, 4 degrees of freedom. You need four independent and consistent design constraints to find a unique set of four resistor values.

Some of the possible design constraints are:

(1) input impedance

(2) output impedance

(3) AC gain

(4) DC collector current

The input impedance is approximately

$$Z_{in} = R_1||R_2||r_{\pi} $$

The output impedance is approximately

$$Z_{out} = R_C||r_o $$

The AC gain is approximately

$$A_v = -g_mR_C||r_o $$

The DC collector current is approximately

$$I_C = \frac{V_{BB} - V_{BE}}{\frac{R_{BB}}{\beta} + \frac{R_E}{\alpha}} $$

where

$$V_{BB} = V_{CC}\frac{R_2}{R_1 + R_2} $$

$$R_{BB} = R_1||R_2 $$

Since the only constraints you've specified are \$I_C\$ and \$V_{CE}\$, you must use some engineering judgement ('best guess', 'rules of thumb') to justify your choice of resistor values as, e.g, Andy aka has demonstrated.

As another example of how to proceed, let's first calculate the small signal parameters:

$$g_m = \frac{I_C}{V_T} = \frac{10mA}{25mV} = 0.4S$$

$$r_{\pi} = \frac{\beta}{g_m} = \frac{200}{0.4S} = 500 \Omega$$

$$r_o = \frac{V_A}{I_C} = \frac{80V}{10mA} = 8k\Omega$$

Now, it is clear that the input impedance must be less than \$r_{\pi}=500\Omega\$ which is quite low.

Assume that the desired (magnitude) voltage gain is \$|A_v| = 100\$, then

$$R_C \approx \frac{|A_v|}{g_m}=\frac{100}{0.4S} = 250\Omega $$

Since \$R_C<<r_o\$, we can ignore \$r_o\$ from here.

The DC collector voltage will be

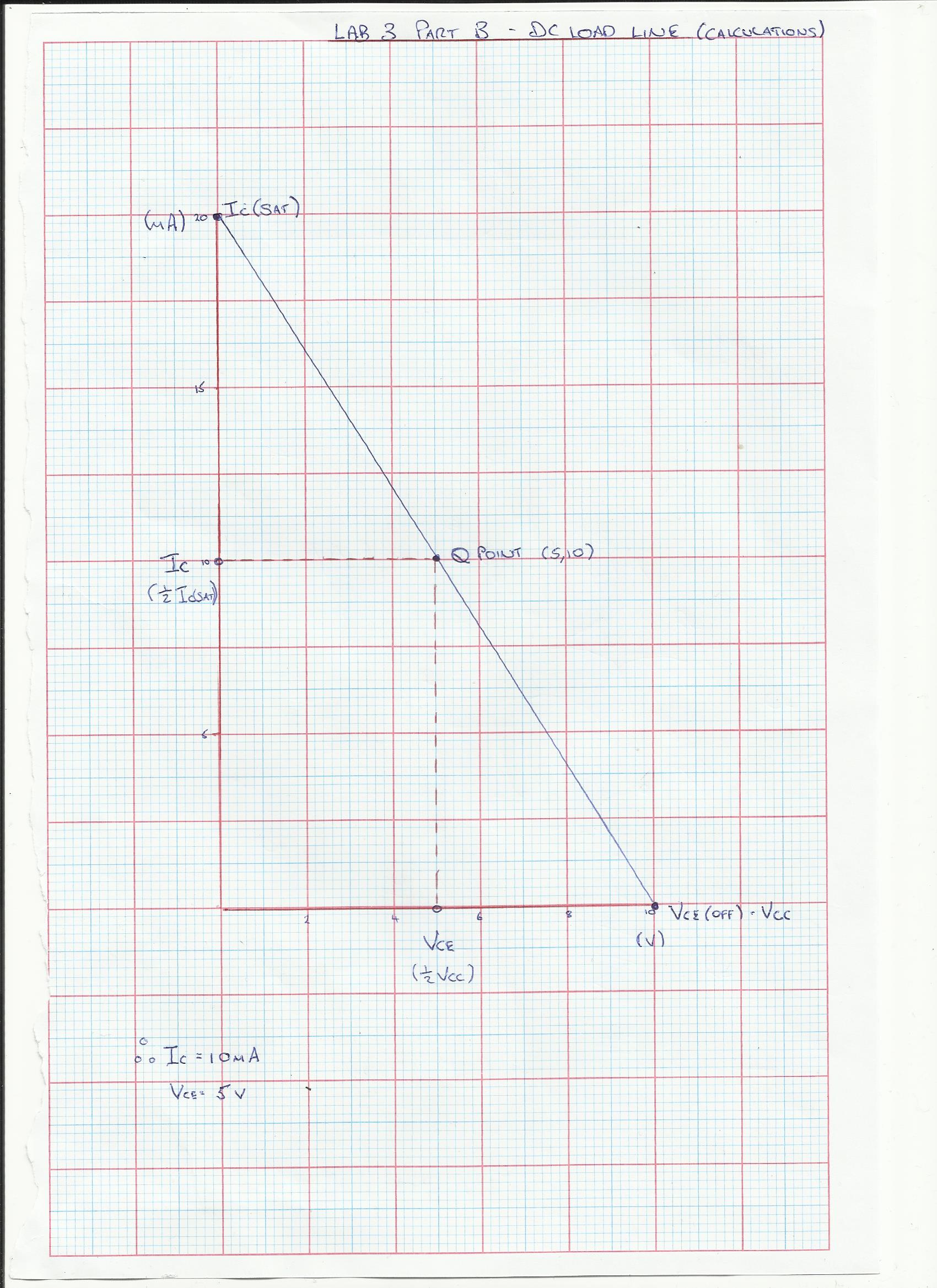
$$V_C = V_{CC} - I_C R_C = 10V - 10mA \cdot 250\Omega = 7.5V$$

You've specified that \$V_{CE} = 5V\$ so the DC emitter voltage is

$$V_E = V_C - V_{CE} = 7.5V - 5V = 2.5V$$

Thus, the required value for \$R_E\$ is

$$R_E = \frac{V_E}{I_E} \approx \frac{V_E}{I_C} = \frac{2.5V}{10mA} = 250\Omega$$

Assuming \$V_{BE} = 0.7V\$, the voltage across \$R_2\$ is

$$V_{R2} = V_E + V_{BE} = 2.5V + 0.7V = 3.2V$$

Now, a rule of thumb for operating point stability is to set the current through \$R_2\$ to be 10 times the DC base current

$$I_{R2} = 10\cdot I_B = 10 \cdot \frac{I_C}{\beta} = \frac{10}{200}10mA = 500\mu A $$

Thus, the required value of \$R_2\$ is

$$R_2 = \frac{V_{R2}}{I_{R2}} = \frac{3.2V}{500\mu A} = 6.4k\Omega$$

By KCL, the current through \$R_1\$ is

$$I_{R1} = (10 + 1)I_B = 11 \cdot 50\mu A = 550 \mu A $$

The voltage across \$R_1\$ is

$$V_{R1} = V_{CC} - V_{R2}= 10V - 3.2V = 6.8V $$

Thus, the required value for \$R_1\$ is

$$R_1 = \frac{V_{R1}}{I_{R1}} = \frac{6.8V}{550\mu A} = 12.4k\Omega$$

Using E96 (1%) values for the resistors yields

$$R_1 = 12.4k\Omega $$

$$R_2 = 6.34k\Omega $$

$$R_E = 249\Omega$$

$$R_C = 249\Omega$$

Answer 2

Warning - rules of thumb being used!!

I always try and get about 0.5 to 1V on the emitter (lets say 0.75V). This means the base will be at about 1.45V and this allows you to calculate R1 and R2 (but be prepared to revise those values should the base current drawn be a little high).

You want 10mA thru the collector and this is virtually the same current through the emitter so now you can decide what Re is - 0.75V/10mA = 75 ohms.

At 10mA collector current you want collector to be half rail so that means Rc is 5V/10mA = 500 ohm. Another rule of thumb - current thru R1 and R2 should be about one-tenth of Hfe lower than Ic. If Hfe is 200 then current in R1 and R2 is about 10mA/20 = 0.5mA.

R1 + R2 therefore should equal 10V/0.5mA = 20 kohm.

R2 needs to be smaller than R1 to develop about 1.45V on the base and on a 10V supply I can immediately see that R2 being about 2k9 would take 0.5mA to develop 1.45V. This leaves R1 as 17k1 ohms.

I'd use 3k0 for R2 and an 18k for R1 for practical reasons of obtainability.

C3 depends on your lowest frequency input and how much voltage gain you need. Without C3 there is a voltage gain of approximately Rc / Re = 6.667. If you want more gain use C3 but be prepared to put a small resistor in series with it to linearize the gain over the full desired bandwidth.

Answer 3

there is difference between voltage and power amplifiers in class A:

the distinction of between voltage and power amplifier is somewhat artificial since useful power (product of voltage and current) is always developed in the load resistance through which current flows.the difference between the two types is really one of degree.it is a question of how much voltage and how much power.a voltage amplifier is designed to achieve maximum voltage amplification.it is however no important to rise the power level.on the other hand a power amplifier is designed to obtain maximum output power.

voltage amplifier: the transistor with high beta is used in the circuit.(beta > 100) the input resistance of the transistor is sought to be quite low as compared to the collector load Rc. a relatively high load Rc is used in the collector.voltage amplifier are always operated at low collector current.(Rc=4 to 10k) output impedance:(high ~12kΩ)

power amplifier: the size of power transistor is made considerably larger in order to dissipate the heat during operation. the transistor with low beta is used in the circuit.(beta=5 to 20) output impedance:(low ~200Ω)