Find Vs in the circuit (not homework)

Question

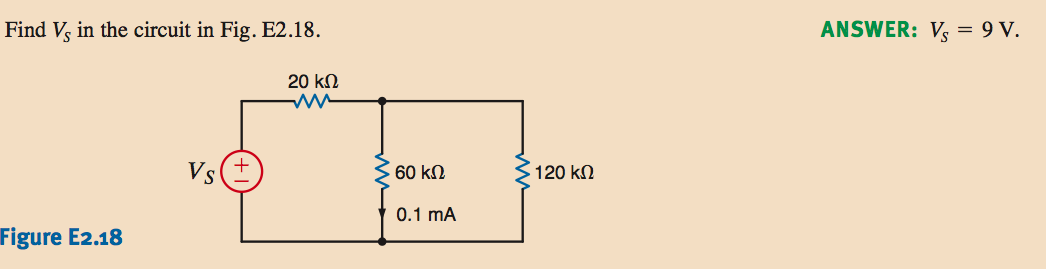
So I solved this using current division and KVL to find \$V_s\$, but I wonder if there is a simpler way to find \$V_s\$ in this circuit.

(This is a textbook example, not homework; the given answer is \$V_s = 9\text{V}\$).

Will someone verify my procedure and let me know if there is a better way to do this problem?

Labels: \$R_1 = 20\text{k}\Omega\$, \$R_2 = 60\text{k}\Omega\$, \$R_3 = 120\text{k}\Omega\$

 Step 1:  Using current division, I_R2 = 0.1mA = (120k/180k)*I_R1
          The unknown here is I_R1.  So, that gives I_R1 = 0.0001 A * (3/2) = 0.00015A.    
 Step 2:   V_R1 = 0.00015A * 20k ohms = 3V
 Step 3:   V_R2 = 0.0001A * 60k = 6V
 Step 4:   Setup KVL around the left loop:   -Vs + 3V + 6V = 0
 Step 5:   Isolate Vs and simplify the KVL equation:   Vs = 9V

So, that's correct, but, did I get the answer correct by chance, or did I find the correct procedure? Also, what could I have done to simplify the process?

Answer 1

I've worked in electronics all my life (and am now retired). I perhaps did do calculations based in Kirchoff's laws, current division, etc in school, but now just consider Kirchoff's laws to be scientific wording of what should be simple, common-sense observations.

When I look at this problem, I see the 0.1 mA through the 60K resistor. I see that there is a resistor of twice that value (120K), in parallel, so it will have half the current of the 60K, so the total current in the circuit will be 0.15 mA.

The voltage across the 60K resistor is 60K x 0.1 mA, or 6 volts.

The voltage across the 20K resistor is 20K x 0.15 mA, or 3 volts, so Vs is 6 + 3 = 9 volts.

Answer 2

So I solved this using current division and KVL to find Vs, but I am posting because I wonder if there is a more simple way to find Vs in this circuit.

An answer has been given and accepted but, for a different perspective, I give another approach using voltage division.

By inspection, the voltage across the parallel resistors is given by the voltage divider:

$$V_{60k} = \frac{60k||120k}{20k + 60k||120k}V_S = \frac{2}{3}V_S$$

since we already have this voltage, simply invert and solve:

$$V_S = \frac{3}{2}V_{60k} = \frac{3}{2}(60k\Omega \cdot 0.1ma) = 9V $$