0
\$\begingroup\$

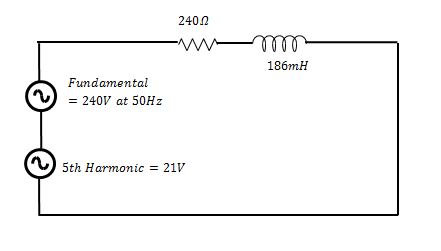
figure 1

I need help with the above circuit.

I first need to calculate the voltage and current distortion. I calculate this as 8.75%(voltage) and 5.7% (current).

I then need to find the displacement power factor. I calculate the current through the circuit using Ohm's law for the fundamental voltage and treat the harmonic as a short circuit and I get 0.97A<-13.68°.

For the fundamental:

Z(inductor) = 186m * 2pi * 50 j = 58.43j

By Ohm's Law: 240 = I(240 + 58.43j); I = 0.97A<-13.68°

For the 5th harmonic:

Z(inductor) = 186m * 2pi * 50 j *5 = 292.168jΩ

21 = I(240 + 292.168j); I = 0.056A<-50.6°

Therefore I assume the displacement power factor is cos(-13.68) as the voltage has no phase. This gives me a power factor of 0.97.

I then need to calculate the true power factor. I use the real power/apparent power equation. I find the total voltage in the circuit (RMS) to be 170.34V and the total current (RMS) to be 0.678A.

Total voltage = sqrt(harmonic voltage^2 + fundamental voltage^2) (all rms)

Total voltage = sqrt(169.7^2+ 14.85^2) = 170.34V

Total current = sqrt(harmonic current^2 + fundamental current^2) (all rms)

Total current = sqrt(0.686^2+ 0.039^2) = 0.678A

I multiply these two together for an apparent power of 115.49 VA. I then use I^2*R to find the real power on the basis that the impedance of the resistor will not vary with frequency. I obtain a true power factor of 0.95.

Have I approached this question in the right way and is my answer correct? Would appreciate some feedback/criticism.

\$\endgroup\$
3
  • \$\begingroup\$ Maybe show your working so others can follow instead of expecting others to repeat the process. \$\endgroup\$
    – Andy aka
    Commented Jul 2, 2014 at 19:27
  • \$\begingroup\$ Added the working \$\endgroup\$
    – user2802349
    Commented Jul 2, 2014 at 19:58
  • \$\begingroup\$ Displacement power factor is a new term to me. I understand power factor and I also understand that there can be several power factors (each harmonic). I was in agreement up till cos(13.68) then I wrote this. Maybe someone else can help from hereonin \$\endgroup\$
    – Andy aka
    Commented Jul 2, 2014 at 20:18

1 Answer 1

Reset to default
1
\$\begingroup\$

A definite mistake is your method of calculating total RMS voltage. In the absence of anything else other than "240V" and "21V", you have to assume these are RMS but you have assumed they are peak.

You could argue that you are right but not really, because you used them as RMS voltages earlier on to calculate currents and these will also be RMS values that you don't need to divide by sqrt(2) either.

Other than that and me not understanding the term "displacement power factor" and "true power factor" you are correct.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.