What is the conductance of this LC tank?

Question

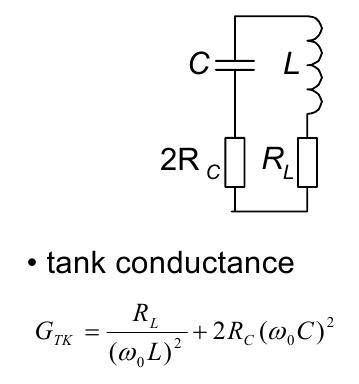
So, I start with total conductance:

$$ G_{TK} = \frac{1}{\frac{1}{j\omega C}+ 2R_C} + \frac{1}{j\omega L + R_L} $$

Then, when I take only the real part of this expression in order to know the conductance at the resonance frequency, I end up with the following expression:

$$ G_{TK} = \frac{2R_C}{\frac{1}{C^2\omega_0^2}+ 4R_C^2} + \frac{R_L}{L^2\omega_0^2 + R_L^2} $$

It's clear that the \$4R_C^2\$ and \$R_L^2\$ were discarded in order to arrive at the expression in the image. However, what is the full argument behind? I'm guessing it's something along the lines of: $$ \frac{1}{C^2\omega^2} \gg 4R_C^2 $$

and the same for the other expression.

The question is: WHY? The problem is that I'm not familiar at all with the practicalities and typical values for LC tanks. If I were not shown the final expression, I wouldn't have known that one term dominates. So, could anyone maybe point me to the right source in order to read more about this LC tank practicalities?

Thank you all!

Answer 1

looks like your guess is right. Imagine frequency = 0, then the inductor becomes a conductor and only \$R_L\$ is responsible for the impedance. on the other end of the spectrum, \$\omega \rightarrow \infty\$ only \$R_c\$ makes for the impedance. So at \$\omega_0\$ the \$L\$ and \$C\$ termes most likely dominate and are much greater than the corresponding resistors. This is due to the fact that at \$\omega_0\$ the overall-impedance is the highest and if \$C\$/\$L\$ do not dominate the resistances at this frequency, then they don't at all and all we have is the resistances anyway.

These resistors probably represent the parasitic resistances which have an effect by decreasing the Q-factor (and therefore are small compared to the corresponding Inductance/Capacitance) So if you assume just that your calculations yield your given formula.