Compute for resistance (Resistance circuit)

Question

Hi how do you solve this problem? I am confused.

^{simulate this circuit – Schematic created using CircuitLab}

Find \$R_{AB}\$, \$R_{CD}\$, \$R_{EF}\$, \$R_{AE}\$

I came up with this: \$R_{A-B}\$ = 1 \$\Omega\$ , \$R_{C-D}\$ = 4 \$\Omega\$ , \$R_{E-F}\$ = 7 \$\Omega\$ , \$R_{A-E}\$ = 2 + 5 = 7 \$\Omega\$

Answer 1

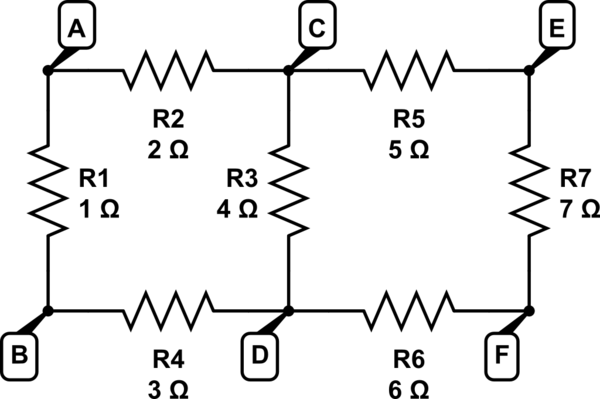
Have you covered equivalent resistances? Essentially, it is a way to combine resistors in series or parallel until you get a single resistance, working your way from out to in.

Let's say you want to find \$R_{AB}\$.

First, your have a series combination \$R_5\$, \$R_6\$, and \$R_7\$. $$ R_{5,6,7} = R_5 + R_6 + R_7 = 18 \Omega $$

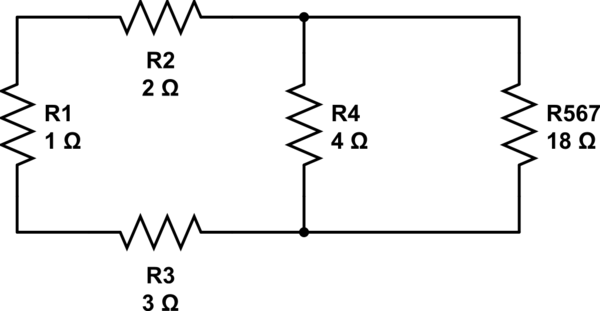
^{simulate this circuit – Schematic created using CircuitLab}

Now you have a parallel combination between \$R_4\$ and \$R_{5,6,7}\$. $$ R_{4,5,6,7} = \frac{R_4 \cdot R_{5,6,7}}{R_4 + R_{5,6,7}} = 3.27 \Omega $$

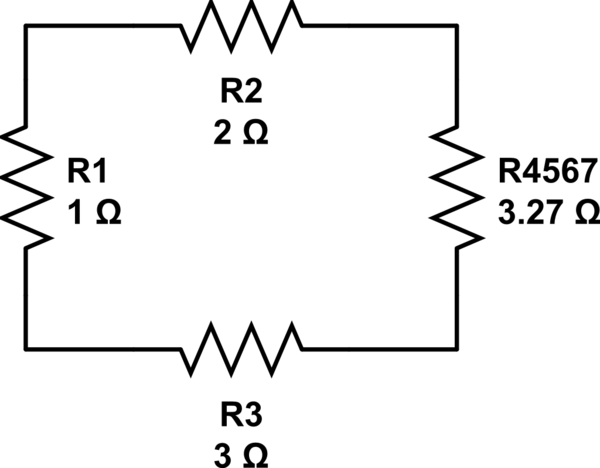
^{simulate this circuit}

You are almost done! Now, since you are looking for the equivalent resistance between A and B, you have a series combination for \$R_2\$, \$R_3\$ and \$R_{4,5,6,7}\$: $$ R_{2,3,4,5,6,7} = R_2 + R_3 + R_{4,5,6,7} = 8.27 \Omega $$

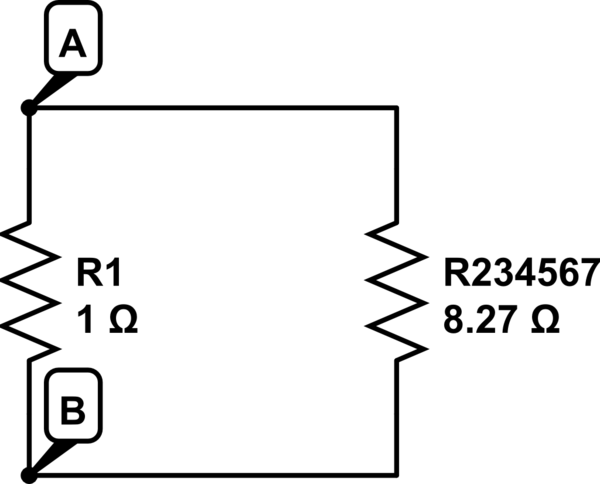
^{simulate this circuit}

Last Step!

You have a parallel combination between \$R_1\$ and \$R_{2,3,4,5,6,7}\$: $$ R_{AB} = \frac{R_1 \cdot R_{2,3,4,5,6,7}}{R_1 + R_{2,3,4,5,6,7}} = 0.892\Omega $$

Answer 2

$$ R_{ab} = (((5 + 7 + 6) || 4) + 2 + 3) || 1 $$

Combine the resistors in series and parallel until you end up with a single resistance between the two nodes and you have your answer.

I believe for \$R_{ae}\$ one would need to employ a wye-delta transform.