0
\$\begingroup\$

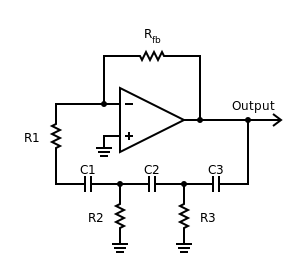
enter image description here

Figure show a RC phase shift oscillator using Op-Amp with gain 5(Vo/Vi).

My assumption : Circuit is generating a sine wave with a peak to peak voltage of 10v.

Consider positive maximum point (+5V) at the output. A fraction of this voltage is feed to inverting terminal of Op-amp with a 180 degree phase shift. ie -1V ( 180 degree phase shifted value 1/5 of 5 ie barkhausen criteria) reaches negative terminal of Op-amp. This -1V is again amplified by the Op-amp and we will get +5v at the output. That means this is a stable condition.

Then why output voltage is oscillating ?

\$\endgroup\$

3 Answers 3

Reset to default
1
\$\begingroup\$

There is stable oscillation if the Barkhausen criterion for oscillation is satisfied.

I.e:

  • loop gain is 1
  • (total) loop phase shift is 0°, (or 360° or an integer multiple of 360°):
    in this circuit there is 180° phase shift by the inverting input of the OpAmp and another 180° by the phase shift network which results in 360° for exact one frequency.
\$\endgroup\$
0
\$\begingroup\$

The output is oscillating because it takes time for the changes in the output voltage to propagate through the filter.

\$\endgroup\$
2
  • \$\begingroup\$ 0 phase shift means there is no delay. Am I right ? \$\endgroup\$
    – tollin jose
    Commented Sep 22, 2014 at 15:37
  • \$\begingroup\$ not necessarily, since a 360 degree delay also exhibits zero phase shift. \$\endgroup\$
    – EM Fields
    Commented Sep 22, 2014 at 17:24
0
\$\begingroup\$

Tollin - you cannot pick up one single moment (example: Why do you think that +5V at the output are producing -1V at the inv. input?).

Rather, you have to consider steady-state condition - and now Barkhausen´s oscillation condition comes into play: A circuit with feedback is able to oscillate if the loop gain around the complete loop is unity (magnitude unity, phase=0) for one single frequency only. The feedback network in your circuit produces a phase shift of -180deg at one single frequency - and the damping at this frequency is 1/29. Therefore, when you close the loop with an inverting amplifier (gain of -29) you fulfill the mentioned condition and the circuit oscillates.

Comment: The above mentioned factor of 1/29 applies if R=R1=R2=R3 and C1=C2=C3 and Rfb=29*R

\$\endgroup\$
2
  • \$\begingroup\$ By Barkhausen criteria loop gain should be 1. I already assumed gain of amplifier as 5, therefore gain of feed back network must be 1/5) since output voltage is 5V, 1/5 of 5 ie 1V will reach input end. Since there is 180 degree phase shift feed back value will be -1V. \$\endgroup\$
    – tollin jose
    Commented Sep 22, 2014 at 15:43
  • \$\begingroup\$ ....but it isn`t 1/5 at the frequenncy which produces -180deg phase shift. You cannot simply "assume" something which contradicts electronic rules. In fact, it is 1/29. \$\endgroup\$
    – LvW
    Commented Sep 22, 2014 at 16:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.