11
\$\begingroup\$

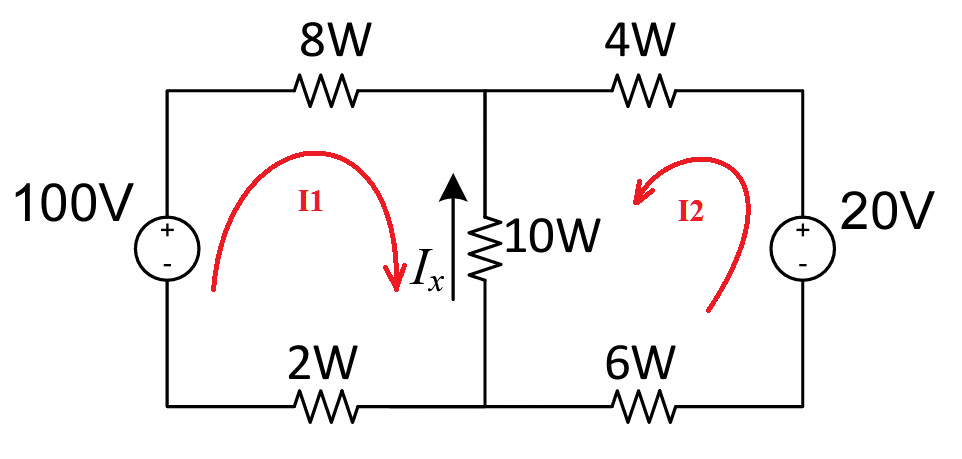
In my electrical circuits class, we've spent the last six weeks learning circuit analysis with resistors rated in ohms. Now, out of the blue, every question on the next practice exam has the resistors rated in the watts they consume. Here is a question from the practice exam where I'm supposed to find Ix.

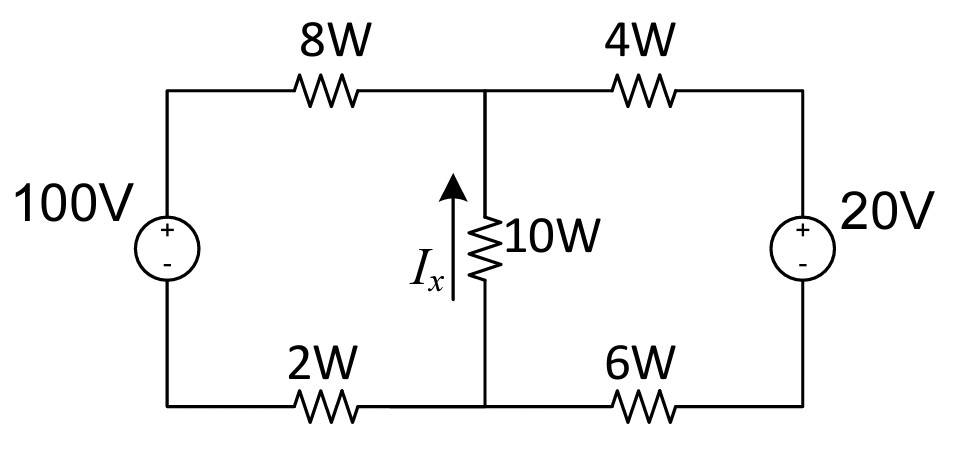
Circuit

Am I really supposed to find all of the node voltages by substituting the resistance values with V^2/P (or some manipulation of it) and then doing a loop current analysis? That gives a bunch of quadratic equations instead of making things easier.

I feel like I'm overlooking something mind-blowingly simple here. I've spent the last few hours searching for help on this and I can't find anything for this type of problem. Any help to point me in the right direction would save what little hair I have left on my scalp.

\$\endgroup\$
2
  • \$\begingroup\$ As others have said - it's probably an Omega/R ont issue BUT looking at how one might solve if dissipation WAS intended is interesting. | Out of head - may be wrong - In either case currents are not changed if you change resistor positions on the same leg. So the 2W and 8W can be combined as a 10W and the 6W + 4W as a 10W. Put both these above their supplies and you have the 100V and 20V with a common ground and the problem LOOKS somewhat simpler. If you think of the 20V as opposing current from the 100V leg and not causing dissipation it suggests I upper left leg = P/V = 30W/100V = 0.3A. .... \$\endgroup\$
    – Russell McMahon ♦
    Commented Oct 15, 2014 at 1:24
  • \$\begingroup\$ .... now the current divides such that current at centre point produces 10W both ways. Vc is centre point voltage. Power is 10W in left leg with Vc applies and in right leg with (Vc-20) applied. P = V x I so I = P/Vc. Ileft = 10/VC. Iright = 10/(Vc-20) | So ... Looks like it may yet work :-) E& OE !!!!!!!!!!!!!!!! \$\endgroup\$
    – Russell McMahon ♦
    Commented Oct 15, 2014 at 1:29

3 Answers 3

Reset to default
33
\$\begingroup\$

The symbol for Ohms is the capital letter Omega, \$\Omega\$. In some word processors if you make the Omega symbol using a Greek font and then convert it to another font like Times New Roman or Arial, then that symbol will show up as a "W.". In other words, your professor probably used the wrong font and those are meant to be Omega's.

\$\endgroup\$
8
  • 11
    \$\begingroup\$ +1 I see this frequently in badly made PDFs etc. \$\endgroup\$
    – Spehro 'speff' Pefhany
    Commented Oct 12, 2014 at 5:44
  • 11
    \$\begingroup\$ Wow, that's so dodgy it's not funny. \$\endgroup\$
    – KyranF
    Commented Oct 12, 2014 at 10:45
  • 1
    \$\begingroup\$ While this may in fact answer the question from a typographical standpoint, it would be nice to also mention if it is possible to do the calculation given resistors rated in watts effectively. \$\endgroup\$
    – nanofarad
    Commented Oct 12, 2014 at 18:11
  • 1
    \$\begingroup\$ Sadly not everyone has the conscience of swithing Ωs for Rs when the format doesn't support them. R is related to "resistance", and also looks like a squished Omega. \$\endgroup\$
    – Kroltan
    Commented Oct 12, 2014 at 22:30
  • 2
    \$\begingroup\$ @hexafraction Watt ratings for resistors are thermal ratings that indicate how much average power it can handle. Generally the wattage of all of the resistors in a circuit will be identical (e.g. 1/4 watt). So they serve a completely different purpose than the resistance and cannot be used to solve a circuit in the same way. However, if the values are power dissipation (not resistor ratings, but the product of the voltage and current) then it may be possible to solve a circuit, but you won't be able to use the conventional methods and there may not be sufficient information. \$\endgroup\$
    – alex.forencich
    Commented Oct 13, 2014 at 5:57
1
\$\begingroup\$

Since it's a practice exam, I encourage you to ask your instructor if you are on the right track or not before assuming it's some kind of typo. Especially if there is a way to solve the problem as stated, albeit somewhat cumbersome. Sometimes texts change the form of problems, assuming that the different approach has been discussed in class.

\$\endgroup\$
1
  • 2
    \$\begingroup\$ He confirmed that they were ohms (finally). The only reason that I felt safe in assuming that it was a typo when Brad answered is because there would be no way to get nine of them done in the 50 minutes we'll get. \$\endgroup\$
    – BStott
    Commented Oct 13, 2014 at 1:41
1
\$\begingroup\$

Let us try the Mesh Currents Method and see what we will get.

enter image description here

$$ \dfrac{8W}{I_1}+\dfrac{10W}{I_1+I_2}+\dfrac{2W}{I_1}=100V \\ (8W)(I_1+I_2)+(10W)I_1+(2W)(I_1+I_2) = (100V)I_1(I_1+I_2) \\ \boxed{(20W)I_1 + (10W)I_2 - (100V)I_1^2 - (100V)I_1I_2 = 0} \\ \\ \dfrac{4W}{I_2}+\dfrac{10W}{I_1+I_2}+\dfrac{6W}{I_2}=20V \\ (4W)(I_1+I_2)+(10W)I_2+(6W)(I_1+I_2) = (20V)I_2(I_1+I_2) \\ \boxed{(10W)I_1 + (20W)I_2 - (20V)I_1I_2 - (20V)I_2^2 = 0} \\ $$

Ends up with a non-linear equation set. The system doesn't seem to have a unique solution.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.