Power resistors equivalent thermal model: Interpretation of datasheet & 0°C terminal of power source

Question

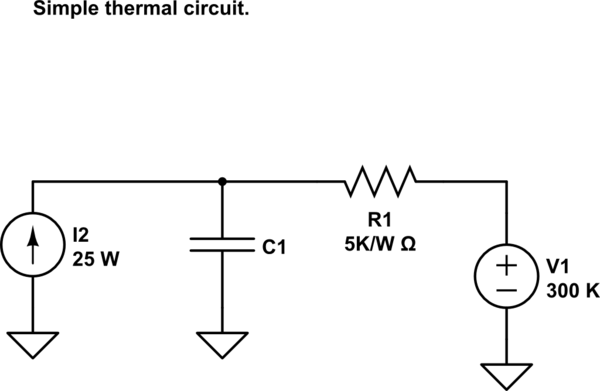
This is borderline electronics, but I think many more of us here have done this kind of studies.

I'm heating an aluminium plate with an Aluminium clad power resistor by mounting it on it and applying 25W of power, just like on the following schematic: 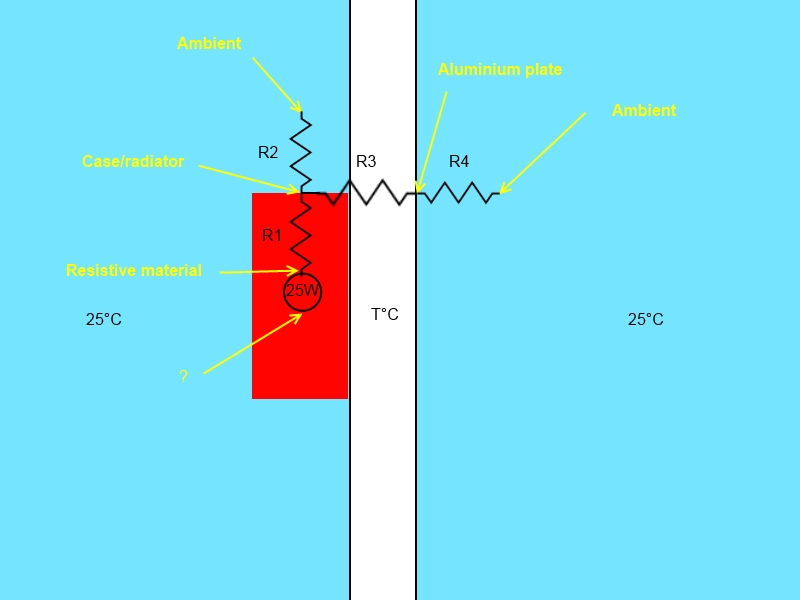Note the thermal equivalent model I think it corresponds to, including the temperature nodes.

1. What is the 0°C terminal of the power source connected to in that case? Ambient (as usual), or aluminium plate? How do we know?
2. I can estimate R3 (0.5K/W) and I have a rough calculation of R4 (4.7K/W which I find really really odd*), but on the datasheet of the resistor I'm using I don't know if the thermal impedance is R1+R2 (junction to ambient) or R1 (junction to case). Numbers seem to point to R1+R2, but how do I know R1 then?

Goal: Calculate the temperature T of the aluminium plate at equilibrium.

*: Addendum: I've used the 0.225m².K/W value of this link together with the surface of my plate to find that, which means a 360x131 mm² plate radiates less than the resistor heatsink?

Answer 1

I'll try an answer.
I'm confused by your first question but let me try this,

So in doing this type of modeling there is the following analogy.

• Temperature (T) = Voltage
• Heat (power) (W) = Current
• Heat capacity (J/K) = Capacitance (coulumbs/volt)
• Thermal resistance (K/W) = resistance (V/A)

^{simulate this circuit – Schematic created using CircuitLab}

So I usually make my temperature reference 0 K. (You could make it 0 C, but it gets confusing if you ever want to work at temperatures below zero C.) The temperature reference like a voltage reference is somewhat arbitrary.

So then my circuit does this. There is a 25W Heater (current source) feeding your Al plate. (I'm ignoring the thermal resistance in the source.. more below.) This goes into the Heat capacity of your plate. (if you tell me the volume of your plate then the heat capacity is about 3 J/K per cm^3.) This plate is connected to the outside world through a resistor to 300K. So you can now go ahead and solve this. And find out not only the final temperature of the plate, but also the time constant.. how long does it take to get there.

OK now the resistance of the heating element. You could add this in as a resistor between the current source and plate C. You only care about this if you want to know how hot the resistor inside will get. I assume the number in your link is to the case. I'd further ignore the convection loss of the heater body, (assuming it's small in area compared to the plate.)

Once I make this type of model I'll get a little thermo-couple and measure temperatures here and there and see how good the model is. (It looks like your plate will get hot.)