0
\$\begingroup\$

I have to implement a Moore machine that has an input L and an output Z.

The input line L provides values of 1 and 0 as input, in a random order.

The output line Z takes the value 1 whenever two values of 0 and two values of 1 have been detected in the input, no matter the order. For example the sequences 0011, 00001001 and 1010 would all set line Z to 1.

I have to use D flip-flops and NAND gates only but am stuck halfway.

So far I have drawn the state diagram and the table below: (CS stands for current state and FS stands for future state).

I know that I must draw 8 Karnaugh maps I think but I am not sure and I have to use 3 or 4 flip flops. 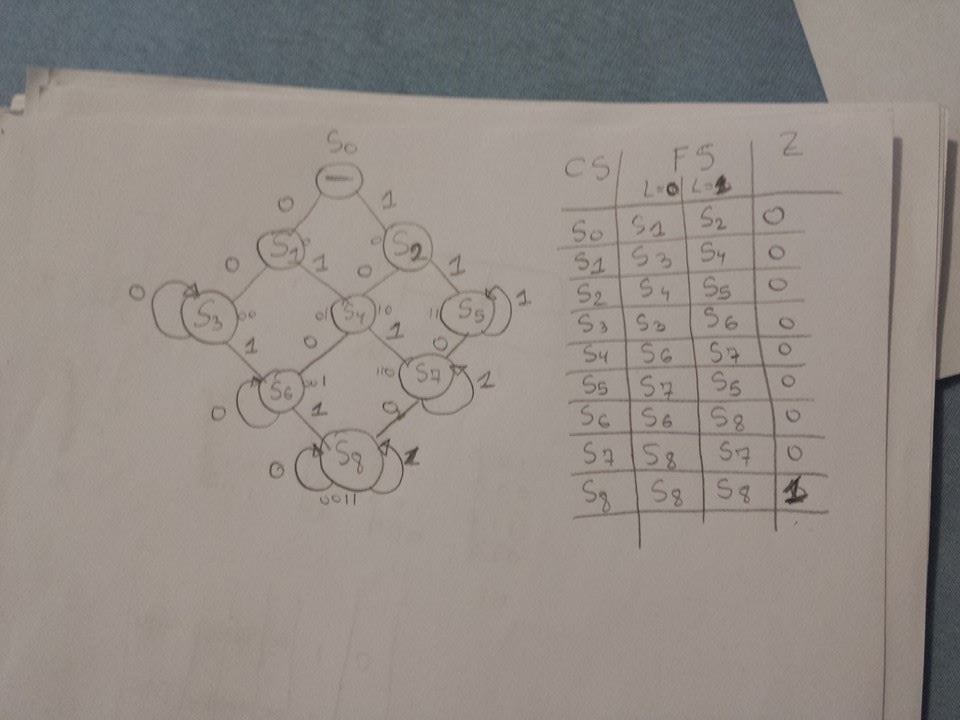state machine

Could you point me in the right direction?

EDIT After some more research I managed to finally draw the Karnaugh maps and extract the functions, and I designed a circuit using LogiSim. I am not sure what I have done wrong and have no idea how to proceed. The circuit is not doing what it's supposed to do. Here is a link to my LogiSim circuit.

\$\endgroup\$
2
  • \$\begingroup\$ Remember that you can build OR, NOR, NOT, AND, and NAND by combining NANDs. \$\endgroup\$
    – JimmyB
    Commented Dec 1, 2014 at 11:10
  • \$\begingroup\$ Maybe try to design a circuit which will detect and store the first 1 (or 0) bit it sees. Then combine some of those elements to the complete circuit. \$\endgroup\$
    – JimmyB
    Commented Dec 1, 2014 at 11:12

1 Answer 1

Reset to default
3
\$\begingroup\$

Being kind of old myself, I expect you to study like #### too. :D

One question may help clarify the circuit you need : how does it distinguish between 2 successive states that are the same? Or alternatively : Is there a separate clock signal, not mentioned above? If so, the basic pattern of the circuit may become clear.

Ask yourself :
how many states do you have?
how many bits are required to implement all these states?

You have already given each state a unique number : it will help to write those numbers out in binary as part of each state table.

Then treat each bit of that number separately : first create a Karnaugh map for the next state for bit 0. What are the input variables for that KMap?

\$\endgroup\$
1
  • \$\begingroup\$ can you look over my circuit and give me some feedback? \$\endgroup\$
    – rares985
    Commented Dec 8, 2014 at 15:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.