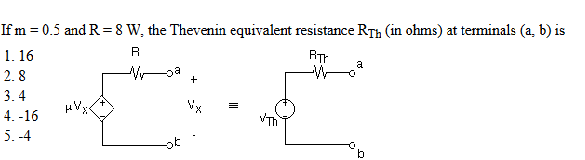
The answer is 16, but can anyone please explain how? note m is u
I confused because, I dont know how to solve for Vx ? I know that once I find Vth and then resolve the circuit closed and finding Isc I can find Rth by ohms law.

The answer is 16, but can anyone please explain how? note m is u
I confused because, I dont know how to solve for Vx ? I know that once I find Vth and then resolve the circuit closed and finding Isc I can find Rth by ohms law.
This is one of those pesky cases where the standard procedure for finding the open circuit voltage or the short circuit current leads to "funny" results.
Another way to compute the Thevenin equivalent resistance of a network N is the following:
(1) Disable all independent voltage/current sources inside N, i.e. current sources replaced by open circuits, voltage sources by short circuits. Dependent sources remain untouched.
(2) Apply an (ideal) voltage \$V_0\$ (current \$I_0\$) source across the terminals of N.
(3) Compute the current \$I_0\$ entering (the voltage \$V_0\$ across) the terminals of N
(4) Compute the equivalent resistance as \$ R_{th} = \dfrac{V_0}{I_0} \$
In our case, applying a voltage source \$V_0\$ to the output makes \$V_x=V_0\$, thus it is easy to see that the current entering the network is:
\$ I_0 = \dfrac{V_0 - \mu V_0}{R} = (1-\mu) \dfrac{V_0}{R} \$
Hence:
\$ R_{th} = \dfrac{V_0}{I_0} = \dfrac{R}{1 -\mu} = \dfrac{8 \Omega}{1 - 0.5} = 16\Omega \$
EDIT (to answer a comment and make the answer more complete)
If you ask yourself what is the equivalent Thevenin's voltage you may puzzled: is it \$\mu V_x\$ or simply \$V_x\$?
Well, according to Thevenin's theorem \$V_{th}\$ must be the open-circuit voltage across terminals a-b, hence both answers are correct!
In fact, when nothing is applied to a-b the following equation must be valid:
\$ \mu V_x\$ = \$V_x \$
This means that the only valid value for a non-zero \$\mu\$ is \$V_{th}=0\$.
In the end, the entire circuit is simply equivalent to a single \$16\Omega\$ resistor!
Vth is not asked but how would you calculate it here? Is it Vx or μ*Vx?
\$\endgroup\$