Maximum output and efficiency for a simple transistor based amplifier circuit

Question

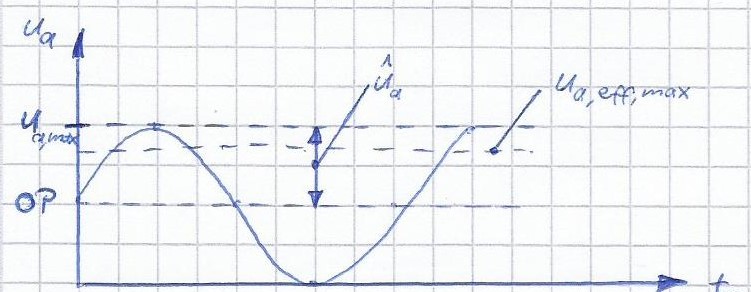
1. Question

What is the maximum output over \$R_L\$ and the maximum efficiency \$\eta\$ for the amplifier circuit shown below:

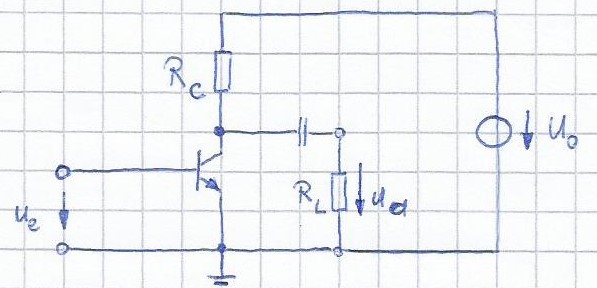
Below you will find my solution. Is this done correctly? What would you have done differently?

2. Voltage over \$R_L\$

Assumed is an ideal transistor with infinite current amplification \$\beta\$ and saturation voltage \$U_{ CE_{ sat } }\$ to be 0. Therefor the base current is 0 and the voltage drop over the transistor is considered constant for the whole output range. Furthermore is the output voltage \$u_a\$ sinusoidal.

This means for $$u_a(t)=\hat{U}_a\sin(\omega t)$$ the amplitude \$\hat{U}_a\$ is the voltage divider over \$R_L\$, when the maximum of the dynamic range of the transistor is reached, that no current flows over the transistor.

$$U_{a,\,max}=U_0\frac{R_L}{R_L+R_C}$$ $$\hat{U}_{a,\,max}=\frac{U_{a,\,max}}{2}$$ $$U_{a,\,eff,\,max}=\frac{\hat{U}_{a,\,max}}{\sqrt{2}}$$

The root mean square leads to:

$$U_{a,\,eff,\,max}=\frac{U_0}{2\sqrt{2}}\frac{R_L}{R_L+R_C}$$

3. Maximum output \$P_L\$ over \$R_L\$

$$P_L=\frac{1}{2}\frac{U_{a,\,eff,\,max}^2}{R_L}$$ $$P_L=\frac{U_0^2}{16}\frac{R_L}{(R_L+R_C)^2}$$

For \$\frac{dP_L}{dR_L}=0\$ leads \$R_L=R_C\$ to the maximum output over \$R_L\$.

$$P_{L,\,max}=\frac{1}{64}\frac{U_0^2}{R_L}$$

4. Maximum efficiency \$\eta\$

The total circuit power is the voltage over both resistors which is \$U_0\$:

$$P_{in}=\frac{1}{2}\frac{U_0^2}{R_L+R_C}$$

With the given condition that \$R_L=R_C\$: $$P_{in}=\frac{1}{4}\frac{U_0^2}{R_L}$$

The maximum efficiency \$\eta\$ is: $$\eta=\frac{P_L}{P_{in}}=\frac{1}{16}=6.25\%$$

Answer 1

What if we start with:

$$U_{a,eff,max} = \frac{U_0}{2\sqrt{2}}\frac{R_L}{R_L+R_C}$$

Then we get:

$$P_L = \frac{(U_{a,eff,max})^2}{R_L}=\frac{(\frac{U_0}{2\sqrt{2}}\frac{R_L}{R_L+R_C})^2}{R_L} = \frac{U^2_0R^2_L}{8R_L(R_L+R_C)^2}=\frac{U^2_0R_L}{8(R_L+R_C)^2}$$

And with \$R_C=R_L\$ we get for\$P_{L,max}\$:

$$P_{L,max}=\frac{U^2_0}{32\cdot R_L}$$

For the circuit power we have to consider the power loss of \$R_C\$ and the losses of the transistor itself.

$$P_{R_C} = P_{R_C,DC}+P_{R_C,AC}$$ $$P_{R_C,DC} = \frac{U_{R_C,DC}^2}{R_C}=\frac{(\frac{1}{2}(U_{R_C,max}+U_{R_C,min}))^2}{R_C}=\frac{(\frac{1}{2}(U_0+U_{a,max}))^2}{R_C}$$

If we set again \$R_C=R_L\$ then \$U_{a,max} = \frac{1}{2}U_0\$ and \$U_{R_C,DC}=\frac{3}{4}U_0\$ we get:

$$P_{R_C,DC} = \frac{9U^2_0}{16R_C}$$

For the AC-Part follows:

$$P_{R_C,AC}=\frac{(U_{R_C,eff})^2}{R_C}=\frac{(\frac{U_{R_C,max}-U_{R_C,DC}}{\sqrt{2}})^2}{R_C}=\frac{(\frac{U_{0}-\frac{3}{4}U_{0}}{\sqrt{2}})^2}{R_C}=\frac{U^2_0}{32R_C}$$

Finlay we get:

$$P_{R_C} = \frac{9U^2_0}{16R_C} + \frac{U^2_0}{32R_C} = \frac{19U^2_0}{32R_C}$$

Next how do we get \$P_{transistor}\$ ?