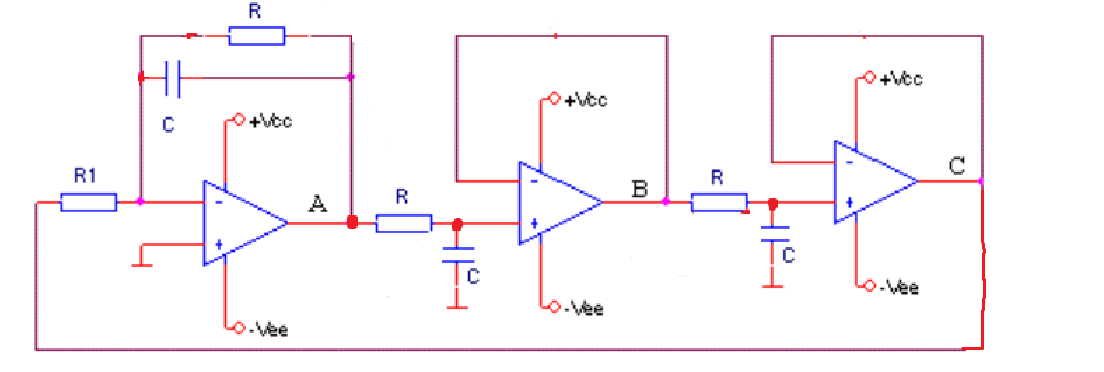
Here is my task:
In oscillator circuit ideal opamps are used, it is known R=10K, C=10nF.
a) Calculate frequency of oscillations,
b) If Vcc=Vee=15V, calculate amplitude of voltages in points A, B and C.
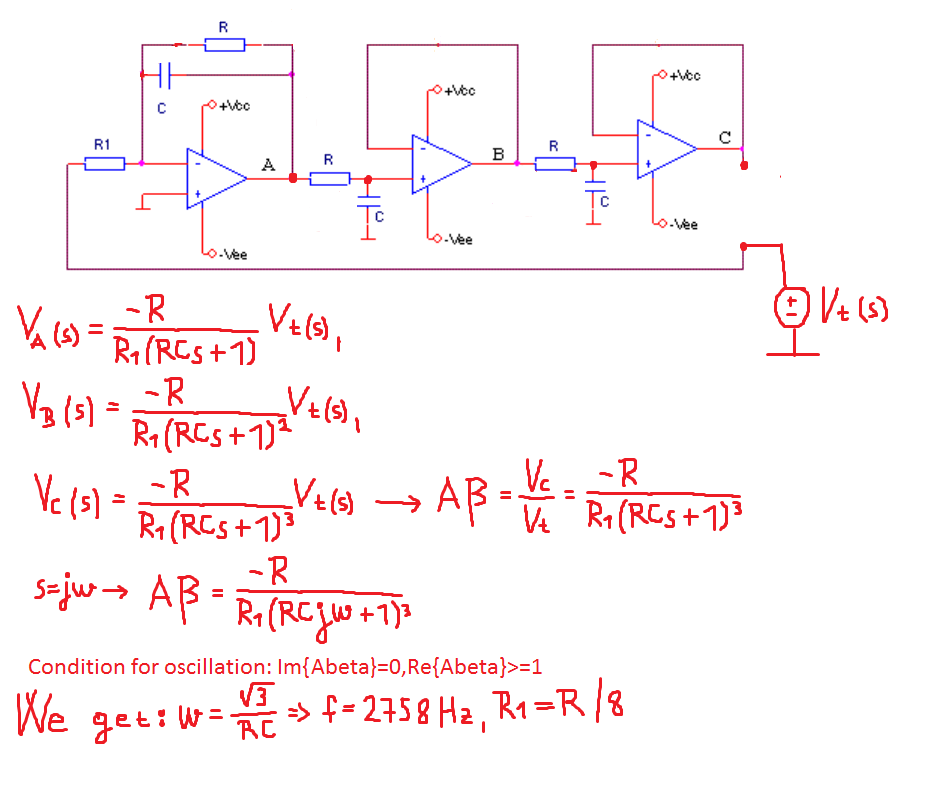
a) Here is my solution. I cut output loop, applied test generator and found loop gain on this way:
I cut loop on output, because they did same in this type of exercises. Is it general rule that to find loop gain Abeta, we cut loop on output? How to solve task b) ?
For oscillation to start, the condition is R1 < R/8.
Assuming you are just at this point, the oscillation will grow (exponentially) until the 1st (actually any one) opamp start clipping. At this point A will be just at +15 an -15 V as a sine wave (if the amplitude tried to go any higher, the amplifier would clip, and the effective gain would fall).
So, A is +/-15 sine wave; B is an opamp follower equal to this times the RC attenuation = 1/(1+jwCR); but wCR = sqrt(3), so you get an amplitude of 15/(1+j.sqrt(3)) = 7.5; C is this again == 3.75
Some supplementary remarks (to jp314`s answer):
Growing oscillation amplitudes will be limited (clipped) by the opamp stage with largest gain - and, in this case, it is the first opamp (DC gain is R/R1>8).
Select the ratio R/R1 only slightly larger than "8". Otherwise, clipping is too strong and will result in a bad THD at the output of the other stages.
Regarding your question: In principle, you can open the loop at any point within the loop. However, it is important to use a node where a small output resistance meets a much larger load resistance. Normally, this condition can be fulfilled at each opamp output node.
