Charging and discharging of capacitors

Question

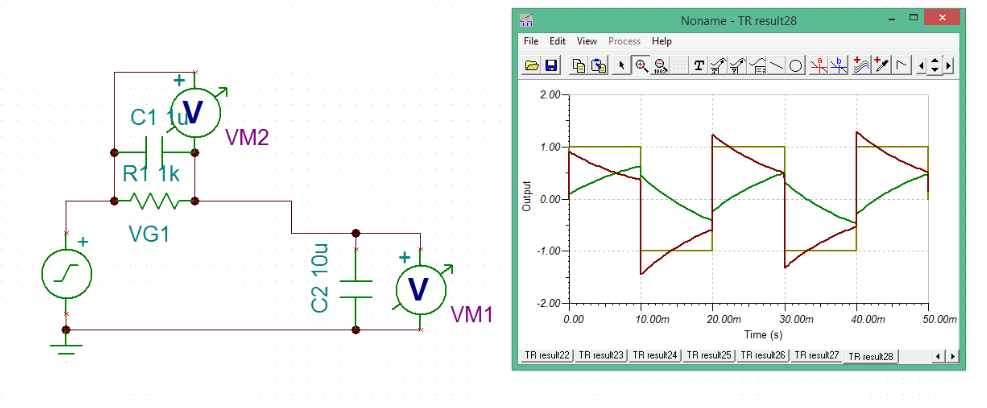
I simulated this circuit in TINA-TI. However I could not understand the output waveform for the negative half of the cycle.

Waveform: Green- Voltage across C2 (10 u cap) Brown- Voltage across C1 (1 u cap) Yello- the input square wave

For the first half at t=0, both the capacitors will charge instantaneously. Of course the capacitor with higher capacitance will charge to a lower voltage as compared to the smaller capacitor.

For t>0, C1 starts to discharge through the resistor R1 which means C2 must start charging so as to maintain an over all voltage of 1V at the input terminal.

However, in the negative half of the cycle the voltage for C2 remains positive and for C1 it drops to less than -1 volts. Why is the voltage of C2 not negative (similar to C1)? Why isn't voltage across C1 more than -1 volt and across C2 <0 such that VC1 + VC2 = -1 volt?

Answer 1

One way to model the voltage source's transition is:

\$ \Delta V_{C1} + \Delta V_{C2} = -2V \$, the combined change of C1 and C2 is a -2V swing.

Along with, \$ \Delta Q \$ for both C1 and C2 are the same. This is due to conservation of charge or current. Although the transition is instantaneous, making the current spike to be infinite which can be seen as a delta function. Also, there is zero time for any charge or current to go through the resistor.

\$ Q = CV \$, therefore \$ \Delta Q = C1 \times \Delta V_{C1} \$, similarly for C2.

\$ C1 \times \Delta V_{C1} = C2 \times \Delta V_{C2} \$, therefore the voltage change across the capacitor is inversely proportional to its capacitance.

For the circuit with C2 being 10 times that of C1, \$\Delta V_{C1}\$ is therefore 10 times that of \$\Delta V_{C2}\$. And it is easy enough to combine the top and bottom equations to solve for both \$\Delta V\$.

Now if you apply the small \$\Delta V_{C2}\$ to \$V_{C2}\$ (which is around 0.6V on the chart right before the first transition), it is not near enough to make it gone negative at the transition.