I have the following question: Since the current through the current source is not given (may be error), I assume it to be 2 A. Now since the resistors 6 Ω and 3 Ω are in parallel, I calculate the equivalent resistance and reduce the circuit.
to the following
But to do mesh analysis I need to know the internal resistance of the current source, right? So how can I do mesh analysis? Please advise.
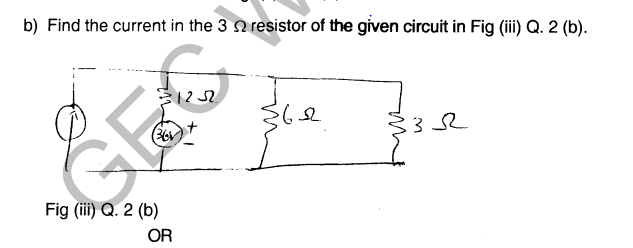
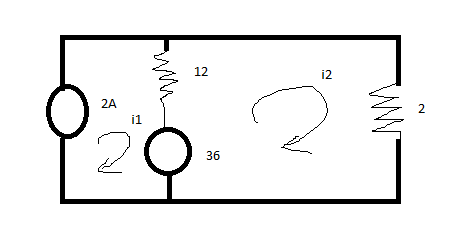
@elecman, there are multiple ways in solving this circuit. One is with Mesh Analysis and the other way is Nodal Analysis. I really wish there was a way you and I can chat while we work on this problem, but let's still solve this circuit for both ways.
FOR MESH ANALYSIS:
You've have simplified the circuit by combining the resistors in parallel. Resistors in parallel have the same voltage. So now once we get the voltage across the 2 ohms resistor, it will be the same voltage across the 3 ohms resistor. Then from Ohm's Law, we can calculate the current going through the 3 ohms resistor.
In order to get the voltage across the 2 ohms resistor, we need to get the current entering it. Let's call this current I2 (since it is in the second loop). Let's call the current in the first loop I1. By Looking at the circuit, I1 = 2A. So there is no need to do a mesh equation for the first loop since we know what I1 is. However, to find I2, let's do a mesh loop for loop 2:
The current going through the 12 ohms resistor can go up or down, depending on what current (between I1 and I2) is stronger, however since we want the current to go in the direction of I2, we will make this current I2 - I1.
-36v + 12 * (I2 - I1) + 2 * I2 = 0
From there you can solve for I2. With I2, you can get the voltage across the 2 ohms resistor which would be 2 * I2 (which is the same voltage across the 3 ohms resistor) and from there you should get the current going through the 3 ohms resistor which should be = (2 ohms * I2)/3 ohms.
FOR NODAL ANALYSIS:
All we have to do is find the voltage across the 3 ohms and from there get the current across the 3 ohms using Ohm's Law. There is no need to combine anything, just write your nodal equations. Let's call the voltage across the 3 ohms resistor, V3. So assuming currents that are entering the top V3 node are -ve, while currents leaving the top node are +ve, we have
-2amps + (V3 - 36v)/12 + V3/6 + V3/3 = 0.
From there you can find V3, and use V3/3 to get the current going through the 3 ohm resistor.
You have 2 unknowns, i1 and i2. Therefore you need 2 equations to calculate their value. One equation comes from KCL applied to the single node: the currents coming into the node must equal the currents leaving the node. This will give you an equation relating i1 and i2. Applying KVL to the right half of the circuit will give you another equation relating i1 and i2. Solving both equations, simultaneously, will give you the values of i1 and i2 from which all other information about the circuit can be obtained.
