0
\$\begingroup\$

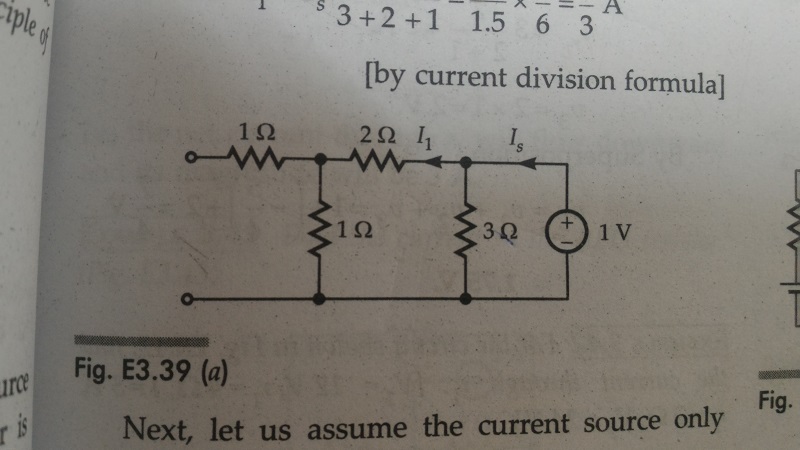
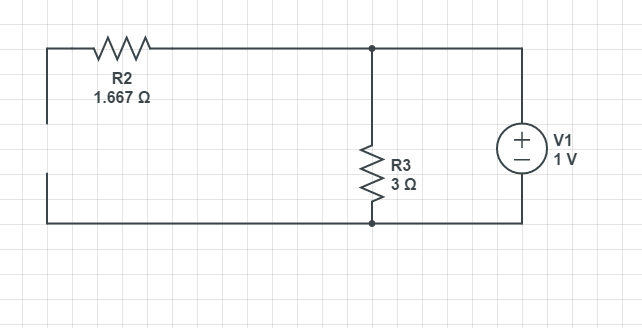
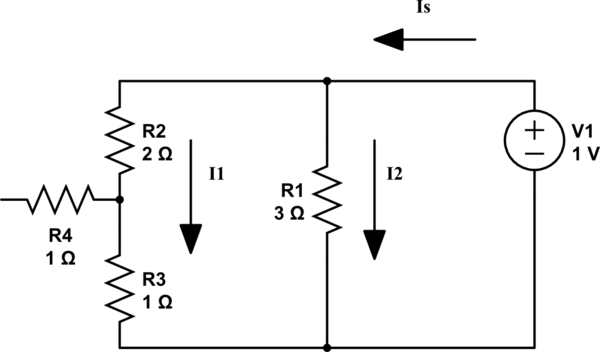
I have the following circuit:

enter image description here

I'm trying to calculate the equivalent current. In my approach resistors 2 and 1 are in parallel so their equivalent resistance would be 2/3 then since resistors 1 and 2/3 are in series I will get an equivalent resistance of 5/3

enter image description here

Now since the 2 resistances are in parallel I get the equivalent resistance as 1.06. Now the current in the circuit is \$V/R\$ so \$1/1.06=0.94\$. But the answer in the textbook says it's 0.66. Please let me know what I'm doing wrong here.

\$\endgroup\$

2 Answers 2

Reset to default
2
\$\begingroup\$

The left 1ohm resistor does not affect the circuit at all, you might as well remove it.

Then the remaining 1ohm and 2ohm resistors are in series making them effectively a single 3ohm resistor.

Then the two 3ohm resistors are in parallel, giving a single 1.5ohm resistor.

And finally 1V/1.5ohm is 0.67A.

\$\endgroup\$
12
  • \$\begingroup\$ Thanks..but what if i dont remove it? There is no rule that says i should remove it,right? \$\endgroup\$
    – elecman
    Commented May 2, 2016 at 7:30
  • \$\begingroup\$ You can also think about the open circuit as a resistor of infinite resistance. Then it is in series with the leftmost 1ohm resistor and together they form a single infinite-resistance resistor. Which in parallel with the other 1ohm resistor forms a 1ohm resistor. \$\endgroup\$
    – avakar
    Commented May 2, 2016 at 7:35
  • \$\begingroup\$ Basically that resistor just dangling off in space, so it doesn't have a path for current to flow through it. (As avakar said, the resistance between those open terminals is infinite.) Now, if something were connected to the circuit between those terminals, things would be different! \$\endgroup\$
    – JohnSpeeks
    Commented May 2, 2016 at 7:37
  • \$\begingroup\$ @JohnSpeeks Well then if you think like that the final resistance 1.5ohm will be left in space so we can ignore it?? \$\endgroup\$
    – elecman
    Commented May 2, 2016 at 8:34
  • \$\begingroup\$ No, the final resistance forms a complete circuit. You can draw a path starting at the voltage source, through any of the other resistors, and back to the voltage source again. Not so for the dangling one! \$\endgroup\$
    – JohnSpeeks
    Commented May 2, 2016 at 8:38
1
\$\begingroup\$

schematic

simulate this circuit – Schematic created using CircuitLab $$I_s = I_1 + I_2$$ $$I_2 = \frac{V_1}{R_1} = \frac{1}{3}A$$ $$I_1 = \frac{V_1}{(R_2+R_3)} = \frac{1}{(2+1)}A = \frac{1}{3}A$$ $$I_s = \frac{1}{3} + \frac{1}{3} = \frac{2}{3}A$$ The R4 does not contribute anything as it has no path.

\$\endgroup\$
6
  • \$\begingroup\$ Thanks... Since 1 ohm does not contribute to anything if we remove 1ohm and see the resultant circuit,why we say that 1ohm and 2 ohm are in series and the resulting resistor and the 3 ohm is in parallel. \$\endgroup\$
    – elecman
    Commented May 3, 2016 at 9:35
  • \$\begingroup\$ Exactly, and most important is that this is a current divider. \$\endgroup\$
    – crowie
    Commented May 3, 2016 at 9:46
  • \$\begingroup\$ How is this circuit reduction possible imgur.com/x4QiEih ? Isnt 2 ohm and 3 ohm in series? \$\endgroup\$
    – elecman
    Commented May 3, 2016 at 9:55
  • \$\begingroup\$ Because the 3ohm is shorted out or bypassed by the wire in parallel, btw don't forget to vote me up if you like my answers. \$\endgroup\$
    – crowie
    Commented May 3, 2016 at 10:01
  • \$\begingroup\$ one more question please.. To use current division the incoming current should not have a resistor in between right ?But in this case imgur.com/x4QiEih 1A first passes through 1 ohm resistor before entering the //circuit. \$\endgroup\$
    – elecman
    Commented May 3, 2016 at 10:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.