So two resistors in series can be a voltage divider. So say I have the following circuit -
From what I have read the voltage gets split across the resistors based on their resistance. So there will be 2V across R1 and 8V across R2. Now I have also read that Vout takes the value of the voltage across R1, in this case, 2V. What I am not sure of is why it takes the value of the voltage across R1 and not R2?
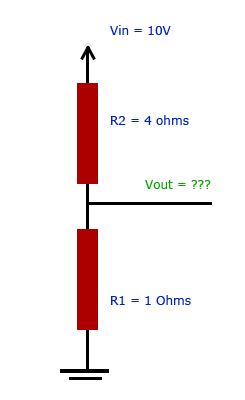
It does not "take" a value. It IS the value at the point you connect to.
\$V\$out, and the bottom of R2, and the top of R1 are all connected.
They are therefore necessarily all at the same voltage.
But R1 & R2 are "comfortable with this" - the point of the calculations that have been done was to find the voltage at which this condition was true.
With 8 V across R2 if you start at 10 V and come "down" R2 to the bottom you get 10 - 8 = 2 V.
With 2 V across R1, if you start at ground and travel "up" R1 you get 0 + 2 V = 2 V at the top.
i.e., starting at top or bottom you conclude that the voltage where R1 and R2 join is 2 V. You would be concerned if you did not get the same answer whatever you did as the whole point in working out the voltage across each is to work out what is needed for them to be "in equilibrium" when connected.
Advice: So far you are missing the intuitive feel for what Ohms law is about. What seems very hard will one day suddenly become blindingly obvious (really) and will make sense from then on. So - play with resistors on paper. Redraw Ohms law 3 ways* and make sense of each. Understand what it means. Then one day ... . :-)
$$R = \frac{V}{I}$$ $$I = \frac{V}{R}$$ $$V = IR$$
What seems puzzling and hard and a=obscure MUST become obvious and simple and trivial. Strange as it may seem, this can and will happen if you continue t play with the subject. All these forms must and in time will make COMPLETE sense to you. They must seem so trivially obvious that there can be no doubt that this is how it should be (even though in the real world there are small variations due to the special magics that indwells the stuff the world is made of).
Once you have grasped Ohm's law you can try Resistive power dissipation.
For P = power.
$$P = \frac{V^2}{R}$$ $$P = I^2 . R$$ $$P = V . I$$
All these are exactly equivalent. Clue - plug in the value for one of the variables from Ohm's law, cancel out what can be cancelled and you will arrive at another form of the statement.
For Example: $$P = \frac{V^2}{R} = V . \frac{V}{R}$$
BUT Ohm's law say V = IR
so P becomes
$$P = \frac{I. R . I . R}{R}$$
$$P = \frac{I^2R^2}{R} = I^2 R$$ Same as above.
Voltage is relative, you always specify the voltage at one point with respect to another point. The current through R2 will cause an 8V drop from the 10V power supply. If we would have taken the 10V as our reference the output would be at -8V. Here the lower end of R1 is the reference, as indicated by the ground symbol. Ground is zero volt, and all voltages in the circuit are referenced to that. So the higher end of R1 is 2V above ground, so we simply say its voltage is +2V. And that's your output.
Voltages exist only between two points. When a voltage is 'taken' at a single point, this is just short-hand for measuring relative to a reference point. The convention is to refer to that reference point as 'ground'.
In your diagram, it is the bottom end of R1 that is grounded. Therefore, it is fair to say that the voltage at Vout is the voltage across R1.
You have 2A flowing through the two resistors, so you have 2V across R1 and 8V across R2.
