Thanks for adding some initial thoughts to your question.
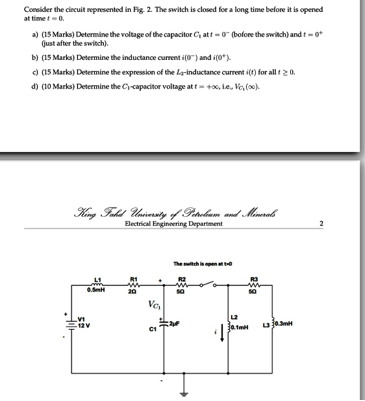
Let's start with (d), since that's what I brought up and it's easy. The switch is opened at \$t=0\$ and then you are asked what the voltage across \$C_1\$ is at \$t=+\infty\$. At this time, there is no more current into or out of \$C_1\$, the drop across \$R_1\$ will be \$0V\$ and \$L_1\$ will also have \$0V\$ across it because \$\frac{dI}{dt} = 0\$ after all that time. So \$V_1\$ must equal the voltage across \$C_1\$, so you are right, as \$V_c\left(t=+\infty\right) = 12V\$.
Back to (a). At this point, the switch is engaged for a very long time, as well. So again, \$\frac{dI}{dt} = 0\$ and \$\frac{dV}{dt} = 0\$ everywhere. This means no voltage drops across inductors, so they are all effectively dead shorts and you can replace them with wires; and no current through capacitors, so they are all effectively open circuits and you can mentally remove them. \$L_2\$ and \$L_3\$ now bypass \$R_3\$. This leaves just \$R_1\$ in series with \$R_2\$, across \$V_1\$, forming a voltage divider. The capacitor voltage will then be \$V_c\left(t=0^-\right) = 12V\cdot\frac{5\Omega}{5\Omega+2\Omega} = \frac{60}{7}\Omega = 8.57142857\Omega\$.
Now to (b). The inductance current doesn't undergo instantaneous change. So \$I_L\left(t=0^+\right) = I_L\left(t=0^-\right) + dI_L\$. But \$dI_L = \frac{dV\cdot dt}{L}\$ and a pair of multiplied infinitesimal values isn't just infinitesimally small, in this context. It's \$0A\$. So there is \$I_L\left(t=0^+\right) = I_L\left(t=0^-\right) = 0A\$.
Finally to (c). The current through \$L_2\$ at \$t=0^-\$ is indeed \$\frac{12V}{2\Omega+5\Omega} = \frac{12}{7}A\$. The voltage across \$L_2\$ is \$0V\$, so no current is flowing through \$R_3\$ and also so no current is flowing through \$L_3\$. So \$I_{L_2}\left(t=0^-\right) = \frac{12}{7}A\$ and \$I_{L_3}\left(t=0^-\right) = 0A\$ and \$I_{R_3}\left(t=0^-\right) = 0A\$.
However, when the switch is opened at \$t=0\$, then the current through \$L_2\$ must instantly now continue through the only remaining path, which is through \$L_3\$ and then \$R_3\$. But this is impossible, as it would require an infinite voltage to attempt such an infinitely fast change in current in \$L_3\$. The equation would be something like, \$\vert dV\vert = L_3\cdot\vert\frac{0A - \frac{12}{7}A}{dt}\vert\$, but given that \$dt\$ is infinitely small when moving from \$t=0^-\$ to \$t=0^+\$, the result is that \$dV\$ is infinitely large.
It's not possible to write an accurate equation for \$I_{L_2}\left(t\right)\$.
Note: One caveat in all of this that I need to add. I'm a hobbyist and I have never had so much as a single course of training in electronics or electricity. So I will defer to any professional disagreeing with me about the above.