I find some text about the winding of a toroid core as below:
But what's the math and physics behind these?
BTW: Can someone suggest some good books about the design of an inductor/transformer not only in theory but also about "how to make them by hand".
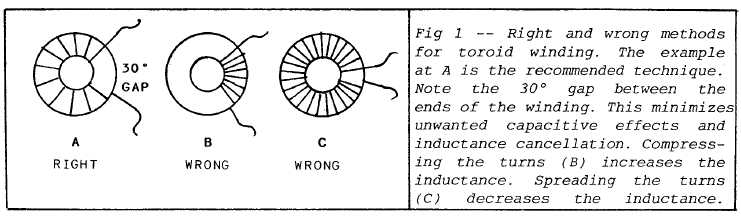
These winding instructions give you a single-layer inductor that minimizes parasitic effects of capacitance. The (B) version suffers from capacitance from one turn to its neighbours. The (C) version minimizes neighbour-capacitance but suffers from capacitance from end-to-end. The (A) version compromises between (B) & (C). Minimizing capacitance increases self-resonant frequency (important where the inductor is used in wide-bandwidth circuits).
For toroids used in high-Q tuned circuits, core relative permeability is often quite low (perhaps a factor of ten higher than air). So not every turn is linked to all other turns as it would for high-permeability cores. Although the rule where inductance is proportional to turns^2 is often applied, turns couple more tightly when bunched together, and couple less tightly when spread. In circuit simulators, the coupling factor (k) is certainly less than one, and is often less than 0.5 for low-permeability cores.
The math is not worth the effort. Besides, tolerance on toroid core permeability is notoriously poor.
