A transformer is basically two coils of wire (inductors) sharing a common core. If you don't connect a load to the secondary you might as well regard a transformer as an inductor. That inductor has (obviously) inductance and the current it takes is dependent on the applied voltage, frequency and inductance. So, usually, the number of turns on an AC power transformer are quite high and in the region of a thousand turns.
This means a few henries of inductance and possibly around 100 mA RMS current taken. This is just the primary with no secondary current being taken. This is typical of a transformer with a VA rating around 30 VA and will vary for different transformers in different applications i.e. it's just a rough guide to give a feel for the numbers involved.
This current is called the magnetization current and is the major source of transformer core saturation problems. It remains ever-present irrespective of what current you take from the secondary but, of course, it is added-to by the primary current caused when connecting a load to the secondary.
So, for a simple (and otherwise perfect) 1:1 transformer taking 0.1 A magnetization current and with a resistive load current of 1 A on the secondary, the total primary current comprises the 0.1 A magnetization current and the 1 A load current.
Given that the load current is resistive (as stated) and the magnetiztion current is due to the primary inductance, the two currents are 90 degrees out of phase hence the total primary current is \$\sqrt{1^2+0.1^2}\$ = 1.005 A.
For a 10:1 step-down transformer with 10 A on the secondary, it's exactly the same primary current.
Complicating things a bit; the magnetization current won't be particularly sinusoidal because iron/steel etc does not have a linear relationship between applied field (ampere turns) and flux density (teslas). That ratio is the permeability of the core material \$\mu\$. Also, the relationship has hysteresis and this gives rise to a resistive loss (called unsurprisingly hysteresis loss) so now there is an extra current present in the primary (irrespective of secondary load current seen by the primary).
Because the steel/iron core is a conductor it can act like a shorted turn therefore laminates are used that are insulated from each other thus, you only get small eddy currents in each laminate. These small currents flowing through the iron/steel generate heat and this is another loss that has nothing to do with load current. So, in summary the currents in the primary are: -
- Magnetization (reactive and not a loss)
- Hysteresis loss current (resistive)
- Eddy current losses (resistive)
- Load current
NB - hysteresis and eddy current loss is sometimes grouped under the term "iron loss".
But there's also leakage inductance and winding resistance to consider. Any current flowing in the secondary or primary flows through copper but it still has resistance and there will be a small volt drop and \$I^2R\$ power (copper) loss. Also, all the turns in the primary do not 100% magnetically couple to all the turns in the secondary so, in effect, there are leakage inductances which (like copper loss) reduce the output voltage on the secondary under load conditions.
It all boils down to the equivalent circuit of a transformer: -
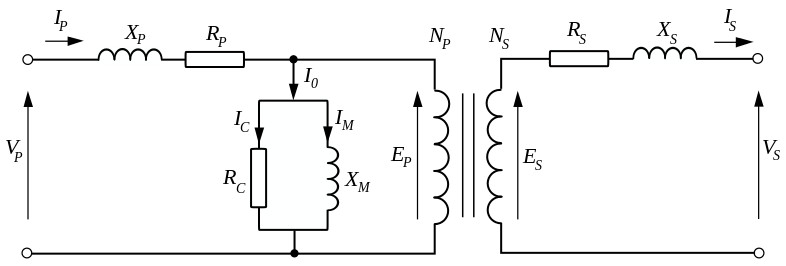
\$X_P\$ and \$X_S\$ are the leakage inductances i.e. those turns that don't couple. \$R_P\$ and \$R_S\$ are the copper losses of primary and secondary. \$R_C\$ represents the core loss (eddy current and hysteresis losses) and \$X_M\$ is the magnetization inductance.
What remains is a perfect lossless transformer represented by the transformer symbol in the picture; it has perfect characteristics and transfers power 100% efficiently - all the components hung aroud it turn that perfect transformer into the everyday imperfect transformer we use.

