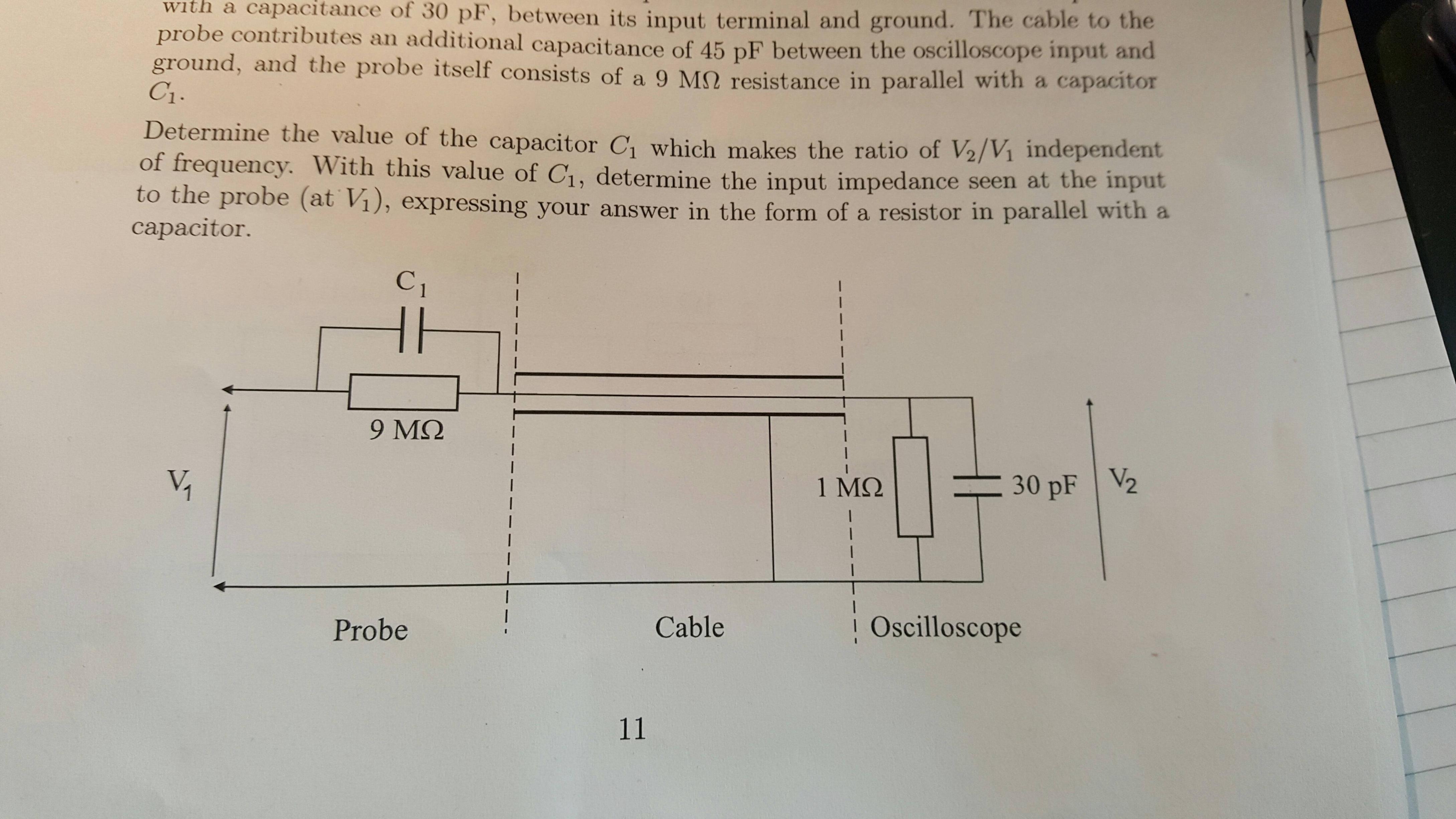
I am just starting out looking at more complex circuit diagrams, and I really do not understand what is being shown here
My initial guess was that this was a series circuit with the probe (consisting of a parallel combination), cable (consisting of a single capacitor) and oscilloscope (consisting of a parallel conbinatiin) all in series, however trying this has led to a very messy equation, so I'm not so sure I got it right. The depiction of the cable is very confusing to me.
Yes, it's showing a "10x" oscilloscope probe and the input it is connected to.
I imagine the point is to explain how a 10x scope probe works. This includes not just the 10x attenuation at DC, but also how the capacitors cause the same attenuation at high frequencies. C1 is the probe "compenstation" capacitor. This diagram sets things up to explain what it's there for and how it works.
The reason for showing the cable is probably to point out that there are unpredictable things going on. You don't know the capacitance of the cable, for example. The text probably explains how C1 should be computed, but I expect also then explains why you can't know it exactly up front and it needs to be quite accurate, and that this is therefore implemented as a trim cap that the user has to set.
I think you identified the parts correctly. Considering the messy equation, have you contracted the cable capacitance and the input capacitance of the oscilloscope into a single value? This might simplify your calculation a bit (cable and input capacitor are in parallel).
However, you will get a fraction with \$j\omega\$ as well as some non-frequency dependent terms in the numerator and denominator. This is to be expected. Your goal is to select the capacitor, so both terms are the same (apart from a real valued factor), so the result becomes independent of frequency.
