Calculation of the input impedance of reactance modulator

Question

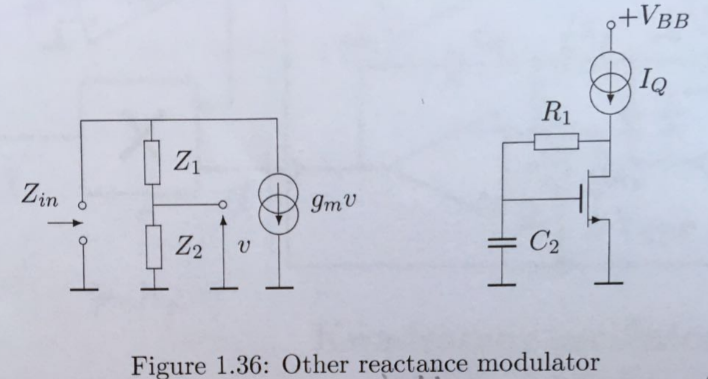
The figure 1.36 is a circuit of reactance modulator which is equivalent to a tunable inductance. I know the input impedance expression is Zin = (Z1 + Z2)/(1 + gm*Z2), but how to derive this expression?

Answer 1

Well $$Zin = \frac{Vx}{Ix} $$

where Vx is a input voltage and

$$ Ix = \frac{Vx}{Z1+Z2} + gm*v $$ additional \$ v =Vx*\frac{Z2}{Z1+Z2}\$

So we have this

$$ Ix = \frac{Vx}{Z1+Z2} + \frac{gm*Vx*Z2}{Z1+Z2} $$

$$ Ix = Vx(\frac{1}{Z1+Z2} + \frac{gm*Z2}{Z1+Z2}) $$

$$ Ix = Vx(\frac{1+gm*Z2}{Z1+Z2}) $$

And finally

$$ Zin = \frac{Vx}{Ix}=\frac{Z1+Z2}{1+gm*Z2} $$

Do you see now how we derive this expression?

Answer 2

This could be taken further to find the equivalent reactance needed in the circuit from the left hand side of the given diagram. It is assumed the active device is a transistor Take: $$ I_T = V_T \left\lbrack \frac{1 + g_m Z_2}{Z_1 + Z_2} \right\rbrack $$

Divide both sides by \$ V_T \$ to find the total admittance of the circuit: $$ \frac{I_T}{V_T} = Y_{in} = \frac{1}{Z_1 + Z_2} + \frac{g_m Z_2}{Z_1 + Z_2} = \frac{1}{Z_{in}} $$ Now divide numerator and denominator of the second expression of \$ Y_{in}\$ (the admittance of the transistor) by \$ g_m Z_2 \$:

$$ Y_{in} = \frac{1}{Z_1 + Z_2} + \frac{1}{ \frac{1}{g_m} + \frac{Z_1}{g_m Z_2}} $$

We are interested in the admittance of the active device. The quantity: $$ jX_{eq} = \frac{Z_1}{g_m Z_2} $$ is the needed equivalent reactive impedance.

An example would be for \$ Z_1 = j \omega L \$ and \$ Z_2 = R \$: The reactance becomes: $$ X_{eq} = \omega \left\lbrack \frac{L}{g_m R} \right\rbrack $$ Notice \$ X_{eq} \$ is positive and \$ \omega \$, the angular frequency, is in the numerator making an equivalent inductance. Thus: $$ Y_{transistor} = \frac{1}{ \frac{1}{g_m} + j \omega L_{eq}} $$ This makes the equivalent inductance equal to:

$$ L{eq} = \frac{L}{g_m R} $$ The impedance of the transistor is $$ Z_{transistor} = \frac{1}{Y_{transistor}} = \frac{1}{g_m} + j\left\lbrack \frac{\omega L }{g_m R} \right\rbrack $$ Let $$ \omega L >> R $$

Then $$ Z_{transistor} \approx \frac{j \omega L}{g_m R} $$

where $$ L{eq} = \frac{L}{g_m R} $$

.