AC Circuit Having Only Capacitor

Question

In my book, it is written that at any instant,

PD across the Capacitor Plates = Applied EMF ---------(1)

Assuming that it's a pure Capacitor of Capacitance C, I think if Voltage across Capacitor becomes equal to the Applied EMF then, it means that the capacitor is fully charged and thus, no current will flow.

Also, how can it be true that the given statement (1) is true at any instant?

As per the Phasor Relationship between Applied EMF and Current in Circuit, the Current is 0 at π/2 and at odd multiples of π/2 where the Applied EMF corresponds to its Peak Value.So Capacitor becomes fully charged at these instances.

Thus, what i think:

PD across Capacitor Plates = Peak Value of Applied EMF

Where am I wrong?

Answer 1

Where am I wrong?

I'm sorry to tell you you're wrong end-to-end. You're missing the whole point of the analysis (the wording of the book doesn't help either).

"P.D. across the Capacitor Plates = Applied EMF" is just a fancy (and confusing for any newbie) way to say "Let's apply an AC voltage to the capacitor and see what happens then with other magnitudes like current through it". I.e.:

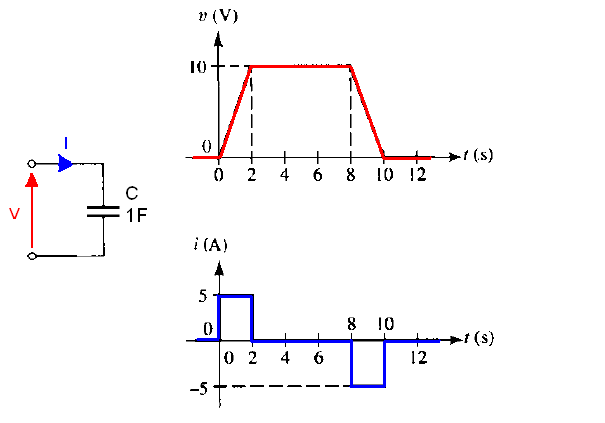
^{simulate this circuit – Schematic created using CircuitLab}

Putting things this way makes easy to answer this question of you:

[...], how can it be true that the given statement (1) is true at any instant?

Well, it's true because we're forcing it to be like that so we can see what happens then with the current through the capacitor.

Next step is finding how V and I are related. One could expect that if we force the potential between the capacitor plates to vary with time, then the current through the capacitor will somehow a similar behaviour.

We recall, as does the book, that:

$$ Q=CV $$

and

$$ I=\dfrac{dQ}{dt} $$

So with the help of a little math, finally we arrive at:

$$ I={\omega}{C}{\xi_0}\sin({\omega}t+\frac{\pi}2) $$

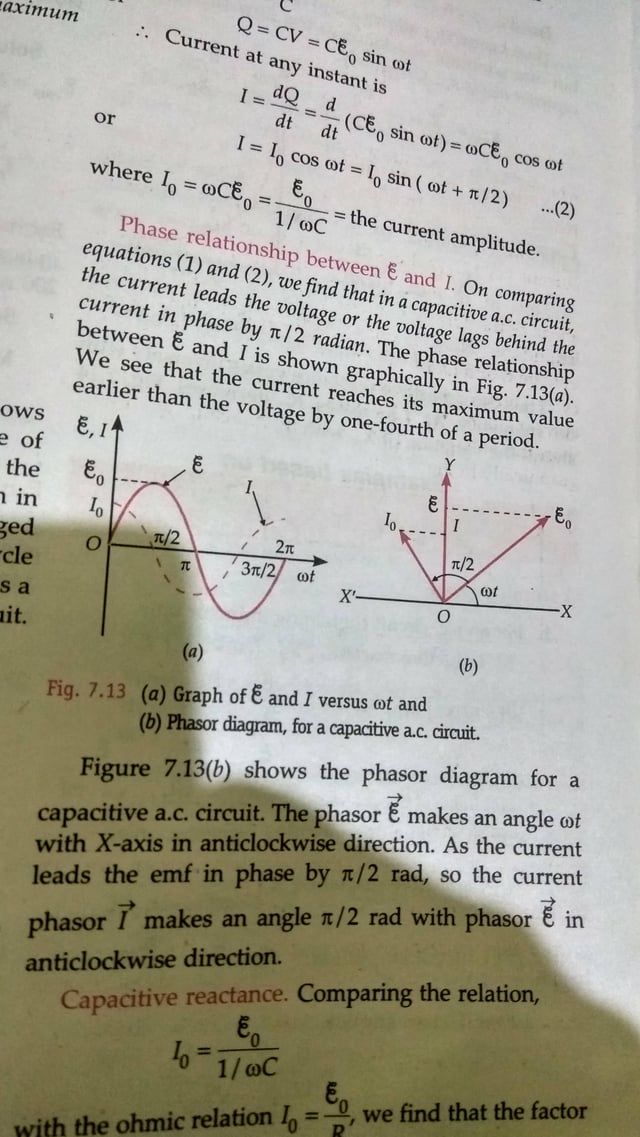
Which, in a mathematical sense, means that current is also sinusoidal and that there is a \$\dfrac{\pi}2\$ phase difference between V and I.

However, what does it mean in a physical sense? Well, somehow your intuition about it wasn't bad after all:

the Current is 0 at π/2 and at odd multiples of π/2 where the Applied Emf corresponds to its Peak Value.So Capacitor becomes fully charged at these instances

That's it! When the capacitor is fully charged, no current flows to it. When it's fully discharged, maximum current flows to it in order to charge it. And the capacitor oscillates through those states all the time while we keep applying an AC voltage to it.

EDIT:

After reading your comment to my answer, I understand where your problem is: your mathematical approach is flawed.

You look at the instant value of \$V\$ and think than you can take an interval of time \${\Delta}t\$ small enough so \$V\$ can be considered constant, that is, \${\Delta}V{\approx}0\$. Then you assimilate this situation with DC (which is also wrong because in DC you charge the capacitor through a resistor, which is absent here) and deduce that if \${\Delta}V=0\$ then \$I=0\$ so no current flows. You then extrapolate that deduction to every possible \$t\$ and conclude that no current flows at all and that the capacitor must be fully charged at all times at the peak value of the applied EMF.

Well, this is mathematically wrong for a number of reasons:

1. If you're dealing with intervals, then apply them to ALL magnitudes involved. Your flaw resides in considering \$I=0\$ when you should be considering \${\Delta}I=0\$ instead (which isn't true either, continue reading to see why).

2. When looking at what happens around any arbitrary time \$t_1\$, your \${\Delta}t\$ is an increment to that \$t_1\$. The same thing goes for \$V\$ and \$I\$: your \${\Delta}V\$ will be an increment to \$V_1=V(t_1)\$, and \${\Delta}I\$ will be an increment to \$I_1=I(t_1)\$. Think of \$V_1\$ and \$I_1\$ as initial conditions at the start of interval \${\Delta}t\$. It's wrong to assume \$V_1\$ and \$I_1\$ are equal to zero. Also, it's wrong to think about \${\Delta}V\$ as the difference between the applied EMF and the voltage at the capacitor. As it has been said, there is no difference between the applied EMF and the voltage at the capacitor, it's just forced to be equal.

3. For very small \${\Delta}t\$ intervals, you'll have \${\Delta}V{\approx}0\$ and \${\Delta}I{\approx}0\$. But that doesn't mean at all that the concatenation of time intervals where \${\Delta}I{\approx}0\$ will yield \$I=0\$ and from there conclude that "no current flows, so the capacitor must be charged and \$V\$ must be constant". It's wrong to think like that. Differential Calculus and Infinitesimal Calculus tells us how to deal with things when \${\Delta}t{\rightarrow}0\$. And someone smarter than you and me already used them to work it up for us to build upon it:

$$ I=\dfrac{dQ}{dt} $$

Answer 2

Current in the capacitor is proportional the rate of voltage change across it (proportional to how quickly the voltage across the capacitor is changing). The faster the voltage change (frequency of an AC signal is high) the large the current flow through the capacitor.

$$I = C*\frac{dV}{dt} $$

This means that to sustain current through a capacitor the applied voltage must change. The more rapidly voltage changes the larger the current. On the other hand, if the voltage is kept constant no current will flow no matter how large the voltage. Likewise, if the current through a capacitor is found to be zero, this means that the voltage across it must be constant, not necessarily zero.

The special case is when the voltage input voltage is a sine-wave. For the sine-wave, the rate of voltage change (\$\frac{dV}{dt}\$) reaches the maximum when voltage signal crossing zero. And the rate of voltage change reaches minimum when the voltage is at a peak value.

This is why current reaches \$0A\$ because at peak the rate of change is \$\frac{dV}{dt} = 0\$ and when a input sine-wave crossing 0, the rate of change is at max \$\frac{dV}{dt} = A_{peak}2\pi f \$.

For example for \$A=1V\$ and \$f = 50Hz;C=1\mu F\$

The highest slope of the signal is \$\frac{dV}{dt} = A_{peak}2\pi f = 1V*6.28*50Hz = 314V/s\$ therefore the capacitor peak current values is

\$I = C*\frac{dV}{dt} = 1\mu F * 314 \frac{V}{s} = 0.314mA\$

And this diagram is trying to shows the charging and discharging phases for a sine-wave input voltage.

All this analysis assumes steady-state situation (The circuit is ON for a very long time).

Answer 3

The P.D. = \$\xi(t)\$ is a definition so it is always true.

Voltage is assumed to be sinusoidal function of time (and steady-state), so physically something (such as a function generator output) is forcing that voltage across the capacitor to be \$\xi(t)\$ = \$\xi_0 \sin(\omega t)\$.

They derive the capacitor current as a function of that applied voltage.

Answer 4

here is how it goes. your 1st statement "P.D. across the Capacitor Plates = Applied EMF" is correct and your 2nd statement that"it means that the capacitor is fully charged " is also correct but your assumpmtion that" no current will flow" is not correct because you see in AC, voltage is incresing and decresing. charge will go into capacitor and come out of it hence current will flow eventually. hope it will help.