Generating a magnetic field with an electromagnet

Question

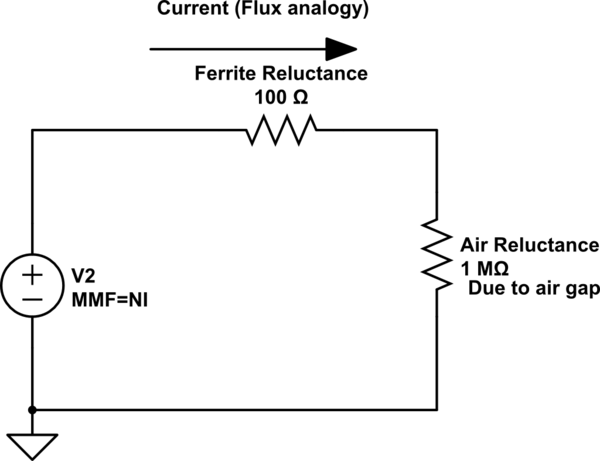
I want to generate an AC magnetic field. However, when trying to do this with a ferrite rod electromagnet, the measured field strength (via magnetometer) was much lower than I expected. I found out that the inductance of this electromagnet was also much lower than expected due to the large "air gap/path" of a rod's magnetic circuit:

Inductor core permeability much lower than expected

My primary goal however is not to achieve a certain inductance, but to generate a certain (AC) magnetic field strength with a coil loop and ferromagnetic core. I figured the steps would be:

1. Given a desired field strength \$B_{min}\$ at a specified distance \$d_{max}\$, determine the necessary dipole moment \$m\$.
2. Given the permeability of a ferromagnetic material \$\mu_r\$ and its saturation flux density \$B_{sat}\$, determine the minimum volume \$V_{min}\$ of the material to achieve the desired \$m\$.
3. Determine the necessary combination of turns \$N\$ and current \$I\$ to achieve the desired local \$B\$-field (and thus \$m\$) given the chosen volume \$V\$.

---

Solving these in order:

1. Let's say I need a source dipole moment of \$m = .075Am^2\$ to generate the desired field. This is half the strength of a small neodymium magnet: https://www.kjmagnetics.com/proddetail.asp?prod=ZD2.

2. To determine the minimum volume, let's say I want to bring the material to saturation (\$B_{loop,center} = B_{sat}\$)in order to achieve minimum volume. In other words, I want \$V = V_{min}\$, and I will assume that the magnetic field generated by the coil is uniform everywhere in this small volume.

   Assuming \$\mu_r\$ is large (e.g. >1000) and linear (even though this is usually not the case for ferromagnetic materials), we can say:

   \$B = \mu_0(H + M) = \mu_0(H + (\mu_r-1)H)\$

   \$\therefore B \approx \mu_0M\$

   And because \$M = \frac{m}{V}\$, we have \$V = \frac{\mu_0}{B_{sat}}m\$.

   For the 6.32mm diameter material with \$B_{sat} \approx .5T\$ referenced in my previous question, this results in a necessary volume of 377mm\$^3\$, or the rod with height 6mm.

3. Using the assumption of uniform \$B\$-field from the current loops to obtain a rough order of magnitude:

   \$B_{loop,center} = \mu_0\mu_r\frac{NI}{2R}\$

   Assume \$B_{loop,center} = B_{sat} = .5T\$, \$2R = 6.32\$mm, \$\mu_r = 2000\$ (type 77 ferrite)

   This results in \$NI = 1.257A\$. So for example, if \$N=100\$, then the necessary \$I\$ is 12.57mA.

However, despite having the electromagnet configuration described above, and at least a few mA of current, I measure an extremely weak field that essentially requires the coil to be right on top of the magnetometer to see anything. Is there something wrong with my reasoning here? Does it again have something to do with the air gap?

Answer 1

Probably the best way to understand what's going on is to use an electrical circuit analogy again.

The MMF is like a voltage source, and is the number of turns in your coil times the current in the coil.

The permeability of your core is like a resistor connected to your MMF source. It's high permeability so just like a low value resistor. You want a high magnetic flux, so your voltage source wants to cause a high current to flow in the resistor.

Now, if your magnetic core were a closed path like a toroid you would be all set.

However, you have a break in your magnetic circuit path. This is like an additional resistance in series with the small resistor you have from the ferrite core.

This "air gap" resistance analog is very much larger than your ferrite resistance analog. So no matter how high a permeability your ferrite has your MMF voltage source will not be able to drive a large current in it. (You won't get a large magnetic flux.) See the schematic below. The resistor values are arbitrary, just to illustrate the point.

So with a large air gap the only way to increase the magnetic flux is to increase the number of turns, N and/or the current in the coil.

^{simulate this circuit – Schematic created using CircuitLab}