Simple Capacitor charging and discharging question

Question

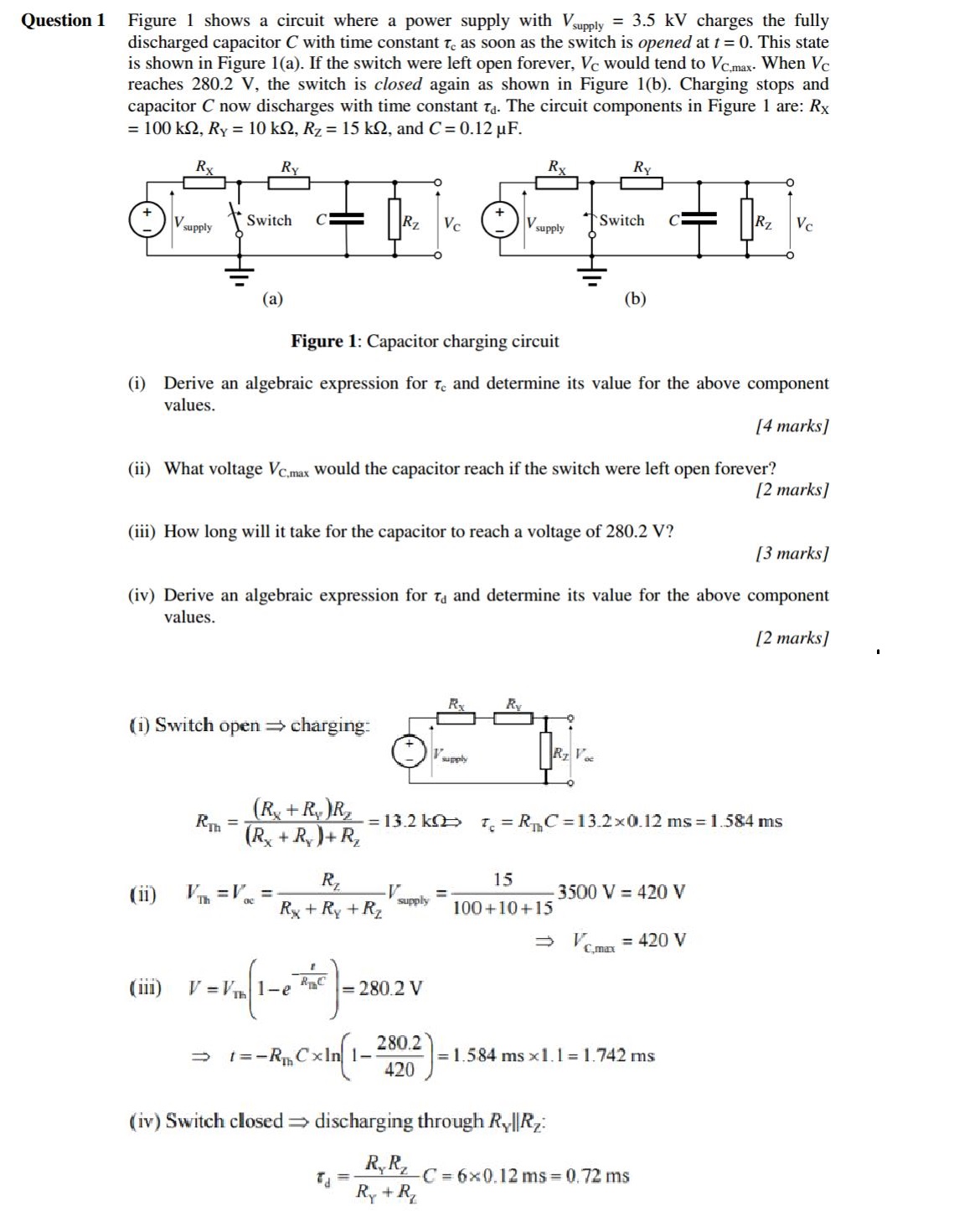
Below is a circuit consisting of a capacitor, voltage supply and resistors. There is a switch that lets the capacitor charge and discharge. I have also uploaded the given answers below;

I understand that for:

(i) Tc: you find the thevenin resistance and multiply it by the capacitor value.

(ii) Vc Max: the voltage across the parralel (Rz) resistor.

(iii): I am not sure that he has done there. Has he used the capacitor charge or discharge formula and rearranged it of some sort?

(iv): Not sure what hes done here?

.

Answer 1

I am not sure that he has done there. Has he used the capacitor charge or discharge formula and rearranged it of some sort?

The charging equation look like this:

$$V_C = V_{th}(1 - e^{\frac{-t}{RC}}) $$

Now to solve for the "time"\$(t)\$ we need to rearranged this formula.

First we can divide by \$V_{th}\$

$$\frac{V_C}{V_{th}} = 1 - e^{\frac{-t}{RC}} $$

We subtract 1 from both sides and multiply both sides by -1

$$\frac{V_C}{V_{th}} - 1 = - e^{\frac{-t}{RC}} $$

$$1-\frac{V_C}{V_{th}} = e^{\frac{-t}{RC}} $$

take the ln() of both sides

$$ln(1-\frac{V_C}{V_{th}}) = {\frac{-t}{RC}} $$

Multiply both sides by -RC

$$-RC*ln(1-\frac{V_C}{V_{th}}) = t $$

Swap sides

$$t = -RC*ln(1-\frac{V_C}{V_{th}})$$

(iv): Not sure what hes done here?

\$t_d\$ is a discharge time constant \$t_d = R*C \$

Where \$R\$ is a resistance seen by the capacitor when discharging.