0
\$\begingroup\$

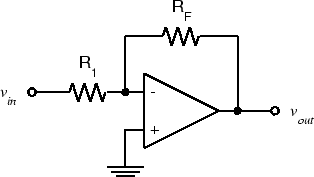
Below is a typical inverting op amp topology:

enter image description here

I was about to answer to this question, but I wasn't sure about the conclusion and couldn't find a supporting document so far.

Normally in tutorials they explain the input impedance of this topology simply as:

Rin = R1.

This is because the voltage at the inverting input is "approximately" zero, so one can say: I = (Vi - 0)/Rin . This looks like an approximation neglecting the effect of Rf.

I'm trying to derive the input impedance in terms of feedback equation to see the effect of Rf.

Calling "a" as the open loop gain.

Ir=If=I

Vo = -a*Vd

Vd = (Vi-I*R1-0)

Vo = Vi-I*(Rf+R1)

-a*(Vi-IR1) = Vi-I(Rf+R1)

Vi(1+a) = aIR1 + I*(Rf+R1)

Vi(1+a) = I(a*R1+R1+Rf)

Vi/I = (R1*(1+a) + Rf) / 1+a

Rin = Vi/I = R1 + (Rf / 1+a)

So is the equation Rin = R1 + (Rf / 1+a) correct?

\$\endgroup\$
10
  • 2
    \$\begingroup\$ No 'approximately' about it. Assuming a ideal opamp in any NFB configuration, the voltage at both inputs is the same, and as one input is grounded the other is a virtual earth, so Rin = R1. This is due to the effect of Rf, not 'neglecting' it. The correct equation is Rin+Rf/(1+a), and again assuming an ideal opamp a is infinite, so R1 = Rin. \$\endgroup\$
    – user207421
    Commented May 2, 2017 at 10:06
  • \$\begingroup\$ I think for Rin you wanted to write R1+Rf/(1+a) as in my question not Rin+Rf/(1+a) \$\endgroup\$
    – user16307
    Commented May 2, 2017 at 10:10
  • \$\begingroup\$ To add to that, high frequencies have a much lower gain so then "a" starts to become important but so does the phase shift change so, in short, I can't see the point of deriving a simplified formula. \$\endgroup\$
    – Andy aka
    Commented May 2, 2017 at 10:11
  • \$\begingroup\$ To be able to make the gain (of the complete circuit) Rf/R1, a needs to be large enough. You cannot make a gain of 100 when a is also 100. So the desired gain sets a requirement for a. If a is too low then Rf will be significant for Rin but since the overall gain will not be as designed, this is not a good choice. A needs to be large enough and that results in that Rf has a very small influence on Rin (as in your formula). \$\endgroup\$
    – Bimpelrekkie
    Commented May 2, 2017 at 10:11
  • \$\begingroup\$ @Andyaka Imagine R1 is zero. If one follows the common way which is Rin = R1 so he might think Rin is literally zero. But Rin actually would be close to Rf/(1+a) in that case is that correct? \$\endgroup\$
    – user16307
    Commented May 2, 2017 at 10:13

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.