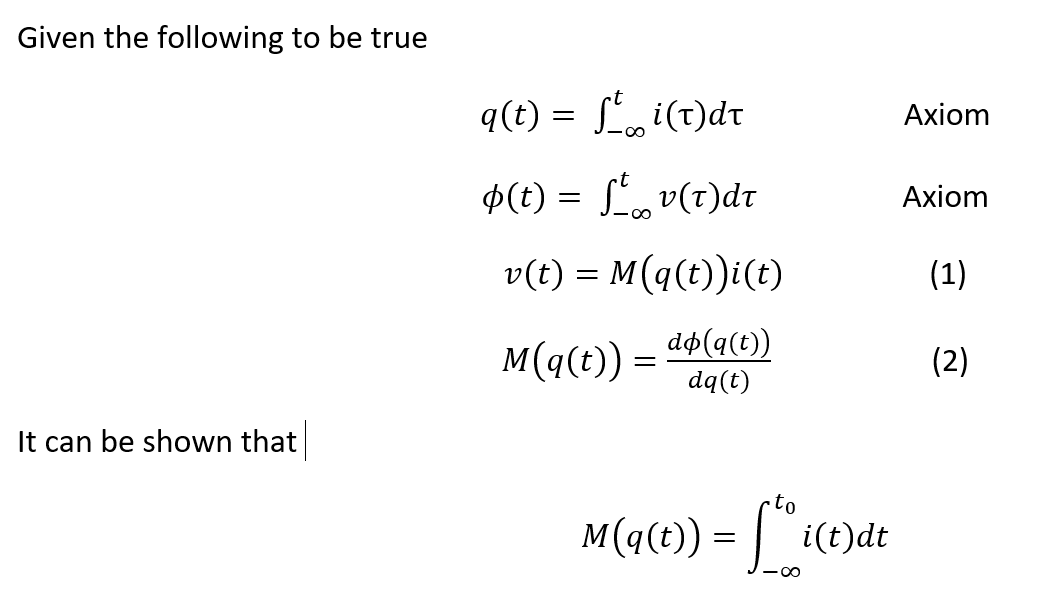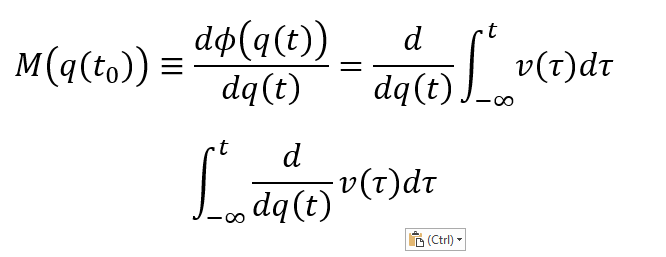3
\$\begingroup\$

I'm going through Chua's 1971 memristor paper. I am stuck on a part that I believe in pretty crucial. It is

Observe that the, value of the incremental memristance (memductance) at any time to depends upon the time integral of the memristor current (voltage) from t = - co to t= to. Hence, while the memristor behaves like an ordinary resistor at a given instant of time to, its resistance (conductance) depends on the complete past history of the memristor current (voltage). - Page 511(page 5 of the pdf) top of the right column

http://www.cpmt.org/scv/meetings/chua.pdf

I'm not seeing the integral from any of the equations 1 through 4 that are presented in the paper.

When I read the section in question, this is what comes to mind, but I cannot see the derivation. Am I thinking about this incorrectly?

enter image description here

To expand further on what I have tried

enter image description here

Does anyone see anything glaringly wrong with my math? Is it a physics thing possibly? I'm not seeing how current gets into this at all. I realize memristors is a rather newish topic that not many people are aware of or even care about.

\$\endgroup\$
2
  • \$\begingroup\$ You know, maybe the equation is supposed to be as I think. I cant find it anywhere on the net. \$\endgroup\$
    – Melendowski
    Commented May 28, 2017 at 2:13
  • \$\begingroup\$ Your question is not clear and has some flaws: (1) Where does the formula after "It can be shown that M(q(t))=..." come from? (2) That formula is obviously wrong: unit of M is resistance; but the unit of the integral is charge (current times time). (3) Also: please use integrated math folmula edititing (backslash dollar) instead if images of formulas if possible. \$\endgroup\$
    – Curd
    Commented Sep 4, 2017 at 10:23

1 Answer 1

Reset to default
1
\$\begingroup\$

I think that you have misunderstood the proposition of Chua: "Observe that the value of the incremental memristance (memductance) at any time \$t_0\$ depends upon the time integral of the memristor current (voltage) from \$t = - \infty \$ to \$t= t_0\$."

In fact the observation just says that \$M \$ is function of the integral of the current $$ M=M (q(t_0))=M\left ( \int_{-\infty}^{t_0}i (\tau)\mathrm d \tau\right) $$ and that $W $ is function of the integral of the voltage $$ W=W (\varphi(t_0))=W\left ( \int_{-\infty}^{t_0} v(\tau)\mathrm d \tau\right) $$

You have nothing to calculate.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.