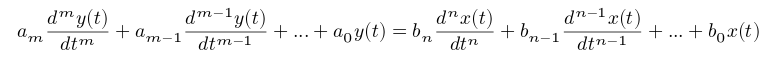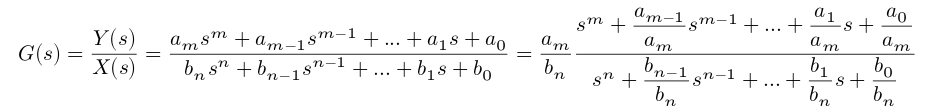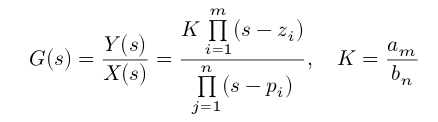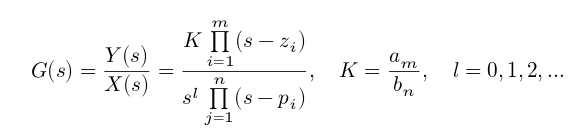A transfer function is derived from an ODE with the Laplace transform as following...
Some times though there is a different form of the transfer function, one that includes the system type number \$l\$.
How is that form derived? As I have listed the way we go from an ODE to a transfer function, how is the system type number came to be included in it? g
The type number is the number of pure integrators in the forward path, \$\small G(s)\$, of a closed loop system. This is equivalent to saying the number of 'free' \$ s\$ terms in the denominator of \$\small G(s)\$ . Alternatively, the number of poles of \$\small G(s)\$ at the origin.
It can easily be shown that the type number defines the steady state response of the closed loop system to various deterministic input signals. Thus a type 1 system will have zero SS error to a step input, a type 2 will have zero SS error to a ramp input; a type 3 to a parabola etc.
Hence if the CLTF is \$ \frac{G(s)}{1+G(s)H(s)}\$, then the type number is the number of denominator free \$s\$ terms in \$\small G(s)\$. Note, type number is not determined by the open loop TF, \$\small G(s)H(s)\$, unless the system is unity feedback.
