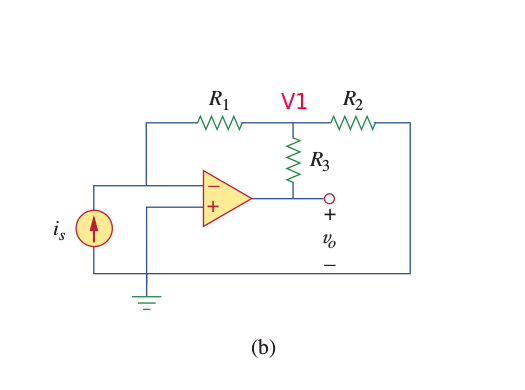
I need to show that for the above current to voltage converter,
\$\frac{V_0}{i_s} = -R_1(1+\frac{R_3}{R_1}+\frac{R_3}{R_2})\$
assuming that the op-amp is ideal,
Voltage at the negative input terminal = \$V_n\$
Current through the negative input terminal = \$i_n\$
Current through the positive input terminal = \$i_p\$
Voltage at the positive input terminal = \$V_p\$
\$V_p\$ = \$V_n = 0V\$
\$i_p = i_n = 0A\$
Using voltage divider rule,
\$V_1\$ = \$\frac{R_2}{R_2+R_3}V_0\$
\$i_s = \frac{0-V_1}{R_1}\$, So , using these two equations ,
\$\frac{V_0}{i_s} = -R_1(1+\frac{R3}{R2})\$
Why my answer is wrong ?
EDIT:
I think have figured out the error on my previous calculation . The voltage divider rule still works here like this.
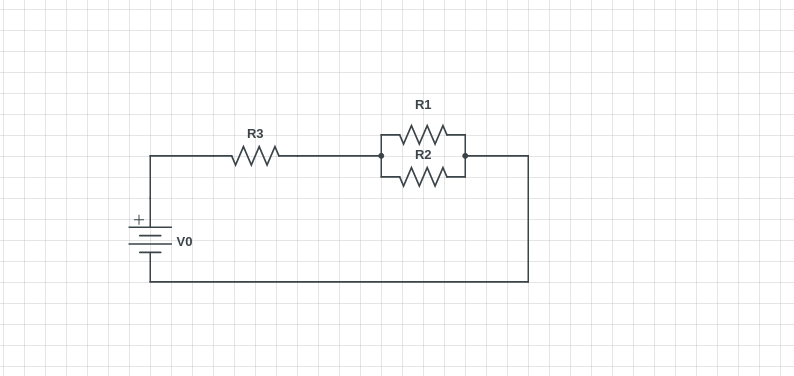
Let, \$R_p\$ be equivalent for R1 and R2
\$R_p = \frac{R_1R_2}{R_1+R_2}\$
\$V_1 = \frac{R_p}{R_p+R_3}V_0\$
\$I_s = \frac{0-V_1}{R_1}\$
\$I_s = \frac{0-\frac{\frac{R_1R_2}{R_1+R_2}}{\frac{R_1R_2}{R_1+R_2}+R_3}V_0}{R_1}\$
After solving this the proof comes.Is there any discrepancy in this?