An association of 4 resistors of 30ohms each, how can one assemble them so you get a resistance equivalent of 18ohms?
-
26\$\begingroup\$ Is this a riddle or a homework? \$\endgroup\$– Eugene Sh.Commented Oct 2, 2017 at 15:45
-
\$\begingroup\$ Definitely not my homework, but I was doing some exercices out of curiosity and stumbled upon this one and i'm definitely stuck. \$\endgroup\$– user35900Commented Oct 2, 2017 at 15:48
-
7\$\begingroup\$ there are not many combinations for 4 registers. Trying each one and calculate the resistance won't take much time \$\endgroup\$– phuclvCommented Oct 2, 2017 at 17:05
-
1\$\begingroup\$ @LưuVĩnhPhúc Actually it is not that simple, and mostly a guessing game. An example of not-that-educational exercise. \$\endgroup\$– Eugene Sh.Commented Oct 2, 2017 at 17:23
-
\$\begingroup\$ The answer by user43804 is by far the best. And don't VTC , this has useful answers and is on topic \$\endgroup\$– Voltage Spike ♦Commented Oct 2, 2017 at 19:46
7 Answers
Clearly to get 18 ohms, you need some of the resistors in parallel.
So, what do you need in parallel with a 30 ohm resistor to get 18? Answer: 45 ohms.
Now, you have a simpler problem: how to make 45 ohms from three 30 ohm resistors. That should be obvious!
Some of the earlier answers have already "given you a fish," but "teaching you how to fish" is more useful in the long term IMO.
-
1
-
39\$\begingroup\$ Or as Terry Pratchett(RIP) said: Give a man a fire and he's warm for a day, but set fire to him and he's warm for the rest of his life. \$\endgroup\$ Commented Oct 3, 2017 at 3:03
-
6\$\begingroup\$ @Namphibian I like 21st Maxim of Maximally Effective Mercenary more ;) "Give a man a fish, feed him for a day. Take his fish away and tell him he's lucky just to be alive, and he'll figure out how to catch another one for you to take tomorrow." \$\endgroup\$ Commented Oct 4, 2017 at 7:15
-
1\$\begingroup\$ On the theme of "teaching him how to fish", the correct answer is "I order an 18R resistor from the E24 range". It's good to be able to calculate resistances in parallel, but this exercise is not a good way to practise that. If your book likes exercises like this, I suggest your book isn't a very good teaching resource. \$\endgroup\$– GrahamCommented Oct 4, 2017 at 8:07
-
1\$\begingroup\$ @glglgl Agreed, but a guessing game of "how many ways can you link four resistors together?" is not the way to do it. \$\endgroup\$– GrahamCommented Oct 4, 2017 at 13:28
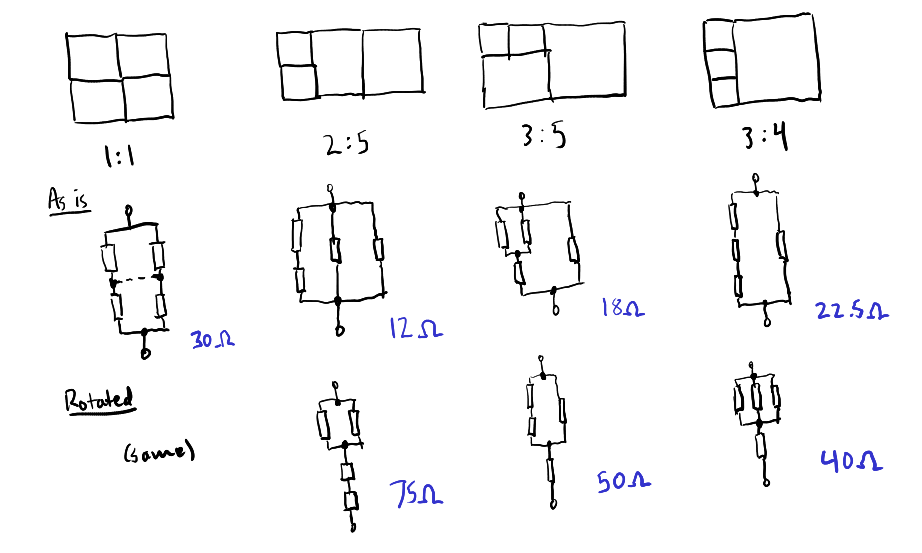
There is a technique I once found in a graph theory book by Béla Bollobás of all places. Imagine having a resistor network where the schematic can be draw without any crossings. Then for a given potential applied to the network, measure the potential and current for each component resistor. If you replace each resistor with a thin resistive plate V units tall and I units wide (V=IR so the aspect ratio is the resistance), the rectangles assemble into a single large rectangle whose aspect ratio is the equivalent resistance, and each junction shows up as a horizontal line.
This suggests a way to look for a resistor network: take four rectangles 30 units tall and 1 unit wide, and we want to find a way to scale the rectangles and assemble them into a rectangle that is 18 times taller than it is wide.
For easier visualization, since all the resistors are equal we can scale everything down vertically: the problem is to make a 18:30 (that is, 3:5) rectangle out of four squares. For illustration, I found I think every possible rectangle size and resistance you can make with these four resistors, but knowing 3:5 is the target size can speed up the search.
(Edit: I forgot 1:4, giving 7.5 and 120 Ohms.)
The equivalent resistance is 30 times the rectangle's aspect ratio. There's no need to do any sort of reduction of series/parallel circuits. An interesting bonus is that by rotating the rectangle, you get a circuit whose resistance is the reciprocal times 30 of the original circuit.
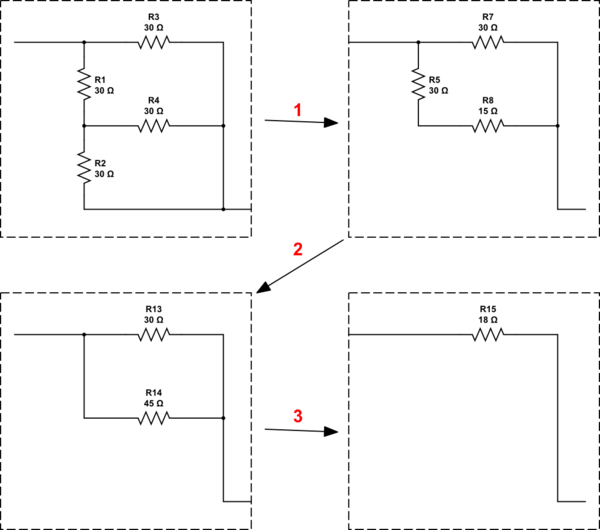
The rectangle method can be somewhat useful in the case of a network that can't be reduced using the series/parallel equations, though I think this one can be done by a delta-wye transformation:
-
\$\begingroup\$ That's a cool way to look at it :) \$\endgroup\$ Commented Oct 2, 2017 at 20:30
-
3\$\begingroup\$ And if you want a quick way to find the minimal network, here it is. \$\endgroup\$ Commented Oct 2, 2017 at 20:37
-
\$\begingroup\$ @MassimoOrtolano For when you are in that special electronics hell where all the bins in the resistor tray are labeled 1 Ohm. \$\endgroup\$ Commented Oct 2, 2017 at 20:57
-
\$\begingroup\$ Not 1 ohm, but quantum Hall resistance standards are all labeled 12.906... kohm ;-) \$\endgroup\$ Commented Oct 2, 2017 at 21:05
-
1\$\begingroup\$ It might be slightly less surprising that this was mentioned in a book by Bollobás once you take into account that he's a Fellow of Trinity College, Cambridge, and this technique was originally developed by four undergraduate members of the Trinity Mathematical Society. Brooks, Smith, Stone, and Tutte, The dissection of rectangles into squares, Duke Math. J. 7 (1940) 312-340. \$\endgroup\$ Commented Oct 3, 2017 at 14:00
Put two in parallel, trim them resistors to 18R, then throw the rest in your parts bin for later.
https://hackaday.com/2017/04/10/hackaday-trims-its-own-resistors/
Or, assuming they're 10% resistors, find the ones that are on the low end (27R) and put two in series, putting that in parallel with one 27R. Put the last one in your parts bin for later.
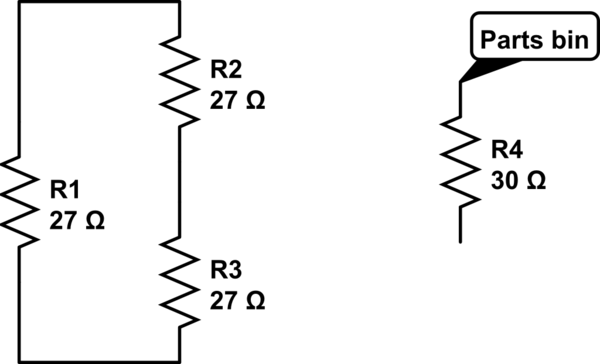
simulate this circuit – Schematic created using CircuitLab
-
5\$\begingroup\$ lol cant help thinking that is cheating \$\endgroup\$– Trevor_GCommented Oct 2, 2017 at 15:52
-
4\$\begingroup\$ I don't think it is a valid answer to this question. \$\endgroup\$ Commented Oct 2, 2017 at 15:53
-
5\$\begingroup\$ How do you intend to trim a 30 ohm resistor down in resistance to 18 ohms? \$\endgroup\$– MattCommented Oct 2, 2017 at 16:15
-
3\$\begingroup\$ @calcium3000 I did, my question stands. You are going the wrong way in resistance for the trimming they did. \$\endgroup\$– MattCommented Oct 2, 2017 at 16:30
-
1\$\begingroup\$ @Matt file it down, then put a thin coating of solder on it to decrease the resistance, then file the solder. Easy, right? \$\endgroup\$– GrantCommented Oct 2, 2017 at 19:33
-
\$\begingroup\$ LOL Same as mine but backwards.. I guess there are two solutions. \$\endgroup\$– Trevor_GCommented Oct 2, 2017 at 16:07
-
\$\begingroup\$ Cool. Didn't see this one... \$\endgroup\$ Commented Oct 2, 2017 at 16:07
-
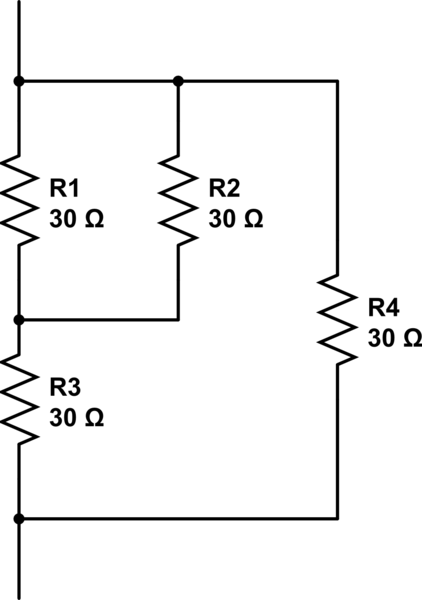
1\$\begingroup\$ @Trevor They're identical. Each of them can be described as a 30 ohm resistor in parallel with (a 30 ohm resistor in parallel with (two 30 ohm resistors in parallel)). The same instructions build both -- 1) Take two resistors in parallel. 2) Add a third in series. 3) Connect a fourth in parallel with the first three. \$\endgroup\$ Commented Oct 2, 2017 at 16:54
-
\$\begingroup\$ @DavidSchwartz effectively identical yes. Just laid out back asswards. \$\endgroup\$– Trevor_GCommented Oct 2, 2017 at 16:55
-
2\$\begingroup\$ @Trevor The schematics are laid out differently, but the schematic is just showing the solution. The solution is, per the question, a way of assembling the resistors. And both schematics depict precisely the same way of assembling the resistors. So they are not two solutions. \$\endgroup\$ Commented Oct 2, 2017 at 16:58
take the four 30 ohms resistors back the shop and ask for an 18 ohm resistor
- you might get some money back
- it is quicker to wire up
- is will cost less to produce
- it looks neater
- it will be more reliable
Invert the problem
Instead of seeking a resistor of 18 ohms, seek a conductor whose conductance is 1/18 siemens.
You have four conductors of 1/30 siemens.
When you parallel conductors, their conductance simply adds.
Placing conductors in series requires some fairly tricky math**, so invert them back into resistors for that math, because series resistances simply add.
** 1 / (1/C1 + 1/C2) ... Look familiar?